What is Optimality Theory? It’s a linguistic framework that, while lauded for its elegance and power, has faced significant criticism regarding its empirical grounding and inherent complexities. The theory posits that linguistic structures emerge from a competition between universal constraints, with the “optimal” form being the one that violates the fewest highly-ranked constraints. This seemingly straightforward approach, however, often leads to debates about constraint ranking and the inherent subjectivity in selecting and weighting these constraints.
The consequences of this inherent subjectivity are far-reaching, influencing not only the specific analyses produced but also the broader acceptance and applicability of the theory within the field of linguistics.
Optimality Theory (OT) emerged as a response to limitations perceived in earlier generative linguistic models. Its core components—the Generator (GEN), the Constraints (CON), and the Evaluator (EVAL)—represent a departure from rule-based systems. GEN generates a range of possible linguistic outputs, CON evaluates these outputs based on a ranked hierarchy of constraints, and EVAL selects the output that minimizes constraint violations.
However, the practical application of OT has proven challenging. The selection of constraints, their ranking, and the interpretation of results often involve subjective judgments, raising concerns about the theory’s scientific rigor and falsifiability. Furthermore, the computational complexity of evaluating numerous candidate outputs can be daunting, especially in complex linguistic phenomena. Despite these criticisms, OT continues to influence linguistic research, offering a powerful, albeit controversial, framework for analyzing linguistic structures.
Introduction to Optimality Theory

Optimality Theory (OT) is a constraint-based framework that has significantly impacted various fields, particularly linguistics. It posits that linguistic outputs are not derived through a series of transformational rules, but rather selected from a set of possible candidates based on the violation of ranked constraints. This approach offers a powerful and elegant method for analyzing linguistic patterns across different levels of language.
Core Principles of Optimality Theory
OT operates on three fundamental components: GEN, CON, and EVAL. GEN (generator) generates a potentially infinite set of candidate outputs for a given input. CON (constraints) represents universal linguistic principles, often expressed as markedness or faithfulness constraints. Markedness constraints penalize outputs with undesirable properties (e.g., complex consonant clusters), while faithfulness constraints penalize outputs that differ significantly from the input (e.g., changes in segments).
EVAL (evaluator) ranks the constraints and selects the optimal candidate, the one that violates the least highly-ranked constraints. This selection process is based on
harmonic alignment*, where the candidate with the fewest violations of the highest-ranked constraints is preferred, even if it violates lower-ranked constraints more often.
For example, consider the English plural rule. The input /kat/ (“cat”) generates candidates like /kat/, /kats/, /katz/. Faithfulness constraints favor /kats/ (preserving the input), while markedness constraints penalize the complex cluster /ts/ in /kats/. If the markedness constraint against complex clusters is ranked higher, /katz/ is selected as the optimal output. The ranking of constraints determines the outcome; a different ranking could yield a different output.
This illustrates the core mechanism of constraint interaction and ranking central to OT.
Historical Overview of Optimality Theory
OT emerged in the early 1990s, primarily through the work of Alan Prince and Paul Smolensky. Their seminal work,
Optimality Theory
Constraint Interaction in Generative Grammar* (1993), laid the groundwork for the theory. Early applications focused heavily on phonology, but OT rapidly expanded into syntax, morphology, and other areas. Key milestones include the development of specific constraint types (e.g., markedness, faithfulness, alignment) and the refinement of constraint ranking methods. Significant debates have revolved around the universality of constraints, the nature of constraint interaction, and the role of learning in constraint ranking.
While initially met with some skepticism, OT has become a major force in linguistic theory, influencing other frameworks and inspiring significant research.
Examples of Fields Where Optimality Theory is Applied
OT’s applicability extends beyond linguistics. The following table provides examples:
| Field | Application Example | Problem Addressed |
|---|---|---|
| Phonology | Analysis of vowel harmony in Turkish, where vowels in a word assimilate to a specific feature (e.g., backness) of a dominant vowel. | Explaining the consistent patterns of vowel changes across words. |
| Syntax | Modeling word order variation in languages with flexible word order, explaining the preferences for certain word orders over others. | Accounting for the range of possible word orders and their relative frequencies. |
| Morphology | Analyzing the selection of allomorphs in inflectional morphology, explaining why certain forms are chosen over others in different contexts. | Predicting the correct form based on morphological and phonological context. |
| Second Language Acquisition | Modeling the acquisition of phonological or grammatical patterns in a second language, explaining why learners make certain errors and how they eventually converge on native-like forms. | Understanding the stages of acquisition and the role of constraints in shaping learner grammars. |
| Music Theory | Analyzing musical structures and explaining preferences for certain melodic or harmonic progressions. | Identifying underlying principles that govern musical perception and composition. |
Comparative Analysis of Optimality Theory
Comparing OT with Government and Binding (GB) theory reveals fundamental differences. GB relies on transformational rules to derive surface structures from deep structures, while OT selects the optimal output from a set of candidates based on constraint ranking.
| Feature | Optimality Theory | Government and Binding |
|---|---|---|
| Core Assumption | Constraint-based selection of optimal output | Transformational rules mapping deep structure to surface structure |
| Methodology | Constraint ranking and tableau evaluation | Derivation through transformations and principles |
| Strengths | Handles variation elegantly, explains patterns without stipulating rules for each case. | Provides a detailed account of syntactic relations and structures. |
| Weaknesses | Constraint ranking can be complex and difficult to determine empirically. | Can be overly complex and difficult to learn; struggles with variation. |
Constraints in Optimality Theory
Optimality Theory (OT) posits that grammar is a system of ranked constraints. These constraints represent universal principles of well-formedness, and their interaction, determined by their ranking, accounts for the variation and patterns observed across different languages. Understanding the nature and interaction of these constraints is crucial to understanding the core mechanisms of OT.Constraints in OT are violable; a given linguistic form might violate one or more constraints.
However, the grammar selects the output that violates the least highly ranked constraints. This process, known as constraint ranking, is central to OT’s power. The theory does not stipulate which constraints exist or how they should be ranked; rather, it provides a framework for investigating these questions empirically.
Types of Constraints
Constraints in OT are typically categorized into faithfulness constraints and markedness constraints. Faithfulness constraints penalize differences between the input (underlying representation) and the output (surface form). Markedness constraints penalize the output form itself, regardless of the input. The interaction of these two constraint types drives the grammatical system. For instance, a faithfulness constraint might favor preserving a consonant in the output that is present in the input, while a markedness constraint might penalize consonant clusters.
The relative ranking of these constraints determines which of these competing tendencies wins out in a particular language.
Constraint Ranking and its Significance
Constraint ranking is the heart of Optimality Theory. It determines which linguistic output is optimal given a specific input and a set of constraints. The ranking is language-specific and is not predetermined; instead, it is learned by language learners based on exposure to the language data. A higher-ranked constraint has precedence over a lower-ranked one. If a constraint is violated, but the violation is less serious (because the constraint is lower-ranked) than the violation of a higher-ranked constraint, the lower-ranked constraint violation is tolerated.
This hierarchical system of constraint interaction allows for a parsimonious account of linguistic variation. For example, a language might rank a constraint favoring syllable-final consonants higher than a constraint penalizing consonant clusters, leading to different outcomes compared to a language with the opposite ranking. The significance lies in its ability to explain variation and typology without resorting to language-specific rules.
Hypothetical Constraint Ranking System: Syllable Structure
Let’s consider the phenomenon of syllable structure, specifically focusing on the potential for consonant clusters at the onset of syllables. We can posit two constraints:* MAX-ONS: Every consonant in the input must be realized in the output (a faithfulness constraint).
*COMPLEX
Syllable onsets should not contain consonant clusters (a markedness constraint).We can then hypothesize two different constraint rankings, reflecting potential cross-linguistic variation: Language A (e.g., English):
COMPLEX >> MAX-ONS*
In Language A, the avoidance of complex onsets is prioritized. Therefore, an input like /str/ might be realized as /str/ (violatingCOMPLEX*) or potentially as /ster/ (violating MAX-ONS). The grammar chooses the least bad option, in this case potentially the latter if another constraint, such as one favoring vowel insertion, is also at play. Language B (e.g., a language with stricter onset restrictions):
- MAX-ONS >>
- COMPLEX*
In Language B, the preservation of consonants in the input is prioritized. Thus, the input /str/ would likely be realized as /str/, even if it violates
COMPLEX*, because violating MAX-ONS is considered more costly.
This hypothetical example demonstrates how different rankings of the same constraints can lead to different surface forms, providing a unified account of cross-linguistic variation in syllable structure. The specific ranking is not predicted by the theory but is instead determined empirically through analysis of language data.
Violation and Ranking
Optimality Theory (OT) hinges on the interplay between constraints and their ranking to determine the optimal output for a given linguistic input. The core mechanism involves evaluating candidate outputs based on the number and severity of constraint violations they incur. The output with the least serious violations, according to the predetermined constraint hierarchy, is selected as the optimal form.Constraint violation is the fundamental mechanism driving output selection in OT.
Each candidate output is evaluated against a predefined set of universal constraints, each representing a well-formedness principle of the language. A candidate that violates a constraint incurs a “violation mark,” indicating a departure from the ideal form dictated by that constraint. The severity of a violation can vary depending on the constraint’s nature; some constraints may be considered “harder” or more important than others.
The ranking of constraints determines which violations are considered more serious and thus have a greater impact on the selection of the optimal candidate. A higher-ranked constraint’s violation outweighs the violation of a lower-ranked constraint, even if the lower-ranked constraint is violated multiple times.
Constraint Ranking Methods
Several methods exist for determining the ranking of constraints within an OT system. One common approach involves employing a learning algorithm that iteratively refines the constraint ranking based on observed linguistic data. This data typically consists of input-output pairs, representing the linguistic forms that are attested in the language under investigation. The algorithm adjusts the constraint ranking to maximize the accuracy of the model in predicting the observed outputs.
Another method involves employing comparative analysis across multiple languages, identifying common constraints and exploring cross-linguistic variation in constraint ranking. This approach assumes that a universal set of constraints exists, but their ranking varies according to the specific linguistic properties of each language. A third approach, more theoretical in nature, proposes establishing constraint ranking based on linguistic principles and theoretical considerations, prioritizing constraints based on their perceived importance or power.
This approach is less data-driven and relies heavily on theoretical assumptions about language structure and acquisition.
Challenges in Determining Optimal Constraint Ranking
Determining the optimal constraint ranking presents several significant challenges. One major challenge is the potential for multiple equally optimal rankings. Given a finite set of constraints and a finite amount of data, it’s possible to have multiple rankings that perfectly account for the observed data. This ambiguity necessitates exploring alternative evaluation metrics and incorporating additional linguistic considerations to refine the ranking.
Another challenge arises from the inherent complexity of natural language. The interaction of multiple constraints can lead to intricate patterns of violation and output selection, making it difficult to establish a clear and unambiguous ranking. Furthermore, the available data may be incomplete or noisy, potentially leading to inaccurate or unstable rankings. Finally, the theoretical assumptions underlying constraint selection and ranking can influence the results, highlighting the need for rigorous testing and validation of different theoretical frameworks.
Generative Processes

Optimality Theory (OT) posits a generative process that accounts for the variation and regularity observed in linguistic data. Unlike rule-based approaches, OT doesn’t employ sequential rules to derive surface forms. Instead, it relies on a parallel evaluation of candidate outputs generated from an underlying representation, with the optimal candidate selected based on a ranked constraint hierarchy. The process is fundamentally competitive, with each candidate vying for selection based on its faithfulness to the input and its adherence to markedness constraints.The generative process in OT begins with a single underlying representation, often referred to as the input.
This input undergoes a process of generation, producing a range of possible output candidates. These candidates are then evaluated simultaneously against a ranked hierarchy of constraints. The constraint ranking determines which candidate is deemed optimal, and this optimal candidate is the surface form predicted by the theory. Crucially, the interaction between the generative process and constraint ranking is what drives the selection of the optimal form.
A highly ranked constraint will exert a stronger influence on the selection process, potentially overriding violations of lower-ranked constraints.
Candidate Generation and Constraint Evaluation
The generation of candidate outputs is not fully specified within OT; it is generally assumed that the generator is capable of producing all logically possible outputs given the input. This allows for the exploration of a wide range of linguistic possibilities. The constraints, then, act as filters, selecting the best candidate from this pool. Each candidate is evaluated against every constraint in the hierarchy.
A violation of a constraint is typically marked with an asterisk (*). The candidate with the least serious violations according to the ranking emerges as the optimal form.
Illustrative Example: Alveolar Stop Deletion in English
Let’s consider a simplified example of alveolar stop deletion in English, where the alveolar stop /t/ may be deleted in word-final position, as in the pronunciation of “street” as [stri:]. We will focus on three constraints:
- MAX-C* (maximize consonants),
- IDENT-IO* (identity between input and output consonants), and
DEP-IO* (dependency between input and output consonants). The ranking we will use is
MAX-C >> IDENT-IO >> DEP-IO. This means that MAX-C is the highest-ranked constraint, followed by IDENT-IO, and then DEP-IO.
| Input | Candidate Output | MAX-C Violations | IDENT-IO Violations |
|---|---|---|---|
| /stri:t/ | [stri:t] | 0 | 0 |
| /stri:t/ | [stri:] | 1 | 0 |
In this example, the input is /stri:t/. The generator produces two candidates: [stri:t] (the faithful pronunciation) and [stri:] (the pronunciation with alveolar stop deletion). The table shows the constraint violations for each candidate. [stri:t] satisfies all constraints, while [stri:] violates MAX-C (it deletes a consonant). However, because MAX-C is outranked by IDENT-IO and DEP-IO, which are satisfied by [stri:], the candidate [stri:] is not selected as the optimal candidate.
In a different ranking, where MAX-C was the lowest ranked constraint, the candidate [stri:] would be selected as optimal. This illustrates how constraint ranking determines the outcome of the generative process.
Evaluation of Candidates
Optimality Theory (OT) evaluates linguistic outputs by comparing a set of candidate forms generated from a given input. This evaluation process is crucial because it determines which candidate best satisfies a ranked hierarchy of linguistic constraints. The candidate that violates the fewest high-ranked constraints emerges as the optimal output.The evaluation process involves a crucial step of constraint violation comparison.
Each candidate is assessed against each constraint in the system. Constraints are ranked hierarchically, meaning that a violation of a higher-ranked constraint outweighs multiple violations of lower-ranked constraints. This hierarchical ranking allows for the resolution of conflicting constraints, as the system prioritizes the satisfaction of higher-ranked constraints even if it means violating lower-ranked ones. The candidate with the least serious violations, as determined by constraint ranking, is selected as the optimal output.
This selection process is not simply a count of violations; the hierarchy of constraints is paramount.
Constraint Violation and Ranking
Consider a simplified example focusing on the constraint ranking and violation process. Let’s assume we have two constraints:Onset* (all syllables must begin with a consonant) and
NoCoda* (no syllables can end with a consonant). We will evaluate two candidate outputs for the input /CV/
Candidate 1: CVC and Candidate 2: CV. The table below illustrates the evaluation:
| Candidate | Onset | NoCoda | Evaluation |
|---|---|---|---|
| CVC | * | ! | * |
| CV | * | * | * |
Here, ‘*’ indicates constraint satisfaction and ‘!’ indicates a violation. Assuming
- Onset* outranks
- NoCoda*, Candidate 1 violates a lower-ranked constraint (*NoCoda*) while satisfying the higher-ranked constraint (*Onset*). Candidate 2 satisfies both constraints. If
- Onset* outranks
- NoCoda*, then Candidate 2 is the optimal output because it satisfies the higher-ranked constraint. However, if
- NoCoda* outranks
- Onset*, then Candidate 1 would be the optimal output. This demonstrates how the ranking of constraints determines the outcome of the evaluation.
Illustrative Example with Multiple Constraints
To further illustrate the evaluation process with multiple constraints, let’s consider a slightly more complex scenario. We’ll analyze three candidates for the input /bæks/:
| Candidate | Max (maximise syllables) | *Onset (all syllables must begin with a consonant) | NoCoda (no syllables can end with a consonant) | Ident(input=output) | Evaluation |
|---|---|---|---|---|---|
| bæks | * | * | ! | * | * |
| bæk.s | ! | * | * | ! | ! |
| bæs.k | ! | ! | * | ! | ! |
Let’s assume the constraint ranking is: Max >>
- Onset >> NoCoda >> Ident. The symbol “>>” represents that the constraint on the left is ranked higher than the constraint on the right. Candidate 1, “bæks,” violates only
- NoCoda*, a relatively low-ranked constraint. Candidates 2 and 3 violate higher-ranked constraints. Therefore, even though “bæks” violates a constraint, it is still the optimal candidate because the violated constraint is lower ranked than those violated by the other candidates. This exemplifies how OT handles multiple conflicting constraints through hierarchical ranking.
Applications in Phonology

Optimality Theory (OT) has significantly impacted phonological analysis, offering a powerful framework for understanding the complex interplay of constraints that shape sound systems. Its constraint-based approach provides a flexible and insightful methodology for modeling phonological processes across diverse languages. This section explores various applications of OT in phonology, examining its strengths and limitations in comparison to other theoretical approaches.
Optimality Theory and Phonological Analysis
The following examples illustrate OT’s application in analyzing phonological processes. Each example demonstrates how a specific constraint ranking accounts for observed patterns.
| Example | Language/Family | Phonological Process | Constraint Ranking (highest to lowest) |
|---|---|---|---|
| Example 1 | English | Tense vowel shortening in unstressed syllables (e.g., “parade” vs. “parade’s”) | *STRESSED-TENSE > MAX-V > DEP |
| Example 2 | Japanese | Mora-timed syllable structure | *COMPLEX-ONSET > ONSET > NO-CODA |
| Example 3 | Korean | Tense/lax vowel neutralization in certain environments | IDENT-VFEATURE > MAX-V > – TENSE-V |
Note:
- STRESSED-TENSE penalizes tense vowels in unstressed syllables; MAX-V penalizes vowel deletion; DEP penalizes consonant deletion;
- COMPLEX-ONSET penalizes complex onsets; ONSET prefers onsets; NO-CODA prefers codas; IDENT-VFEATURE prefers identical vowel features;
- TENSE-V penalizes tense vowels. These are simplified representations and may require further refinement depending on the specific analysis.
Explaining Specific Phonological Patterns with Optimality Theory
(a) Vowel Harmony in Turkish: Turkish vowel harmony involves the agreement of backness (front vs. back) and roundness (rounded vs. unrounded) between vowels in a word. OT explains this through constraints like
- BACK-FRONT,
- ROUND-UNROUND, and IDENT(BACK), IDENT(ROUND). These constraints interact to ensure harmony. A high ranking of IDENT(BACK) and IDENT(ROUND) will force the vowels to agree in backness and roundness, while violations of these constraints are tolerated if other higher-ranked constraints are satisfied. For example, if a suffix contains a front vowel, the stem vowel will also be fronted to satisfy IDENT(BACK), even if this results in a less preferred vowel.
(b) Syllable Structure Constraints in Japanese: Japanese syllable structure is largely (CV) or (V). OT accounts for this through constraints like
Optimality Theory? Think of it as finding the best solution, like choosing between a million ice cream flavors. But sometimes, the best solution needs a little…brewing time, which is where understanding what is the incubation theory space comes in handy. Essentially, Optimality Theory’s all about efficiency – even if that efficiency needs a mental coffee break first!
- COMPLEX-ONSET, which penalizes consonant clusters in onset position, and
- CODA, which penalizes consonants in coda position. The high ranking of these constraints enforces the (CV) or (V) syllable structure. Exceptions, where consonant clusters or codas appear, are handled by lower-ranked constraints or through the interaction of multiple constraints.
(c) Consonant Assimilation in English: Consonant assimilation, such as the voicing assimilation in “dogs” ( /dɔgz/), is explained by constraints like IDENT(VOICE) and MAX. IDENT(VOICE) prefers identical voicing features between adjacent consonants. MAX penalizes consonant deletion. The high ranking of IDENT(VOICE) forces voicing assimilation, resulting in the voiced /ɡ/ in “dogs”. However, the constraint MAX prevents complete assimilation in cases where it would lead to consonant deletion.
Advantages and Limitations of Optimality Theory in Phonology
| Feature | Optimality Theory | Alternative Framework (Rule-Based Phonology) |
|---|---|---|
| Power | Provides a unified framework for explaining diverse phonological phenomena through constraint interaction. | Relies on ordered rules, which can become complex and difficult to manage in languages with numerous exceptions. |
| Predictive Power | Can predict phonological patterns based on constraint ranking, though this prediction is dependent on the accuracy of the proposed constraint set and ranking. | Predictive power is limited as it relies on specifying rules for each pattern. |
| Simplicity/Elegance | Aims for simplicity by reducing phonological descriptions to a set of universal constraints and language-specific rankings. | Can become cumbersome with a large number of rules and exception specifications. |
| Handling Exceptions | Handles exceptions by allowing violations of lower-ranked constraints. | Often requires additional rules or exception specifications to account for exceptions, leading to complexity. |
| Empirical Coverage | Demonstrates broad empirical coverage across many languages and phonological processes. | Can struggle to account for some phonological patterns elegantly, particularly those with numerous exceptions. |
Case Study: Phonotactics of Hawaiian
Hawaiian phonology exhibits a relatively simple syllable structure, primarily consisting of CV syllables. The language features a limited consonant inventory and a relatively small set of vowels. Several constraints within the Optimality Theory framework account for these patterns. The constraint
- COMPLEX-ONSET severely restricts consonant clusters, forcing the language towards a preference for simple onsets. Similarly, the constraint
- CODA penalizes syllable-final consonants, leading to the prevalence of open syllables. The constraint MAX, which penalizes the deletion of segments, ensures that all intended segments are present in the pronunciation. The interaction of these constraints shapes the phonotactic inventory and syllable structure. For instance, the absence of consonant clusters in Hawaiian is a direct result of the high ranking of
- COMPLEX-ONSET. The constraint ranking, therefore, successfully predicts and explains the observed patterns in Hawaiian phonotactics. Further, the relatively small consonant and vowel inventories can be accounted for by constraints prioritizing simple segments, which may include constraints penalizing certain articulatory features or positions.
Applications in Morphology
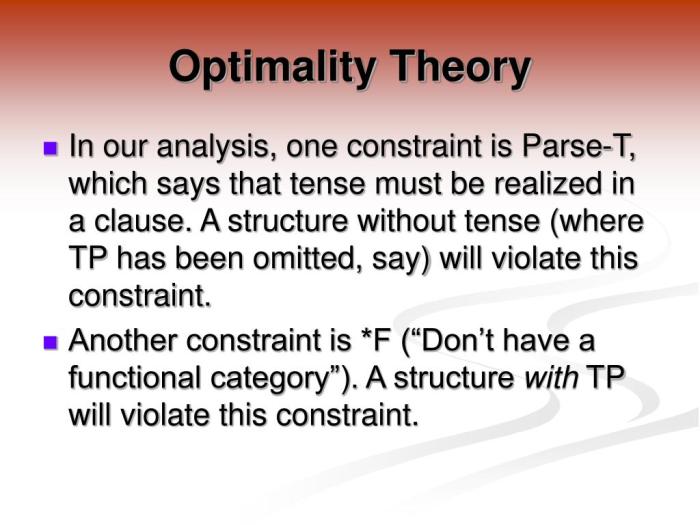
Optimality Theory (OT), initially developed for phonology, has proven remarkably adaptable to morphological analysis. Its framework of competing constraints and ranked optimization provides a powerful tool for understanding the complexities of word formation, accounting for allomorphy, reduplication, and morphological productivity in ways that previous models struggled to achieve. This section explores OT’s application in morphology, examining specific examples across different language families and highlighting its strengths and limitations.
Examples of Optimality Theory in Morphological Analysis
The application of Optimality Theory to morphological processes offers a nuanced approach to understanding the complexities of word formation across diverse languages. By analyzing the interaction of competing constraints, OT provides insights into the selection of optimal morphological forms. The following examples illustrate this approach across different language families.
- Niger-Congo Language (e.g., Yoruba): Consider Yoruba nominal pluralization. The underlying morpheme for plural might be /-o/, but it surfaces as /-o/ after vowels and as /-wɔ/ after consonants. Constraints such as
-Vowel-Vowel (penalizing vowel sequences) and
-Consonant-Vowel (penalizing consonant-vowel sequences) interact with MAX (a faithfulness constraint requiring the presence of the underlying plural morpheme) and DEP (a faithfulness constraint requiring the absence of extra segments).The ranking
-Vowel-Vowel >>
-Consonant-Vowel >> MAX >> DEP would lead to /-o/ after vowels and /-wɔ/ after consonants, maximizing faithfulness while minimizing markedness violations. The optimal output reflects the interaction between faithfulness to the underlying form and avoidance of phonotactically undesirable sequences. - Indo-European Language (e.g., English): English past tense formation presents an interesting case. The regular past tense morpheme /-d/ can surface as /-t/, /-d/, or /-ɪd/ depending on the phonological context of the verb stem. Constraints like
-[-t] (avoiding a [-t] suffix after a voiceless obstruent),
-[-d] (avoiding a [-d] suffix after a voiced obstruent), and
-[-ɪd] (avoiding a [-ɪd] suffix in other contexts) interact with MAX and DEP.A likely constraint ranking would prioritize markedness constraints (*[-t],
-[-d],
-[-ɪd]) to varying degrees, followed by MAX and then DEP, leading to the appropriate allomorph selection depending on the phonological environment. - Austronesian Language (e.g., Tagalog): Tagalog employs reduplication for pluralization and other grammatical functions. Consider the reduplication of the word
-sulat* (“write”). Constraints such as IDENT(Root, Reduplicant) (requiring identity between the root and the reduplicant), MAX(Root), and MAX(Reduplicant) (requiring the presence of all segments in the root and the reduplicant) interact. A high ranking of IDENT(Root, Reduplicant) would lead to a perfect reduplication (*sulat-sulat*), while a lower ranking would allow for partial reduplication or other variations depending on the specific phonological context and other interacting constraints.
Allomorphy in Optimality Theory
Allomorphy, the phenomenon where a single morpheme has multiple surface forms, is elegantly handled within the OT framework. Consider the English plural morpheme, which surfaces as /-s/, /-z/, or /-əz/. The underlying morpheme is /-z/. Constraints like
- [+voice]-[-voice] (avoiding a voiced consonant followed by a voiceless consonant),
- Sibilant-Sibilant (avoiding sequences of sibilants), and
- Sibilant-Sibilant >>
- Complex-Onset >>
- [+voice]-[-voice] >> MAX >> DEP. This ranking predicts /-s/ after voiceless consonants, /-z/ after voiced consonants, and /-əz/ after sibilants, reflecting the observed allomorphy.
Complex-Onset (avoiding complex syllable onsets) interact with MAX and DEP. The optimal allomorph is selected based on the constraint ranking which prioritizes the avoidance of phonotactically marked sequences while maintaining faithfulness to the underlying morpheme. A possible ranking would be
Reduplication in Optimality Theory
Reduplication, a morphological process involving the repetition of all or part of a word, is another area where OT shines. Let’s analyze reduplication in Indonesian, where reduplication is used to form intensive verbs. Consider the verb
- baca* (“read”). The intensive form is
- baca-baca*. Constraints like IDENT(Root, Reduplicant),
COMPLEX (penalizing complex structures), and MAX and DEP, are relevant. A possible tableau illustrating the selection of the optimal reduplicated form is shown below
| Candidate | IDENT(Root, Reduplicant) | *COMPLEX | MAX | DEP |
|---|---|---|---|---|
| baca-baca | 0 | 0 | 0 | 0 |
| baca-ba | 1 | 0 | 1 | 0 |
| baca-ca | * | 0 | * | 0 |
| baca | * | 0 | * | * |
In this scenario, IDENT(Root, Reduplicant) is highly ranked, leading to the selection of the full reduplicationbaca-baca* as the optimal form. Violations are marked with an asterisk (*).
Explaining Optimality Theory’s Approach to Morphological Processes
Optimality Theory offers a powerful framework for understanding various aspects of morphological processes.
Morphological Productivity
OT accounts for morphological productivity by considering the interaction between markedness and faithfulness constraints. Highly productive morphological processes tend to involve a relatively low ranking of markedness constraints, allowing for the creation of novel forms. Conversely, less productive processes might involve higher-ranked markedness constraints, restricting the creation of new forms. For example, the relatively high productivity of English -ed past tense formation reflects a lower ranking of markedness constraints compared to, say, the less productive conversion process (e.g.,
noun to verb*).
Interaction between Phonological and Morphological Processes
OT elegantly handles the interaction between phonological and morphological processes. Phonological rules can influence morphological well-formedness, and vice-versa. For example, the English plural allomorphy discussed earlier shows how phonological constraints (*Sibilant-Sibilant*,Complex-Onset*) directly influence the choice of the morphological allomorph. Similarly, morphological constraints can influence phonological processes; a constraint requiring a specific syllable structure might lead to phonological adjustments to ensure morphological well-formedness.
Role of Markedness Constraints
Markedness constraints in OT morphology penalize the use of less common or more complex morphological structures. These constraints interact with faithfulness constraints, which aim to preserve the underlying morphological form. The relative ranking of markedness and faithfulness constraints determines the optimal output. For instance, a constraint penalizing consonant clusters might lead to a simplification of a complex morpheme, reflecting a trade-off between faithfulness and markedness.
Comparing and Contrasting Optimality Theory in Phonology and Morphology
While OT’s core principles apply to both phonology and morphology, there are important differences in the types of constraints and their interactions.
Constraint Types
Phonological constraints often focus on syllable structure, segmental features, and phonotactics, while morphological constraints deal with morpheme boundaries, affixation, reduplication, and other word-formation processes. For example,
- NoCoda* is a phonological constraint, while
- Max-Root* is a morphological constraint.
Interaction of Linguistic Levels
In both phonology and morphology, OT handles interactions between different linguistic levels. In phonology, this involves the interplay between segmental and suprasegmental phonology. In morphology, it involves the interaction between morphology and phonology, as seen in the examples above. However, the specific nature of these interactions differs. In phonology, the interaction is often within a single word; in morphology, it might involve interactions across words or phrases.
| Aspect | Phonology | Morphology |
|---|---|---|
| Constraint Types | Syllable structure, segmental features, phonotactics | Morpheme boundaries, affixation, reduplication |
| Violation Evaluation | Based on phonetic/phonological properties | Based on morphological well-formedness and relationships between morphemes |
| Optimal Output | Phonetically/phonologically well-formed surface form | Morphologically well-formed surface form |
| Focus | Sound patterns | Word formation processes |
| Interaction with other levels | Primarily with phonetics and syntax | Primarily with phonology and syntax |
Applications in Syntax
Optimality Theory (OT), initially developed for phonology, has been extended to syntactic analysis, offering a framework for understanding the interaction of grammatical constraints and the selection of optimal syntactic structures. Unlike traditional generative grammar which relies heavily on transformational rules, OT proposes that syntactic structures emerge from the competition between ranked constraints, with the winning structure being the one that violates the fewest highly-ranked constraints.The application of OT to syntax involves positing a set of universal constraints that govern syntactic well-formedness.
These constraints can be violated, but the ranking of these constraints determines which violations are tolerated and which lead to ungrammaticality. Syntactic phenomena are explained by identifying the relevant constraints and their ranking in a particular language. The grammaticality of a given sentence is determined by the interaction of these ranked constraints, leading to the selection of the optimal syntactic structure.
Constraint Interaction and Word Order Variation
OT provides a compelling account of word order variation across languages. Consider the basic word order Subject-Verb-Object (SVO) versus Subject-Object-Verb (SOV). OT can model this variation by positing constraints such as
- HEAD-FINAL* (heads should appear at the end of phrases) and
- HEAD-INITIAL* (heads should appear at the beginning of phrases). In an SOV language,
- HEAD-FINAL* would outrank
- HEAD-INITIAL*, leading to the surface word order reflecting the final placement of heads. Conversely, in an SVO language,
- HEAD-INITIAL* would dominate
- HEAD-FINAL*. The specific ranking of these constraints, along with others such as constraints on subject position and object position, determines the preferred word order for a given language. For example, a language with a high ranking of
- HEAD-FINAL* and a lower ranking of
- SUBJECT-FIRST* might exhibit SOV word order, even if it has other constraints favoring other orders.
Constraint Ranking and Scrambling
Scrambling, the phenomenon where constituents in a sentence can appear in various orders without changing the basic meaning, is another area where OT has shown promise. Scrambling can be modeled by proposing constraints that favor certain constituent orders (e.g., a constraint favoring subjects preceding objects) and constraints that penalize deviations from a basic word order. The ranking of these constraints determines the permissible degree of scrambling in a given language.
A language with a highly ranked constraint against deviations from a basic word order will allow little or no scrambling, while a language with a lower ranking of this constraint will allow a greater degree of flexibility in constituent order. The resulting surface word orders are not derived through transformations but rather emerge as optimal candidates given the ranked constraint set.
Challenges and Successes of Optimality Theory in Syntax
While OT offers a powerful and elegant framework for analyzing syntactic phenomena, its application faces certain challenges. The identification of the relevant universal constraints and the determination of their ranking for a given language can be complex and require careful consideration of a wide range of data. Furthermore, the computational complexity of evaluating all possible candidate structures can be significant, particularly for complex sentences.Despite these challenges, OT has achieved considerable success in explaining various syntactic phenomena, including word order variation, scrambling, and the interaction of different grammatical processes.
Its emphasis on constraint interaction and the competition among candidate structures provides a more nuanced and empirically grounded approach to syntactic analysis than traditional rule-based systems. The ability to account for variation across languages through the ranking of universal constraints is a particularly appealing aspect of the OT approach. Further research is needed to refine the set of universal constraints and to develop more efficient computational methods for evaluating candidate structures, but the potential of OT in syntax remains considerable.
Formalization of Optimality Theory
Optimality Theory (OT), while intuitively appealing in its conceptual framework, gains significant rigor and predictive power through formalization. This allows for precise representation of constraint interactions, candidate evaluation, and the derivation of optimal forms. Mathematical formalization provides a framework for unambiguous analysis and facilitates computational modeling, enhancing the theory’s scope and applicability across diverse linguistic phenomena.
Mathematical Representation
The core of OT’s mathematical representation lies in set theory and the concept of partial orderings. A candidate set, denoted as
- C*, represents all possible linguistic outputs for a given input. Each candidate is a string or structure conforming to the underlying grammatical system. The constraint set, denoted as
- CON*, comprises a collection of ranked constraints, each evaluating a specific aspect of grammatical well-formedness. Each constraint,
- c 1 ≫ c 2* signifies that constraint
- c 1* outranks
- c 2*. Total ordering implies a linear ranking where every constraint’s relative priority is explicitly defined; partial ordering allows for incomparability between some constraints, reflecting situations where the relative priority remains indeterminate.
c ∈ CON*, is a function mapping candidates to non-negative integers representing the number of violations
c
C → ℕ*. Constraint ranking is represented as a strict weak order, often denoted as ≫, indicating the relative priority of constraints. For instance,
Different ranking scenarios can be visualized. For example, a total ordering of three constraints (A, B, C) where A ≫ B ≫ C can be easily represented linearly. A partial ordering, however, might involve A ≫ B and C ≫ B, but leave the relative ranking of A and C unspecified. This could be visualized using a Hasse diagram, where constraints are represented as nodes and the ranking is represented by directed edges.
For instance, a Hasse diagram illustrating the partial order A ≫ B and C ≫ B would show A and C above B, with arrows pointing downwards from A to B and from C to B, but no arrow connecting A and C. The absence of a connecting arrow indicates the incomparability of A and C.Optimality is formally defined within this framework as the selection of the candidate with the minimal violation profile according to the ranked constraint set.
The optimal candidate,
- c* opt, is the candidate that is not dominated by any other candidate in
- C*. A candidate
- c i* dominates another candidate
- c j* if and only if for every constraint
- c ∈ CON*,
- c(c i) ≤ c(c j)*, and there exists at least one constraint
- c ∈ CON* such that
- c(c i) < c(cj)*. In simpler terms, the optimal candidate minimizes violations across all ranked constraints.
Role of Formalization in Rigorous Application
Formalization significantly enhances OT’s predictive power. By explicitly defining constraints and their rankings, it allows for precise predictions about the outcome of grammatical processes. For example, without formalization, describing vowel harmony might be vague. Formalizing it with constraints like[±back] agreement* and specifying their ranking enables precise predictions of which vowels will harmonize and how. Less formal approaches may lead to ad hoc explanations, while formalization allows for falsifiable hypotheses and more systematic testing.Formalization minimizes ambiguity and ensures consistency.
Different researchers using the same formal framework will arrive at similar conclusions when analyzing the same linguistic data. This contrasts with less formal approaches where interpretations can be subjective and lead to inconsistent analyses.Different formalization methods exist, each with advantages and disadvantages. While set-theoretic representations are common, others incorporate logical frameworks or algebraic structures. Set-theoretic approaches are relatively intuitive and easy to grasp, but may not capture the full complexity of certain linguistic phenomena.
More sophisticated formalisms offer greater expressiveness but may increase the complexity of analysis.Formalization facilitates computational modeling and simulation. Software can be developed to simulate the OT evaluation process, automatically generating optimal candidates based on specified constraints and rankings. This allows for large-scale simulations and the exploration of complex constraint interactions.
Demonstration of Formal Notation
Consider the phenomenon of consonant gemination in a simplified system. Let’s assume the input is /CV/ and the possible outputs are geminated [CVC] and non-geminated [CV]. We define two constraints:
- MAX*, which penalizes the deletion of consonants, and
- DEP*, which penalizes consonant clusters.
| Candidate | MAX | DEP | Ranking ||—|—|—|—|| [CVC] | 0 | 1 | 1 || [CV] | 1 | 0 | 2 |In this scenario, we assume
- MAX ≫ DEP*. [CVC] violates DEP once, while [CV] violates MAX once. Because
- MAX* outranks
- DEP*, the candidate with fewer violations of
- MAX* is selected as optimal, resulting in [CVC]. If the ranking were reversed (*DEP ≫ MAX*), [CV] would be optimal.
This simplified example highlights how constraint ranking directly determines the optimal output. Alternative rankings would lead to different predictions. The limitations of this formalization lie in its simplicity. Real-world consonant gemination is often influenced by other factors, such as phonotactics or morphological processes, which this simple model does not capture.
Variations and Extensions of Optimality Theory
Optimality Theory (OT), while influential, has undergone significant variations and extensions since its inception. These modifications address limitations, expand its applicability, and refine its theoretical underpinnings, leading to a diverse landscape of related frameworks. This section explores key variations, extensions, applications, and ongoing debates within the field.
Core Framework Variations
Several variations of the core OT framework exist, each modifying key principles and impacting application areas. The following table summarizes five prominent variations.
| Variation Name | Key Defining Principle(s) | Primary Application Area(s) | Strengths | Weaknesses |
|---|---|---|---|---|
| Classical Optimality Theory | Strict ranking of violable constraints; selection of the candidate with the least constraint violations. | Phonology, morphology, syntax | Elegant simplicity, wide applicability. | Difficulty handling gradience, overgeneration in some cases. |
| Harmonic Grammar (HG) | Constraints are weighted; candidate evaluation based on weighted harmony score. | Phonology, morphology | Handles gradience more naturally than classical OT. | Weight assignment can be complex and less transparent. |
| MaxEnt OT | Combines OT with maximum entropy modeling; constraint weights are learned from data. | Phonology, morphology | Learns constraint weights from data, better accounts for probabilistic phenomena. | Computationally more intensive; weight learning can be sensitive to data. |
| Stochastic OT | Introduces probability into constraint ranking and candidate selection. | Phonology, language acquisition | Models variation and gradience, relevant to language acquisition. | Increased complexity, challenges in parameter estimation. |
| Optimality-Theoretic Syntax (OTS) | Applies OT principles to syntactic analysis, focusing on constraint interaction in sentence structure. | Syntax | Provides a unified framework for syntactic analysis. | Can be complex to apply to complex syntactic phenomena; debate on constraint typology. |
Five Distinct Extensions to the Core Optimality Theory Framework
Several extensions broaden OT’s scope and address its limitations.
The following list describes five distinct extensions, each addressing specific limitations or expanding the applicability of the original framework.
- Weighted Constraints: This extension allows for different weights to be assigned to constraints, addressing the limitations of strictly ranked constraints in handling gradience and probabilistic phenomena. This is central to Harmonic Grammar.
- Variable Constraint Ranking: This allows for different rankings of constraints across different contexts or speakers, addressing the variability observed in language. This is crucial for modeling dialectal variation.
- Constraint Conjunction and Disjunction: This extension allows for the combination or separation of constraints, enabling a more nuanced representation of complex interactions. This enhances modeling of complex linguistic phenomena.
- Input-Output Correspondence Constraints: These constraints regulate the relationship between input and output forms, allowing for a better representation of processes involving faithfulness to underlying representations. This is particularly relevant for phonological processes.
- Lexical Entries and Constraint Interaction: This approach incorporates lexical information and allows for interactions between lexical entries and constraint rankings, enriching the model’s power, particularly useful for morphological analysis.
Comparison of Constraint Interaction in Harmonic Grammar and Classical OT
Classical OT employs a strict ranking of violable constraints, selecting the candidate with the fewest violations. Harmonic Grammar, conversely, uses weighted constraints, evaluating candidates based on a weighted harmony score. This difference significantly impacts the analysis of linguistic phenomena. For example, in classical OT, the interaction between a markedness constraint (*[voice]) and a faithfulness constraint (IDENT[voice]) leads to a binary outcome: either voicing is preserved or not.
In HG, the relative weights of these constraints could lead to a gradient outcome, where voicing is sometimes preserved and sometimes not, depending on the strength of the competing constraints. This better reflects the gradience often observed in natural language.
Application of Optimality Theory to Vowel Harmony in Turkic Languages
Turkic languages exhibit vowel harmony, where vowels in a word agree in features like backness and roundness. An OT analysis might posit constraints such as
- BACK-FRONT (prohibiting front and back vowels in the same word),
- ROUND-UNROUND (prohibiting round and unround vowels), and faithfulness constraints maintaining the input vowels. The ranking of these constraints determines the surface form. For example, if
- BACK-FRONT outranks faithfulness, a word with both front and back vowels might undergo a change to harmonize the vowels. Limitations might include difficulty in capturing exceptions or subtle variations in harmony patterns across different Turkic languages.
Three Areas Needing Further Development or Refinement in Optimality Theory
Further development is needed in several areas.
- Handling exceptions and markedness: OT struggles with exceptions to generalizations. Further research is needed on how to incorporate exceptions elegantly without compromising the framework’s parsimony.
- Computational tractability: The computational complexity of OT, particularly with large constraint sets, remains a challenge. Developing more efficient algorithms and computational tools is crucial.
- Integration with other linguistic frameworks: A better integration of OT with other linguistic frameworks, such as probabilistic models or connectionist approaches, would enhance its power and applicability.
Computational Modeling in Optimality Theory
Computational modeling plays a crucial role in testing and refining OT. Two approaches are commonly used:
- Constraint-based optimizers: These algorithms systematically search through candidate sets to find the optimal form based on constraint rankings. Software like “Optimality Theory Software” (OTS) exemplifies this approach.
- Statistical learning methods: These methods learn constraint weights from data, offering a data-driven approach to OT. Tools incorporating machine learning techniques are increasingly used.
Theoretical Debate: The Role of Markedness and Faithfulness
A central debate revolves around the relative importance of markedness (constraints penalizing undesirable structures) and faithfulness (constraints preserving input forms). Some argue that markedness constraints are primary, driving language change and shaping phonological systems. Others emphasize the role of faithfulness, highlighting the importance of preserving input information. This debate impacts how we interpret constraint interactions and the overall power of OT.
(See Prince & Smolensky, 1993; McCarthy, 2002 for contrasting perspectives).
Three Unresolved Issues or Controversies in Optimality Theory Research
- The nature of constraint ranking: Is constraint ranking universal or language-specific? Are rankings fixed or can they vary across contexts?
- The role of learning in OT: How do learners acquire constraint rankings? What is the role of innate knowledge versus experience?
- The relationship between OT and other linguistic theories: How can OT be integrated with other linguistic frameworks, such as HPSG or GB?
Three Promising Avenues for Future Research in Optimality Theory
- Developing more sophisticated learning models: This would allow for a better understanding of how constraint rankings are acquired and how they vary across individuals and languages.
- Integrating OT with probabilistic models: This would allow for a more nuanced representation of variation and gradience in language.
- Applying OT to new domains: This could include areas like language evolution, sign language, or computational linguistics.
Essay Artikel: Variations and Extensions of Optimality Theory
This essay will explore variations and extensions of Optimality Theory (OT), examining their strengths, weaknesses, and implications for linguistic analysis. I. Introduction:
Optimality Theory? Think of it as linguistic survival of the fittest – the best grammar wins! But will this principle apply to geopolitics? Check out this article on whether will south east asia start a domino theory to see if regional stability follows a similar “best outcome” model. Ultimately, understanding optimality theory helps us analyze complex systems, whether they’re sentences or nations.
- Brief overview of Optimality Theory and its core principles.
- Introduction of the key variations and extensions to be discussed.
- Thesis statement outlining the essay’s main argument.
II. Classical Optimality Theory and its Limitations:
- Detailed explanation of the core tenets of classical OT.
- Discussion of the limitations of classical OT, including its difficulty in handling gradience and probabilistic phenomena.
III. Harmonic Grammar:
- Explanation of the key principles of Harmonic Grammar (HG), including the use of weighted constraints.
- Comparison of HG with classical OT, highlighting its advantages and disadvantages.
- Examples of the application of HG to specific linguistic phenomena.
IV. MaxEnt OT:
- Description of MaxEnt OT and its integration of maximum entropy modeling with OT.
- Discussion of the strengths and weaknesses of MaxEnt OT, particularly its ability to learn constraint weights from data.
- Examples of the application of MaxEnt OT to phonological and morphological phenomena.
V. Conclusion:
- Summary of the main findings of the essay.
- Evaluation of the comparative strengths and weaknesses of the discussed variations and extensions.
- Suggestions for future research directions in the field of Optimality Theory.
Preliminary Bibliography:Prince, A., & Smolensky, P. (1993).
Optimality theory
Constraint interaction in generative grammar*. Rutgers Center for Cognitive Science.
- McCarthy, J. J. (2002).
- A thematic guide to Optimality Theory*. Cambridge University Press.
- Hayes, B. (2004).
- Legendre, G., Miyata, Y., & Smolensky, P. (1993). Harmonic Grammar: A formal framework for constraint-based grammar. In
- Proceedings of the 1993 CUNY Conference on Human Sentence Processing*.
- Goldsmith, J. (2005).
- Optimality theory in phonology*. Blackwell Publishing.
Phonological theory
The essentials*. Blackwell Publishing.
Strengths and Weaknesses of Optimality Theory

Optimality Theory (OT) has significantly impacted linguistic theory, offering a powerful framework for analyzing the interaction of constraints in shaping linguistic structures. However, like any theoretical framework, it presents both strengths and weaknesses that warrant careful consideration. This section will delve into a detailed examination of these aspects, providing concrete examples to illustrate the points discussed.
Formal Rigor of Optimality Theory
The formal rigor of OT stems from its reliance on precisely defined constraints and a transparent evaluation mechanism. Constraints, typically expressed as universally applicable principles, represent preferences for certain linguistic structures over others (e.g., a preference for simpler syllable structures or for maintaining faithfulness to the underlying form). The evaluation process involves comparing multiple candidate outputs generated by a grammar, each representing a potential realization of a linguistic input.
These candidates are evaluated against the ranked constraints; the candidate that incurs the least serious violations, according to the constraint ranking, emerges as the optimal output. For example, consider a constraint
- NoCoda* which penalizes syllables ending in consonants and a constraint
- Max-C* which penalizes the deletion of consonants. If
- NoCoda* outranks
- Max-C*, a word like /bakt/ might surface as [bak] (coda deletion) even though it violates
- Max-C*, because the violation of
- NoCoda* is deemed more serious. Conversely, if
- Max-C* outranks
- NoCoda*, the word would surface as [bakt], respecting the consonant in the coda. This formal system allows for precise predictions and testable hypotheses about linguistic behavior.
Power of Optimality Theory
OT has demonstrated significant power in various linguistic domains. In phonology, it elegantly accounts for phenomena like vowel harmony, consonant assimilation, and metathesis by showing how the interaction of faithfulness and markedness constraints shapes surface forms. For instance, in vowel harmony, a constraint requiring harmony between vowels in a word might interact with a constraint preferring a specific vowel.
The resulting surface form would reflect the ranking of these constraints. In syntax, OT has been applied to explain word order variation, explaining how different constraint rankings can lead to distinct word order patterns across languages. For example, a constraint preferring subject-verb-object (SVO) order might conflict with a constraint favoring verb-final order. The language’s specific word order will depend on the relative ranking of these constraints.
OT’s success lies in its ability to provide unified explanations for diverse phenomena based on a small set of universal constraints. Predictions made by OT models, such as the specific surface forms under certain constraint rankings, are often borne out by empirical data, enhancing its power.
Cross-linguistic Applicability of Optimality Theory
OT’s strength lies in its potential to account for cross-linguistic variation by adjusting the ranking of universal constraints. The same set of constraints can be applied to different languages, yielding diverse surface forms based on varying constraint rankings. For example, the constraints
- NoCoda* and
- Max-C* mentioned previously can be applied to languages with different syllable structures. In a language that allows codas,
- Max-C* will outrank
- NoCoda*, while in a language that disallows codas,
- NoCoda* will outrank
- Max-C*. This approach elegantly captures the diversity of phonological systems without resorting to language-specific rules. Similarly, in syntax, OT can explain cross-linguistic differences in word order by varying the ranking of constraints related to subject position, object position, and verb placement. The applicability of OT across diverse language families underscores its potential as a universal framework for linguistic analysis.
Interaction of Constraints in Optimality Theory
A core strength of OT lies in its explicit handling of constraint interaction. Multiple constraints often apply simultaneously to a single linguistic input, potentially leading to conflicting demands. OT resolves these conflicts through constraint ranking. The higher-ranked constraint takes precedence; violations of lower-ranked constraints are tolerated if necessary to satisfy higher-ranked ones. This mechanism allows OT to model complex linguistic phenomena arising from the interplay of multiple factors.
| Constraint Ranking | Output A (/bakt/) | Output B (/bakt/) | Output C (/bakt/) | Winning Candidate |
|---|---|---|---|---|
| *Faithfulness >> Markedness* | [bakt] (no violations) | [bak] (*NoCoda* violation) | [batk] (*Metathesis* violation) | [bakt] |
| *Markedness >> Faithfulness* | [bakt] (*NoCoda* violation) | [bak] (*NoCoda* violation, but less serious) | [batk] (*Metathesis* violation) | [bak] |
Challenges in Constraint Discovery in Optimality Theory, What is optimality theory
One significant weakness of OT is the challenge of identifying and formulating the relevant constraints for a given linguistic phenomenon. There is no algorithmic procedure for discovering constraints; researchers rely on linguistic intuition and observation to propose constraints. This process can be subjective, potentially leading to biases in constraint selection. Different researchers might propose different constraint sets for the same phenomenon, leading to conflicting analyses.
The lack of a clear methodology for constraint discovery raises questions about the objectivity and replicability of OT analyses.
Computational Complexity of Optimality Theory
Evaluating all possible candidate outputs under various constraint rankings can be computationally expensive, especially for complex linguistic structures. The number of candidate outputs grows exponentially with the complexity of the input, making exhaustive evaluation impractical for many realistic scenarios. This computational burden limits the applicability of OT to less complex linguistic phenomena and raises questions about its scalability.
Approximations and heuristics are often employed to mitigate this problem, but these can compromise the rigor of the analysis.
Limited Empirical Evidence for Optimality Theory
While OT has provided elegant explanations for many linguistic phenomena, empirical evidence supporting its predictions is not universally strong. In some cases, alternative analyses based on different theoretical frameworks provide equally compelling explanations. The lack of definitive empirical support for certain OT analyses weakens its overall credibility. For example, while OT can explain certain aspects of phonological processes, alternative rule-based approaches might also account for the same data.
This lack of strong empirical distinction between competing theories undermines OT’s claim to be a superior framework.
Challenges in Parameter Setting in Optimality Theory
Determining the optimal constraint ranking for a given language is a significant challenge. Multiple rankings might produce equally good fits to the data, leading to ambiguity in the analysis. This lack of unique solutions weakens the predictive power of OT and raises concerns about its falsifiability. Moreover, the criteria for selecting among equally valid rankings are often unclear, introducing subjectivity into the analysis.
This lack of clear criteria for ranking selection reduces the objectivity and replicability of OT analyses.
The Problem of Undergeneration and Overgeneration in Optimality Theory
OT faces criticism for both undergeneration and overgeneration. Undergeneration occurs when the theory fails to generate certain attested linguistic forms. This can be due to an incomplete or incorrectly ranked constraint set. Overgeneration occurs when the theory generates forms that are not attested. This might result from an overly permissive constraint set or an incorrect ranking.
For example, an OT analysis might fail to generate a specific word form due to an inadequate constraint, leading to undergeneration. Conversely, it might generate ungrammatical forms due to an overly permissive constraint, resulting in overgeneration. Proponents of OT argue that these problems can be addressed by refining the constraint set and their ranking, but the iterative nature of this process raises concerns about the theory’s stability and predictive power.
Criticisms Regarding the Role of Markedness in Optimality Theory
The notion of “markedness” in OT, which refers to the relative complexity or frequency of linguistic structures, has been subject to criticism. The definition and identification of markedness are often subjective and context-dependent. Different researchers might assign different markedness values to the same structure, leading to inconsistencies in OT analyses. Critics argue that the lack of a clear and objective measure of markedness weakens the foundation of OT and makes its predictions less reliable.
Counterarguments from OT proponents typically emphasize the importance of empirical data in determining markedness, arguing that the markedness scales are ultimately grounded in observed linguistic patterns.
Testability and Falsifiability of Optimality Theory
The testability and falsifiability of OT predictions are debated. While OT makes specific predictions about linguistic outputs based on constraint rankings, the flexibility in constraint selection and ranking can make it difficult to definitively falsify the theory. Critics argue that the lack of clear criteria for constraint selection and ranking makes it possible to adjust the model to accommodate almost any data, reducing its falsifiability.
Proponents of OT counter that while the theory is flexible, it is still subject to empirical testing. The success of OT in explaining diverse linguistic phenomena, along with the testable predictions derived from specific constraint rankings, strengthens its claim to be a scientifically valid theory. However, the ongoing debate about the criteria for evaluating OT analyses highlights the need for further research to strengthen its empirical foundation and refine its methodological rigor.
Comparison with Other Theories
Optimality Theory (OT) stands as a significant departure from many earlier generative linguistic frameworks. Its emphasis on constraint interaction and the evaluation of competing candidates offers a unique perspective on linguistic analysis, contrasting sharply with the rule-based approaches of earlier theories. Comparing OT with other prominent theories reveals both its strengths and limitations in accounting for the complexities of human language.Optimality Theory’s core mechanism, the interaction of violable, ranked constraints, differs fundamentally from the rule-based systems found in theories like Government and Binding (GB) or Lexical Functional Grammar (LFG).
GB, for instance, relies on a system of principles and parameters, along with transformational rules, to generate grammatical structures. These rules operate sequentially, applying in a predetermined order. LFG, on the other hand, uses a functional-based approach, mapping syntactic structures onto semantic representations via functional annotations. Both GB and LFG focus on the well-formedness of individual grammatical structures, while OT focuses on selecting the optimal candidate from a set of possible outputs.
Comparison of Optimality Theory and Government and Binding
Government and Binding theory, a prominent framework within generative linguistics, differs from Optimality Theory in several key aspects. GB utilizes a system of principles and parameters, along with transformational rules, to generate grammatical structures. These rules operate sequentially, applying in a predetermined order to derive surface forms from underlying representations. This contrasts with OT’s parallel evaluation of candidate outputs based on constraint violations.
For example, GB might account for subject-verb agreement through movement rules and features, while OT would achieve the same effect through the interaction of constraints like
- AGREEMENT* and
- PROXIMITY*. GB’s reliance on movement operations can lead to complexities in accounting for long-distance dependencies, while OT’s constraint-based approach potentially offers a more elegant solution in such cases, by focusing on the output’s overall harmony with the constraint ranking. However, GB’s detailed articulation of syntactic principles provides a deep understanding of structural relationships that might be less explicit in OT’s more surface-oriented approach.
Comparison of Optimality Theory and Lexical Functional Grammar
Lexical Functional Grammar, a functionalist approach to syntax, represents another significant contrast to Optimality Theory. LFG employs a functional architecture, mapping syntactic structures onto semantic representations via functional annotations. This focus on grammatical functions like subject, object, and predicate allows for a detailed analysis of the semantic roles of constituents. In contrast, OT’s focus is on the interaction of constraints in the selection of optimal outputs, without explicit mapping to functional annotations.
LFG might analyze passive constructions through a detailed description of the functional changes involved, mapping the underlying agent to a different grammatical function in the surface structure. OT, on the other hand, would analyze the passive by considering the interaction of constraints related to word order, thematic roles, and grammatical agreement. LFG’s strength lies in its detailed representation of grammatical functions and their semantic interpretations, whereas OT’s strength lies in its ability to handle a wider range of variation and exceptions through constraint ranking.
Comparative Table: Optimality Theory vs. Government and Binding
| Feature | Optimality Theory | Government and Binding |
|---|---|---|
| Underlying Mechanism | Constraint interaction and ranking; parallel evaluation | Principles and parameters; transformational rules; sequential application |
| Focus | Output selection; constraint violation minimization | Structure generation; movement operations; feature checking |
| Treatment of Variation | Handles variation through constraint reranking | Handles variation through parameter settings |
| Predictive Power | Predicts optimal outputs given a constraint ranking | Predicts grammatical structures based on principles and parameters |
| Empirical Coverage | Broad application across phonology, morphology, and syntax | Primarily focused on syntax |
Illustrative Example: Syllable Structure: What Is Optimality Theory
Optimality Theory (OT) provides a powerful framework for analyzing syllable structure across languages. Unlike rule-based approaches, OT explains syllable patterns through the interaction of ranked constraints, revealing the underlying principles governing phonological well-formedness. This section will demonstrate how OT accounts for syllable structure using a simplified example inspired by the syllable patterns found in languages like Japanese.This example focuses on the interaction of two primary constraints:
- Onset* and
- NoCoda*.
- Onset* requires every syllable to begin with a consonant, while
- NoCoda* prohibits syllables from ending with a consonant. The ranking of these constraints will determine the preferred syllable structure in the language. We will consider the underlying form /CV/, where C represents a consonant and V represents a vowel.
Constraint Interaction and Ranking
The language under consideration exhibits a preference for open syllables (CV). This preference is captured by the ranking of
- Onset* and
- NoCoda*. Specifically,
- NoCoda* >>
- Onset*. This ranking signifies that violations of
- NoCoda* are considered more harmful than violations of
- Onset*. Let’s analyze the possible surface forms for the underlying form /CV/ given this constraint ranking.
Tableau Representation of Syllable Structure
The following tableau illustrates the evaluation of candidate outputs for the underlying form /CV/. Each row represents a possible surface form, and the columns represent the constraints. A checkmark (✓) indicates that the constraint is satisfied, while an asterisk (*) indicates a violation. The optimal candidate is the one that minimizes constraint violations according to the constraint ranking.
| Candidate | NoCoda | Onset |
|---|---|---|
| CV | ✓ | ✓ |
| VC | * | ✓ |
| CVC | * | ✓ |
In this tableau, the candidate “CV” satisfies both constraints. The candidate “VC” violates
- NoCoda*, and the candidate “CVC” also violates
- NoCoda*. Since
- NoCoda* outranks
- Onset*, the candidate “CV” is the optimal output, reflecting the language’s preference for open syllables. The tableau demonstrates how the constraint ranking dictates the selection of the optimal form, showcasing the power of OT in explaining language-specific patterns. A different ranking, such as
- Onset* >>
- NoCoda*, would result in a different optimal output, potentially favoring closed syllables. This exemplifies the flexibility of OT in modeling diverse phonological systems.
Future Directions of Research
Optimality Theory (OT), despite its significant contributions to linguistic theory, remains a vibrant area of ongoing research. Several avenues promise to yield valuable insights into the nature of grammar and its acquisition, while also addressing existing limitations within the framework itself. Future research should focus on expanding the theory’s applicability to under-researched domains, refining existing methodologies, and developing robust computational models to simulate OT’s core mechanisms.
Identifying Promising Areas for Future Research within Optimality Theory
The continued development and refinement of Optimality Theory require exploration of new linguistic domains and methodologies. Three key areas, focusing on underspecified domains, cross-linguistic comparison, and computational modeling, hold significant promise for advancing our understanding of OT’s power and limitations.
Focus on Underspecified Domains
Three specific linguistic domains currently under-explored within the OT framework offer significant potential for theoretical advancement. These domains, characterized by complex interactions and a relative lack of existing OT analyses, are: (1) the syntax-semantics interface in languages with rich morphology, (2) the acquisition of prosodic structure in sign languages, and (3) the modeling of language variation and change through constraint demotion and promotion.
Investigating these areas can provide novel insights into the interplay of constraints across different linguistic levels and the dynamic nature of grammatical systems.
| Domain | Anticipated Theoretical Contribution | Potential Methodological Challenges |
|---|---|---|
| Syntax-Semantics Interface in Morphologically Rich Languages | Revealing how constraints on syntactic structure interact with constraints on semantic interpretation, particularly in languages with complex morphological systems where a single word may encode multiple grammatical functions. This could lead to a more unified theory of grammar, bridging the gap between syntax and semantics. | Difficulties in accurately representing complex morphological structures within the OT framework, and the challenge of formulating constraints that capture the intricate relationships between syntax and semantics. |
| Acquisition of Prosodic Structure in Sign Languages | Providing a deeper understanding of how constraints on handshape, location, and movement interact to shape the prosodic structure of sign languages. This could challenge existing assumptions about the universality of prosodic constraints and provide a richer understanding of the nature of prosody itself. | The need to develop new formalizations of constraints that are specifically tailored to the visual-motor modality of sign languages, and the difficulty of obtaining sufficient data on the acquisition of prosodic structure in sign languages. |
| Modeling Language Variation and Change through Constraint Demotion/Promotion | Developing formal models that simulate how changes in constraint rankings over time lead to language variation and change. This could provide a more dynamic and evolutionary perspective on grammar. | The difficulty in formulating computationally tractable models of constraint ranking change and the challenge of linking these models to empirical data on language change. |
Cross-Linguistic Comparison of Vowel Harmony
A comparative study of vowel harmony in Turkish (agglutinative), Hungarian (agglutinative with vowel harmony), and Swahili (Bantu with vowel harmony) will illuminate the cross-linguistic variation in constraint ranking and interaction. Vowel harmony, a process where vowels in a word assimilate to a specific vowel, presents an ideal case study due to its prevalence across diverse language families. This comparison will focus on the ranking of constraints related to feature spreading, faithfulness, and markedness, revealing similarities and differences in how these constraints interact to shape vowel harmony patterns in each language.
The analysis will consider the influence of factors like vowel inventory size and the presence of other phonological processes.
Computational Modeling of Constraint Interaction
A computational model employing a constraint satisfaction approach will simulate constraint interaction in OT. The model will focus on the resolution of conflicting constraints during the generation of optimal forms. The model will use a weighted constraint satisfaction system, where each constraint is assigned a weight reflecting its relative importance. The algorithm will iteratively adjust the weights based on the outcomes of candidate evaluation, aiming to converge on a weight distribution that accurately reflects the observed constraint rankings.
Expected outcomes include the generation of optimal forms consistent with empirical data and insights into the dynamic nature of constraint interaction. Limitations include the potential difficulty in finding a globally optimal weight distribution and the need for large datasets to train the model effectively.
Application to Sign Languages
Applying Optimality Theory to the analysis of phonological processes in American Sign Language (ASL) offers significant potential. Specifically, the analysis will focus on the interaction of handshape constraints and location constraints in the formation of compound signs. Existing OT frameworks may need adaptation to account for the unique spatial and temporal aspects of sign language phonology. For example, constraints on handshape might need to incorporate spatial information, while constraints on movement might need to incorporate temporal information.
Application to Language Acquisition
Optimality Theory can contribute to our understanding of the acquisition of phonotactic constraints. A specific testable hypothesis is that children initially acquire a less restrictive constraint ranking, allowing for a wider range of phonological forms. Over time, exposure to the input language leads to a refinement of the constraint ranking, resulting in the emergence of native-like phonotactic patterns.
This hypothesis can be tested by comparing the phonological output of children at different ages with the constraint rankings inferred from adult language data.
The Problem of Constraint Ranking
The arbitrariness of constraint rankings in Optimality Theory is a significant challenge. To address this, probabilistic methods can be incorporated. Instead of assigning fixed rankings, constraints would be assigned probabilities reflecting their relative importance. The optimal candidate would be the one maximizing the probability of satisfying all constraints. This probabilistic approach would allow for variation in constraint rankings across speakers and dialects, providing a more realistic model of linguistic variation.
The Interaction of Constraints
Constraint interaction in Optimality Theory can lead to emergent properties not predictable from the individual constraints. For example, the interaction of constraints on syllable structure and stress assignment can result in complex patterns of stress placement. Analyzing such interactions requires careful consideration of the interplay between different constraints and the development of methods for predicting emergent properties. This analysis will enhance our understanding of the complex interplay of constraints and the emergence of linguistic patterns.
Commonly Asked Questions
What are the main criticisms of Optimality Theory?
Criticisms include the subjectivity in constraint selection and ranking, computational complexity, difficulty in falsifying predictions, and the problem of undergeneration/overgeneration.
How does Optimality Theory compare to other linguistic theories?
Compared to rule-based approaches, OT offers a more constraint-based and parallel processing model. However, debates exist regarding its power and predictive accuracy compared to other frameworks like Lexical Functional Grammar or Head-driven Phrase Structure Grammar.
Can Optimality Theory be applied to areas outside of linguistics?
While primarily used in linguistics, the principles of constraint-based optimization have found applications in other fields, such as music theory and computer science, although their direct applicability and success vary.
What are some current research directions in Optimality Theory?
Current research focuses on refining the formalization of OT, developing more robust computational models, addressing issues of constraint learning, and expanding its application to under-researched linguistic domains, such as sign languages and language acquisition.

