Precalculus with limits answers? Yeah, we’re diving deep into the wild world of limits! Think of it like this: you’re trying to reach a destination, but you can only get infinitely closer – never quite arriving. Limits help us figure out where that “almost there” point actually is. We’ll crack the code on limit laws, one-sided limits, and even tackle those pesky indeterminate forms.
Get ready to level up your precalculus game, Pontianak style!
This guide breaks down the core concepts of limits in precalculus, providing clear explanations, worked examples, and even a few real-world analogies to make it all click. We’ll cover everything from basic limit laws to more advanced techniques for evaluating limits of different types of functions. We’ll also address common mistakes and offer strategies to avoid them, so you can confidently tackle any limit problem that comes your way.
Get ready to conquer limits!
Introduction to Limits in Precalculus
My dear students, embarking on the journey of limits is akin to scaling a majestic mountain. At first glance, the peak seems distant and unreachable, but with each step, each understanding, you will ascend closer to grasping its profound beauty. Limits, my friends, are the very foundation upon which the edifice of calculus is built. Let us begin our ascent together.
Limits Explained Through Real-World Analogies
Understanding limits requires moving beyond abstract mathematical concepts and connecting them to the tangible world around us. Consider these analogies:
1. Approaching a Target
Imagine an archer aiming for the bullseye. Each shot gets closer and closer, but might never perfectly hit the center. The bullseye represents the limit, and the arrow’s trajectory represents the function approaching the limit. The closer the arrow gets to the bullseye, the closer the function’s value gets to the limit.
2. Heating Water
Think about heating water on a stove. As the heat increases, the temperature of the water rises. The temperature approaches the boiling point (100°C), but might not everexactly* reach it due to various factors. The boiling point represents the limit, and the water’s temperature represents the function approaching that limit.
3. Approaching a Point on a Graph (Visual Representation)
Consider a simple function, f(x) = x. Let’s examine the limit as x approaches 2.“` ^ y | | | / | / | / | / |/
—–+——–> x
| | 2“`As x gets closer and closer to 2 (from both the left and the right), the value of f(x) gets correspondingly closer to 2. The limit, in this case, is 2. The graph visually demonstrates the function’s values approaching the limit.
Examples of Functions with and Without Limits
Let’s explore some functions to solidify our understanding. Functions with Limits:
- lim x→2 (x²
- 4) / (x – 2) = 4. (This limit can be evaluated by factoring and simplifying the expression.)
- lim x→0 sin(x) / x = 1. (This is a fundamental limit in calculus.)
- lim x→∞ 1/x = 0. (As x becomes infinitely large, 1/x approaches 0.)
Functions Without Limits:
1. limx→0 1/x
This limit does not exist because the function approaches positive infinity from the right and negative infinity from the left. This is an example of an infinite limit.
2. limx→0 sin(1/x)
This limit does not exist because the function oscillates infinitely between -1 and 1 as x approaches 0. This is an example of an oscillating limit.
3. limx→0 |x|/x
This limit does not exist because the function approaches 1 from the right and -1 from the left.
Evaluating Limits Graphically
A graphical approach offers a visual understanding of limits. Here’s a step-by-step procedure:
1. Locate the Point
Find the x-value at which you’re evaluating the limit on the graph.
2. Approach from the Left
Trace the graph as x approaches the point from values less than it. Observe the y-value the function seems to be approaching. This is the left-hand limit.
3. Approach from the Right
Trace the graph as x approaches the point from values greater than it. Observe the y-value the function seems to be approaching. This is the right-hand limit.
4. Compare Limits
If the left-hand and right-hand limits are equal, then the limit exists and is equal to their common value. If they are unequal, the limit does not exist. Example: Consider the function f(x) = x + 1. To find lim x→2 f(x), we observe the graph, approaching x = 2 from both sides. We see that the function approaches y = 3 from both the left and the right, so the limit is 3.
Limit Laws Summary
Understanding limit laws simplifies the evaluation of complex limits.
| Law | Mathematical Statement | Explanation |
|---|---|---|
| Sum Law | limx→c [f(x) + g(x)] = limx→c f(x) + limx→c g(x) | The limit of a sum is the sum of the limits. |
| Product Law | limx→c [f(x)
| The limit of a product is the product of the limits. |
| Quotient Law | limx→c [f(x) / g(x)] = limx→c f(x) / limx→c g(x) (provided limx→c g(x) ≠ 0) | The limit of a quotient is the quotient of the limits, provided the denominator’s limit is not zero. |
| Constant Multiple Law | limx→c [k
| The limit of a constant times a function is the constant times the limit of the function. |
| Power Law | limx→c [f(x)]n = [limx→c f(x)]n | The limit of a function raised to a power is the limit of the function raised to that power. |
One-Sided Limits
One-sided limits examine the behavior of a function as it approaches a point from either the left or the right. The existence of a limit requires that both one-sided limits are equal. Examples:Consider the function f(x) = |x|/x. The left-hand limit as x approaches 0 is -1, while the right-hand limit is 1. Since these limits are different, the limit as x approaches 0 does not exist.
A graph would show a jump discontinuity at x = 0.
Continuity and Limits
A function is continuous at a point if the limit of the function at that point exists, is equal to the function’s value at that point, and the function is defined at that point. In simpler terms, if you can draw the function without lifting your pen at a given point, it’s continuous at that point. However, a limit can exist at a point even if the function is not continuous there.
For example, consider a piecewise function where f(x) = x for x ≠ 0 and f(0) = 1. The limit as x approaches 0 is 0, but the function is not continuous at x = 0 because the limit does not equal the function value at that point.
Limit Quiz
Let’s test your understanding!
1. Question
What is lim x→2 (x + 1)? (a) 1 (b) 2 (c) 3 (d) 4 (Answer: c)
2. Question
If lim x→a f(x) = 3 and lim x→a g(x) = 2, what is lim x→a [f(x)
g(x)]?
(a) 1 (b) 5 (c) 6 (d) 9 (Answer: c)
3. Question
Based on the graph [describe a graph with a jump discontinuity at x=1, where the left-hand limit is 2 and the right-hand limit is 4], what is lim x→1 f(x)? (a) 2 (b) 3 (c) 4 (d) Does not exist (Answer: d)
4. Question
What is lim x→∞ 1/(x² + 1)? (a) 1 (b) ∞ (c) 0 (d) -∞ (Answer: c)
5. Question
What is the left-hand limit of f(x) = 1/x as x approaches 0? (a) 1 (b) 0 (c) ∞ (d) -∞ (Answer: d)
Limits at Infinity
Limits at infinity explore the behavior of a function as x grows infinitely large (positive or negative). Horizontal asymptotes represent the values the function approaches as x approaches infinity or negative infinity. Examples:The function f(x) = 1/x has a horizontal asymptote at y = 0 because lim x→∞ 1/x = 0 and lim x→-∞ 1/x = 0. A graph would show the function approaching the x-axis as x moves towards positive and negative infinity.
The function f(x) = (2x + 1)/(x -1) has a horizontal asymptote at y = 2, since the limit as x approaches infinity or negative infinity is 2.
Limit Laws and Properties: Precalculus With Limits Answers
My dear students, as we journey deeper into the fascinating world of limits, we encounter a set of powerful tools – the limit laws. These laws provide a systematic approach to evaluating limits, transforming complex expressions into manageable calculations. Understanding and mastering these laws is crucial for your success in precalculus and beyond. Let us embark on this enlightening exploration together.
Limit Laws
The limit laws provide a framework for simplifying the evaluation of limits of algebraic combinations of functions. Each law applies under specific conditions, primarily ensuring that the limit of each component function exists. The failure to meet these conditions can lead to indeterminate forms, which require further analysis.
Sum, Difference, Product, and Quotient Laws
The sum law states that the limit of a sum is the sum of the limits: lim x→a [f(x) + g(x)] = lim x→a f(x) + lim x→a g(x), provided both lim x→a f(x) and lim x→a g(x) exist. Similarly, the difference, product, and quotient laws apply to subtraction, multiplication, and division of functions, respectively. The quotient law, lim x→a [f(x) / g(x)] = [lim x→a f(x)] / [lim x→a g(x)], requires the additional condition that lim x→a g(x) ≠ 0.
Constant Multiple, Power, and Root Laws
The constant multiple law simplifies limits involving constant multiples of functions: lim x→a [cf(x)] = c lim x→a f(x), where ‘c’ is a constant. The power law allows us to evaluate limits of powers of functions: lim x→a [f(x)] n = [lim x→a f(x)] n. Finally, the root law handles limits of roots of functions: lim x→a √f(x) = √[lim x→a f(x)], provided the root is defined for the limit of f(x).
Indeterminate Forms
Indeterminate forms, such as 0/0, ∞/∞, 0·∞, ∞∞, 0 0, 1 ∞, and ∞ 0, arise when direct application of limit laws yields inconclusive results. These forms signal the need for further manipulation, such as factorization, simplification, or application of L’Hôpital’s rule (which is beyond the scope of precalculus).
Examples of Applying Limit Laws
Let’s illustrate the application of these laws with some examples. Example 1: Rational FunctionEvaluate lim x→2 [(x²
4) / (x – 2)].
Solution: We have an indeterminate form 0/0. Factoring the numerator, we get lim x→2 [(x – 2)(x + 2) / (x – 2)] = lim x→2 (x + 2) = 4. Example 2: Trigonometric FunctionEvaluate lim x→0 [(sin x) / x].Solution: This is a well-known limit, equal to 1. We can use trigonometric identities and the squeeze theorem to prove this, which is shown later.
Example 3: Exponential FunctionEvaluate lim x→∞ (e -x).Solution: As x approaches infinity, e -x approaches 0. Therefore, the limit is 0.
Summary of Limit Laws with Examples
| Law | Formal Mathematical Expression | Example | Detailed Step-by-Step Solution |
|---|---|---|---|
| Sum Law | limx→a [f(x) + g(x)] = limx→a f(x) + limx→a g(x) | limx→1 (x² + 2x) | limx→1 x² + limx→1 2x = 1 + 2 = 3 |
| Product Law | limx→a [f(x)g(x)] = [limx→a f(x)][limx→a g(x)] | limx→2 (x)(x – 1) | [limx→2 x][limx→2 (x – 1)] = (2)(1) = 2 |
| Quotient Law | limx→a [f(x) / g(x)] = [limx→a f(x)] / [limx→a g(x)], g(x) ≠ 0 | limx→3 (x²
| Factoring: limx→3 (x – 3)(x + 3) / (x – 3) = lim x→3 (x + 3) = 6 |
| Constant Multiple Law | limx→a [cf(x)] = c lim x→a f(x) | limx→0 5cos(x) | 5 limx→0 cos(x) = 5(1) = 5 |
Squeeze Theorem
The Squeeze Theorem states that if f(x) ≤ g(x) ≤ h(x) for all x in some interval containing ‘a’ (except possibly at ‘a’ itself), and lim x→a f(x) = lim x→a h(x) = L, then lim x→a g(x) = L.
This theorem is particularly useful when evaluating limits of functions that are difficult to manipulate directly. Example 1: Consider evaluating lim x→0 x²sin(1/x). Since -x² ≤ x²sin(1/x) ≤ x², and lim x→0 -x² = lim x→0 x² = 0, by the Squeeze Theorem, lim x→0 x²sin(1/x) = 0. (Imagine a graph where y = x² forms an upper bound and y = -x² forms a lower bound, with y = x²sin(1/x) oscillating wildly but always staying within these bounds.
As x approaches 0, all three functions approach 0.)* Example 2: Similarly, lim x→0 x cos(1/x) can be evaluated using the squeeze theorem, noting that -|x| ≤ x cos(1/x) ≤ |x|.
Limits at Infinity
Evaluating limits as x approaches infinity involves analyzing the behavior of the function as x grows without bound. For rational functions, we can compare the degrees of the numerator and denominator. If the degree of the numerator is less than the degree of the denominator, the limit is 0. If the degrees are equal, the limit is the ratio of the leading coefficients.
If the degree of the numerator is greater than the degree of the denominator, the limit is ∞ or -∞. Horizontal asymptotes represent the values that a function approaches as x approaches positive or negative infinity.
Limits Involving Absolute Values
Absolute value functions are piecewise defined. To evaluate limits involving absolute values, consider the limits from the left and right separately. If the left-hand limit and the right-hand limit are equal, the limit exists and is equal to that common value.
Flowchart for Evaluating Limits
(A flowchart would be visually represented here. It would begin with a decision point
Is the limit of the form f(a)? If yes, substitute a into f(x). If no, proceed to another decision point: Is it an indeterminate form? If yes, use algebraic manipulation or other techniques. If no, apply relevant limit laws.
The flowchart would guide the user through these steps, leading to the final solution.)*
Summary of Key Concepts and Strategies for Evaluating Limits: Limit laws provide a systematic approach to evaluating limits. Understanding indeterminate forms is crucial. The Squeeze Theorem helps in cases where direct application of limit laws is not feasible. Limits at infinity involve analyzing the behavior of functions as x approaches infinity. Absolute value functions require consideration of left-hand and right-hand limits.
Techniques for Evaluating Limits

My dear students, as we journey deeper into the fascinating world of limits, we now arrive at the crucial techniques that unlock their secrets. Understanding these methods is paramount to mastering precalculus and beyond. Think of these techniques as the keys to opening doors to more complex mathematical concepts. Let’s unlock them together!
Direct Substitution
Direct substitution is our first and often simplest approach to evaluating limits. It involves directly substituting the value that the variable approaches into the function. If the result is a defined real number, then that number is the limit. However, this method is not a panacea. Its simplicity belies a crucial limitation: it fails when the substitution results in an indeterminate form, such as 0/0 or ∞/∞.
These forms tell us that more sophisticated techniques are required to unveil the limit’s true nature. For instance, consider the limit of f(x) = x² + 2x as x approaches 3. Direct substitution yields 3² + 2(3) = 15. This is straightforward. However, if we had a function like (x²
9)/(x – 3), direct substitution at x=3 would yield 0/0, which is indeterminate, highlighting the limitations of this straightforward approach.
Factoring and Cancellation, Precalculus with limits answers
When direct substitution yields an indeterminate form, factoring and cancellation often emerge as our saviors. The essence of this technique lies in algebraic manipulation to simplify the expression, thereby eliminating the indeterminate form. By factoring the numerator and denominator, we often find common factors that can be canceled, revealing the true limit. Let’s illustrate with an example: Consider the limit of (x²9)/(x – 3) as x approaches 3.
Direct substitution gives 0/0. However, factoring the numerator as (x – 3)(x + 3) and canceling the common factor (x – 3) in the numerator and denominator, we obtain x + 3. Now, substituting x = 3, we find the limit to be 6. This elegantly resolves the indeterminate form. This method is a powerful tool in our arsenal, enabling us to navigate seemingly insurmountable indeterminate forms.
L’Hôpital’s Rule
While L’Hôpital’s Rule is typically introduced in calculus, a brief mention is warranted here. It provides an elegant method for handling indeterminate forms of the type 0/0 or ∞/∞. The rule states that if the limit of f(x)/g(x) as x approaches a is of an indeterminate form, then the limit is equal to the limit of f'(x)/g'(x) as x approaches a, provided the latter limit exists.
This involves taking the derivatives of the numerator and denominator separately before evaluating the limit. This powerful technique is a testament to the interconnectedness of mathematical concepts. While beyond the strict confines of precalculus, understanding its basic application provides a glimpse into the advanced techniques you’ll encounter in your calculus journey. Remember, my dear students, the mathematical landscape is vast and interconnected, and each concept builds upon the foundations laid before it.
Limits at Infinity
My dear students, as we journey deeper into the fascinating world of precalculus, we now arrive at a concept of profound elegance and practical significance: limits at infinity. This isn’t just about numbers getting bigger and bigger; it’s about understanding the ultimate behavior of functions, their asymptotic tendencies, and what they reveal about the systems they model. Prepare yourselves for a revelation!Limits at infinity explore the behavior of a function as its input (typically denoted as ‘x’) grows without bound, either towards positive infinity or negative infinity.
It’s about asking: “What happens to the function’s output as x becomes incredibly large, or incredibly small (in the negative sense)?” The answer reveals crucial information about the function’s long-term trends, its stability, and its potential for growth or decay. This understanding forms the bedrock for many advanced mathematical concepts and applications in fields like physics, engineering, and economics.
Definition of Limits at Infinity
A limit at infinity describes the value a function approaches as its input grows infinitely large (positive or negative). Formally, we say that the limit of f(x) as x approaches infinity is L, written as:
limx→∞ f(x) = L
if for any ε > 0, there exists an M such that if x > M, then |f(x)-L| < ε. A similar definition applies for the limit as x approaches negative infinity. This precise definition ensures that as x gets arbitrarily large, f(x) gets arbitrarily close to L. The value of L might be a real number, positive infinity, negative infinity, or the limit might not exist.
Examples of Evaluating Limits at Infinity
Let’s illuminate this with some examples, my inquisitive minds. Consider the function f(x) = 1/x. As x approaches positive infinity, 1/x approaches
0. Thus
limx→∞ (1/x) = 0
Conversely, as x approaches negative infinity, 1/x also approaches 0:
limx→∞ (1/x) = 0
Now, let’s consider a rational function: g(x) = (2x² + 3x)/(x²
1). To find the limit as x approaches infinity, we can divide both the numerator and denominator by the highest power of x (which is x² in this case)
limx→∞ [(2x² + 3x)/(x²
1)] = limx→∞ [(2 + 3/x)/(1 – 1/x²)] = 2/1 = 2
As x becomes very large, the terms 3/x and 1/x² become insignificant, leaving us with 2.
Visual Representation of a Function as x Approaches Infinity
Imagine a graph. Consider a function that approaches a horizontal asymptote. Draw a smooth curve representing the function. As the x-values move further to the right (towards positive infinity), the curve gets increasingly closer to a horizontal line—this line represents the limit of the function as x approaches infinity. The distance between the curve and the horizontal line decreases, but never actually reaches zero.
This visually demonstrates how the function’s output settles towards a specific value (or perhaps infinity) as the input grows without bound. The same principle applies to negative infinity, except we’d be observing the behavior of the curve as x-values move far to the left along the x-axis. This visual representation captures the essence of limits at infinity—the ultimate trend of the function.
One-Sided Limits
My dear students, as we journey deeper into the fascinating world of limits, we encounter a subtle yet crucial distinction: one-sided limits. These limits reveal the behavior of a function as it approaches a specific point from either the left or the right, providing a more nuanced understanding of function behavior near points of potential discontinuity. Prepare yourselves for a profound exploration!
Left-Hand and Right-Hand Limits
The concept of one-sided limits allows us to examine how a function behaves as it approaches a point from only one direction. The left-hand limit, denoted as lim x→a– f(x), describes the value the function approaches as
- x* approaches
- a* from values
- less* than
- a*. Similarly, the right-hand limit, lim x→a+ f(x), represents the function’s behavior as
- x* approaches
- a* from values
- greater* than
- a*.
Graphically, consider the point ‘a’ on the x-axis. The left-hand limit examines the y-values of the function as we move along the x-axis towards ‘a’ from the left (values smaller than ‘a’). The right-hand limit does the same, but approaching ‘a’ from the right (values larger than ‘a’).Example 1: Imagine a function that smoothly approaches a value of 2 asx* approaches 3 from both sides.
In this case, both the left-hand and right-hand limits would equal 2. The graph would show a continuous curve passing through (3,2).Example 2: Now, picture a function with a jump discontinuity at x = 1. Asx* approaches 1 from the left, the function might approach 1, while from the right it might approach 3. Here, the left-hand limit is 1 and the right-hand limit is 3.
The graph would show a distinct jump at x=1.Intuitively, one-sided limits help us understand the function’s behavior in a localized neighborhood of a point. They provide information about the function’s trend immediately before and after a given point.Let’s examine some examples:
- f(x) = x 2, a = 2: lim x→2– f(x) = 4 and lim x→2+ f(x) =
- 2. f(x) = 1/x, a = 0: lim x→0– f(x) = -∞ and lim x→0+ f(x) = ∞.
- f(x) = x + 1, if x < 0; x2, if x ≥ 0; a = 0: lim x→0– f(x) = 1 and lim x→0+ f(x) = 0.
Comparison of One-Sided and Two-Sided Limits
A two-sided limit, lim x→a f(x), exists if and only if both the left-hand limit and the right-hand limit exist and are equal. This is a fundamental theorem in the study of limits. Formally:
limx→a f(x) = L if and only if lim x→a– f(x) = L and lim x→a+ f(x) = L.
The following table summarizes the key differences:
| Feature | One-Sided Limit | Two-Sided Limit |
|---|---|---|
| Notation | limx→a– f(x), limx→a+ f(x) | limx→a f(x) |
| Meaning | Limit from the left/right | Limit from both sides |
| Existence | Exists if the function approaches a value as x approaches ‘a’ from the specified side. | Exists if and only if both one-sided limits exist and are equal. |
Examples where the two-sided limit exists: f(x) = x 2 (at any point a), f(x) = sin(x) (at any point a).Examples where the two-sided limit does not exist due to differing one-sided limits: f(x) = |x|/x (at a = 0), the piecewise function defined earlier (at a = 2).
Functions with Different Left and Right Limits
Discontinuities, jumps, and vertical asymptotes often indicate points where the left-hand and right-hand limits might differ. Identifying these features is crucial for analyzing function behavior.Here are four examples:
- f(x) = 1/x, a = 0: lim x→0– f(x) = -∞, lim x→0+ f(x) = ∞. The difference stems from the vertical asymptote at x =
- 2. f(x) = |x|/x, a = 0: lim x→0– f(x) = -1, lim x→0+ f(x) = 1. The difference is due to the jump discontinuity at x =
- 3. f(x) = floor(x), a = 1: lim x→1– f(x) = 0, lim x→1+ f(x) = 1. The difference is due to the step discontinuity inherent in the floor function.
- f(x) = x, if x < 1; 2, if x ≥ 1; a = 1: limx→1– f(x) = 1, lim x→1+ f(x) = 2. The jump discontinuity at x=1 causes the difference.
In each case, the differing limits reflect the function’s distinct behavior as approached from the left and right. The discontinuities create this difference.
Illustrative Example with a Table
Consider the piecewise function: f(x) = x + 1, if x < 2; x2
3, if x ≥ 2
| x | f(x) | Left-hand Limit at x | Right-hand Limit at x | Two-sided Limit at x |
|---|---|---|---|---|
| 1 | 2 | 2 | 2 | 2 |
| 1.9 | 2.9 | 2.9 | 2.9 | 2.9 |
| 2 | 1 | 3 | 1 | Does Not Exist |
| 2.1 | 1.41 | 1.41 | 1.41 | 1.41 |
| 3 | 6 | 6 | 6 | 6 |
Continuity and Limits

My dear students, as we journey deeper into the fascinating world of precalculus, we now arrive at a pivotal concept: the interplay between continuity and limits. Understanding this relationship unlocks a deeper appreciation for the behavior of functions and paves the way for more advanced mathematical explorations. It’s like understanding the rhythm of a beautiful melody – each note (limit) contributes to the seamless flow of the entire piece (continuity).The essence of continuity lies in the smooth, unbroken nature of a function’s graph.
Imagine tracing the graph with your pen – if you can do so without lifting your pen, the function is likely continuous. This intuitive understanding is formalized by precise mathematical definitions, which we will explore. The relationship between limits and continuity is profound; one cannot truly understand continuity without a solid grasp of limits. Limits describe the function’s behavior as it approaches a point, while continuity ensures the function’s value at that point aligns seamlessly with its limiting behavior.
Continuity of a Function at a Point
A function f(x) is continuous at a point x = c if three conditions are met: 1) f(c) exists (the function is defined at c); 2) lim x→c f(x) exists (the limit exists as x approaches c); and 3) lim x→c f(x) = f(c) (the limit equals the function’s value at c). These conditions ensure a smooth transition of the function’s value around the point c.
If even one of these conditions fails, the function is discontinuous at x = c. Consider the function f(x) = x². This function is continuous everywhere because for any point ‘c’, f(c) = c², lim x→c f(x) = c², and these two are equal. This is a simple example of a continuous function.
Continuity of a Function Over an Interval
A function is continuous over an interval if it is continuous at every point within that interval. For example, the function f(x) = x² is continuous over the entire real number line (-∞, ∞). However, some functions exhibit continuity only over specific intervals. Consider the function g(x) = √x. This function is continuous only over the interval [0, ∞), as the square root of a negative number is undefined.
The concept of continuity over an interval is crucial for applying many important theorems in calculus.
Examples of Continuous and Discontinuous Functions
Let’s illustrate these concepts with some examples. The function f(x) = x³ is continuous everywhere; its graph is a smooth curve with no breaks or jumps. Its limit at any point c is simply c³. In contrast, the function g(x) = 1/x is discontinuous at x = 0 because g(0) is undefined; the function approaches positive infinity as x approaches 0 from the right and negative infinity as x approaches 0 from the left.
The limit at x = 0 does not exist. Another example of a discontinuous function is the piecewise function h(x) = x if x ≠ 1; 2 if x = 1. This function has a jump discontinuity at x = 1, as the limit as x approaches 1 is 1, but the function’s value at x = 1 is 2.
These examples highlight the diverse ways functions can exhibit continuity or discontinuity, emphasizing the importance of examining the function’s behavior near each point.
The Relationship Between Limits and Continuity
The connection between limits and continuity is fundamental. Continuity at a point implies that the limit of the function as x approaches that point exists and is equal to the function’s value at that point. Conversely, if the limit of a function at a point exists and equals the function’s value at that point, the function is continuous at that point.
This elegant relationship forms the bedrock of many calculus theorems and applications. It’s like two sides of the same coin – you cannot fully understand one without grasping the other. This interconnectedness is what makes the study of functions so captivating.
Infinite Limits and Vertical Asymptotes
My dear students, we now embark on a journey into the fascinating realm of infinite limits and their visual counterparts, the vertical asymptotes. These concepts are crucial for a deeper understanding of function behavior, particularly near points where the function becomes unbounded. Prepare yourselves for a captivating exploration!Infinite limits describe the behavior of a function as its input approaches a specific value, resulting in an output that grows without bound, either towards positive or negative infinity.
This unbounded behavior is visually represented by vertical asymptotes, which are vertical lines that the graph of the function approaches but never touches. Think of them as invisible barriers that the function gets infinitely close to, yet never quite reaches. Understanding this interplay between the algebraic concept of an infinite limit and the geometric representation of a vertical asymptote is paramount.
Vertical Asymptotes and Function Behavior
Let’s delve into the mechanics. A vertical asymptote occurs at x = c if the limit of the function as x approaches c from either the left or the right is positive or negative infinity. In simpler terms, as x gets closer and closer to c, the function’s value shoots off towards infinity (either positive or negative). Consider the function f(x) = 1/x.
As x approaches 0 from the right (x → 0+), f(x) approaches positive infinity. Conversely, as x approaches 0 from the left (x → 0−), f(x) approaches negative infinity. Therefore, x = 0 is a vertical asymptote for f(x) = 1/x. The graph gets infinitely close to the y-axis but never actually touches it. Another example is the function g(x) = tan(x), which has vertical asymptotes at x = (π/2) + nπ, where n is an integer.
At these points, the tangent function becomes unbounded.
Types of Infinite Limits and Graphical Representations
Now, let’s organize our understanding with a visual aid. The table below illustrates the different types of infinite limits and how they manifest graphically. Remember, these graphical descriptions are simplified representations to aid comprehension. The actual graphs can be more complex depending on the specific function.
| Type of Limit | Graphical Description |
|---|---|
| limx→c+ f(x) = ∞ | The graph approaches the vertical asymptote x = c from the right, rising indefinitely upwards. |
| limx→c+ f(x) = -∞ | The graph approaches the vertical asymptote x = c from the right, descending indefinitely downwards. |
| limx→c- f(x) = ∞ | The graph approaches the vertical asymptote x = c from the left, rising indefinitely upwards. |
| limx→c- f(x) = -∞ | The graph approaches the vertical asymptote x = c from the left, descending indefinitely downwards. |
Limits and Piecewise Functions
My dear students, as we journey deeper into the enchanting world of limits, we encounter a fascinating new terrain: piecewise functions. These functions, defined by different expressions across different intervals, present a unique challenge, but also a beautiful opportunity to hone our understanding of limits. They are like a captivating mosaic, each piece contributing to the overall picture, and understanding their behavior at the boundaries of these pieces is key to mastering their limits.Piecewise functions, as you know, are defined differently over different intervals of their domain.
This means that the approach to a particular point may depend entirely on which piece of the function is relevant near that point. Understanding how to evaluate limits in these situations requires careful consideration of the function’s definition near the point of interest. Let’s delve into the intricacies of this concept.
Evaluating Limits of Piecewise Functions
Evaluating limits of piecewise functions involves examining the behavior of the function as the input variable approaches a specific point. The key is to identify which part of the function’s definition is relevant as the input approaches the point in question. If the point lies within an interval where the function is defined by a single expression, the limit can be evaluated directly using the techniques we’ve already mastered.
However, if the point is at a boundary where the function’s definition changes, we must carefully examine the one-sided limits.
Limits from the Left and Right of Points of Discontinuity
Consider a piecewise function where the function definition changes at a particular point, say x = c. To evaluate the limit at x = c, we must evaluate the left-hand limit (approaching from values less than c) and the right-hand limit (approaching from values greater than c). If these one-sided limits are equal, then the limit exists and is equal to their common value.
If they are unequal, the limit does not exist.Let’s illustrate this with an example: Consider the function
f(x) = x² if x < 2; 4x - 4 if x ≥ 2
Let’s evaluate the limit as x approaches 2. As x approaches 2 from the left (x < 2), we use the expression x². The limit is lim (x→2⁻) x² = 4. As x approaches 2 from the right (x ≥ 2), we use the expression 4x - 4. The limit is lim (x→2⁺) (4x - 4) = 4. Since the left-hand and right-hand limits are equal, the limit as x approaches 2 exists and is equal to 4.Now, let's consider another example where the limit does not exist. Let's say we have:
g(x) = x + 1 if x < 1; x -1 if x ≥ 1
The limit as x approaches 1 from the left is lim (x→1⁻) (x + 1) = 2, while the limit as x approaches 1 from the right is lim (x→1⁺) (x – 1) = 0. Since the left-hand and right-hand limits are different, the limit as x approaches 1 does not exist for this function.
Step-by-Step Procedure for Evaluating Limits of Piecewise Functions
A systematic approach ensures accuracy and understanding. Here’s a step-by-step procedure:
1. Identify the point
Determine the value of x at which you are evaluating the limit.
2. Identify the relevant interval
Determine which piece of the function’s definition applies as x approaches the point from the left and from the right.
3. Evaluate the one-sided limits
Evaluate the limit as x approaches the point from the left (using the appropriate expression) and from the right (using the appropriate expression).
4. Compare the one-sided limits
If the left-hand limit and the right-hand limit are equal, the limit exists and is equal to their common value. If they are not equal, the limit does not exist.
Applications of Limits in Precalculus
My dear students, we’ve journeyed through the fascinating world of limits, exploring their properties and techniques. Now, let us delve into their practical applications, revealing their power and elegance in solving real-world problems within the realm of precalculus. Limits aren’t just abstract concepts; they are the keys that unlock deeper understanding of functions and their behavior.Limits provide us with powerful tools for analyzing the intricacies of functions, allowing us to understand their behavior at specific points and over intervals.
This understanding is fundamental to many areas of mathematics and science.
Finding the Slope of a Tangent Line
The slope of a tangent line to a curve at a specific point represents the instantaneous rate of change of the function at that point. This concept is crucial in calculus, but its foundation lies firmly in the concept of limits. Consider a curve defined by the function f(x). To find the slope of the tangent line at a point (x, f(x)), we consider a nearby point (x + Δx, f(x + Δx)).
The slope of the secant line connecting these two points is given by:
msec = [f(x + Δx)
f(x)] / Δx
As the distance Δx between the points approaches zero, the secant line approaches the tangent line. The slope of the tangent line, m tan, is then defined as the limit of the slope of the secant line as Δx approaches zero:
mtan = lim Δx→0 [f(x + Δx)
f(x)] / Δx
This limit, if it exists, gives us the slope of the tangent line at the point (x, f(x)). For example, if f(x) = x², the slope of the tangent line at x = 2 is found by evaluating:
limΔx→0 [(2 + Δx)²
- 2²] / Δx = lim Δx→0 (4 + 4Δx + (Δx)²
- 4) / Δx = lim Δx→0 (4 + Δx) = 4
Thus, the slope of the tangent line to f(x) = x² at x = 2 is 4.
Analyzing Function Behavior Near Specific Points
Limits are invaluable in analyzing the behavior of functions near specific points, particularly those where the function might be undefined or exhibit unusual behavior. For instance, limits help us identify vertical asymptotes, where the function approaches infinity or negative infinity as x approaches a specific value. They also help determine whether a function is continuous at a point. Analyzing the limit as x approaches a point allows us to understand the function’s trend and predict its behavior.Consider the function f(x) = 1/x.
As x approaches 0 from the right (x → 0+), f(x) approaches positive infinity. As x approaches 0 from the left (x → 0-), f(x) approaches negative infinity. This behavior is indicative of a vertical asymptote at x =
0. The limits illustrate the function’s unbounded behavior near this point. We can express this as
limx→0+ 1/x = ∞ and lim x→0- 1/x = -∞
Another example involves piecewise functions. Understanding the limits as x approaches the points where the function definition changes is crucial for determining continuity. If the left-hand and right-hand limits are equal and match the function’s value at that point, then the function is continuous at that point. Otherwise, a discontinuity exists.
Common Mistakes in Evaluating Limits
My dear students, the path to mastering limits is paved with understanding, but also with the awareness of common pitfalls. Let us embark on a journey to illuminate these stumbling blocks, so you may confidently navigate the intricacies of limit evaluation. This exploration will not only reveal the typical errors but will also equip you with the strategies to avoid them.
Common Errors in Limit Evaluation
A thorough understanding of limit properties and techniques is crucial, yet even the most diligent student can fall prey to certain common mistakes. These errors often stem from overlooking fundamental rules or misinterpreting the behavior of functions near specific points. Identifying these pitfalls is the first step towards mastery.
- Incorrect Simplification of Expressions: Failing to properly simplify algebraic expressions before substituting values can lead to incorrect results, especially when dealing with indeterminate forms. For example, incorrectly canceling terms that are not factors can lead to an erroneous limit.
- Ignoring One-Sided Limits: For piecewise functions, neglecting to consider the appropriate function definition based on whether the limit is approached from the left or right can result in an incorrect limit value.
- Misapplication of Limit Laws: Improper application of limit laws, such as attempting to apply them when they are not valid (e.g., when dealing with indeterminate forms or discontinuous functions), will lead to incorrect solutions.
- Incorrect Handling of Infinite Limits: Misinterpreting the behavior of a function as x approaches infinity or negative infinity can lead to erroneous conclusions about the existence or value of the limit.
- Overlooking Discontinuities: Failing to recognize and properly handle discontinuities in the function can lead to incorrect limit evaluations, especially when the limit point is a point of discontinuity.
Examples of Incorrect Limit Evaluations
Let us now delve into specific instances of flawed limit calculations, categorized by the type of error made. Understanding these examples will solidify your grasp of correct methodology.
| Mistake Type | Example of Incorrect Solution | Explanation of Error |
|---|---|---|
| Incorrect Cancellation | limx→2 (x2
| Incorrectly canceled (x-2) before evaluating the limit. The correct approach involves factoring the numerator: limx→2 (x – 2)(x + 2) / (x – 2) = lim x→2 (x + 2) = 4 |
| Ignoring One-Sided Limits | limx→1 f(x), where f(x) = x, x <1; 2x, x≥1 = 1 | The limit from the left is 1, but the limit from the right is 2. Since these are unequal, the limit does not exist. |
| Misapplication of Limit Laws | limx→0 (sin x) / x = lim x→0 sin x / lim x→0 x = 0/0 = undefined | The limit laws cannot be directly applied to the indeterminate form 0/0. L’Hôpital’s rule or other techniques are required. The actual limit is 1. |
Errors in Applying L’Hôpital’s Rule
L’Hôpital’s Rule, a powerful tool, demands careful application.
Its misuse can lead to significant errors.
- Applying L’Hôpital’s Rule to Non-Indeterminate Forms: L’Hôpital’s Rule is only applicable to indeterminate forms such as 0/0 or ∞/∞. Applying it to other forms will yield incorrect results.
- Incorrect Differentiation: Errors in differentiating the numerator and denominator will lead to an incorrect result. Careful and accurate differentiation is crucial.
- Repeated Application Without Checking for Indeterminate Form: It’s essential to verify that the indeterminate form persists after each application of L’Hôpital’s Rule. Continuing to apply it without checking may lead to an incorrect result.
Strategies to Avoid Mistakes
My students, the avoidance of errors requires a multifaceted approach, a blend of carefulness and strategic thinking.
- Always Simplify First: Before substituting values, simplify the expression as much as possible. Factor, cancel common terms, and use algebraic manipulations to reduce the expression to its simplest form.
- Consider One-Sided Limits: For piecewise functions, always evaluate the left-hand and right-hand limits separately before concluding the existence and value of the limit.
- Verify the Applicability of Limit Laws: Before applying any limit law, ensure that the conditions for its application are met. Remember that limit laws don’t always apply to indeterminate forms.
- Analyze Infinite Limits Carefully: When dealing with limits at infinity, carefully consider the behavior of the function as x approaches positive or negative infinity. Use techniques like dividing by the highest power of x.
- Check for Discontinuities: Always check for discontinuities in the function at the limit point. Discontinuities can significantly affect the limit’s value or existence.
Flowchart for Evaluating Limits
Imagine a roadmap, guiding your steps through the process. This flowchart serves as that guide, highlighting crucial decision points.[A detailed flowchart would be described here if images were allowed. The flowchart would begin with the problem statement, then branch to check for direct substitution. If direct substitution works, the answer is found. If not, it would branch to identify the indeterminate form (0/0, ∞/∞, etc.).
Then, further branches would lead to appropriate techniques such as factoring, rationalizing, L’Hôpital’s rule, or considering one-sided limits. Each branch would highlight potential errors and suggest corrective actions.]
Examples of Incorrect and Correct Solutions
Let’s examine specific examples where the application of proper techniques avoids common errors.
- Example 1 (0/0): Incorrect: lim x→0 (sin x)/x = sin(0)/0 = 0/0 = undefined. Correct: Using L’Hôpital’s rule, lim x→0 (cos x)/1 = 1.
- Example 2 (∞/∞): Incorrect: lim x→∞ (2x + 1)/(x – 1) = ∞/∞ = undefined. Correct: Dividing by the highest power of x, lim x→∞ (2 + 1/x)/(1 – 1/x) = 2/1 = 2.
- Example 3 (0*∞): Incorrect: lim x→0+ x ln x = 0
(-∞) = undefined. Correct
Rewriting as lim x→0+ ln x / (1/x) and applying L’Hôpital’s rule, we get lim x→0+ (1/x) / (-1/x 2) = lim x→0+ -x = 0.
Incorrect and Correct Limit Evaluations for Piecewise Functions
Piecewise functions require careful attention to the domain.
- Example 1: Incorrect evaluation of lim x→2 f(x) where f(x) = x+1, x <2; x-1, x≥2 as 3, ignoring the different definitions of f(x) around x= 2. Correct: limx→2- f(x) = 3 and lim x→2+ f(x) = 1; therefore the limit does not exist.
- Example 2: Incorrectly assuming continuity where none exists, leading to an incorrect limit value for a piecewise function with a jump discontinuity. The correct approach would involve evaluating the left and right-hand limits separately and determining if they are equal.
Short Quiz on Common Errors
Test your understanding!
- Evaluate limx→1 (x 2
1) / (x – 1).
- Evaluate lim x→0 (sin 2x) / x.
- Evaluate lim x→∞ (3x 2 + 2x) / (x 2 – 1).
- Evaluate lim x→2 f(x) where f(x) = x 2, x < 2; x + 2, x ≥ 2.
- Evaluate lim x→∞ (e x)/(x 2).
[Answer Key would be provided here. Each problem would show the correct solution and explain the common error that might be made and how to avoid it.]
Limits Where Direct Substitution Fails
Direct substitution is not always sufficient.
- Example 1: Incorrect: lim x→1 (x 2
1) / (x – 1) = 0/0 = undefined. Correct
Factoring gives lim x→1 (x – 1)(x + 1) / (x – 1) = lim x→1 (x + 1) = 2.
- Example 2: Incorrect: lim x→0 (√(x+1)
1) / x = 0/0 = undefined. Correct
Finding solutions for precalculus problems involving limits can be challenging for students. A valuable resource for supplementary practice problems and solutions is often sought, and access to comprehensive answer keys can significantly aid in understanding the concepts. For instance, the availability of a detailed answer key, such as the one provided by gina wilson answer key , can be extremely beneficial.
This ultimately enhances a student’s grasp of precalculus with limits answers and improves problem-solving skills.
Rationalizing the numerator gives lim x→0 [(√(x+1)
1)(√(x+1) + 1)] / [x(√(x+1) + 1)] = limx→0 x / [x(√(x+1) + 1)] = lim x→0 1 / (√(x+1) + 1) = 1/2.
- Example 3: Incorrect: lim x→0 (1 – cos x) / x = 0/0 = undefined. Correct: Using L’Hôpital’s rule gives lim x→0 sin x / 1 = 0.
Limits of Sequences
The concepts of limits extend to sequences. Errors in evaluating limits of functions often mirror errors in evaluating limits of sequences. For example, incorrectly applying limit laws or failing to consider the behavior of the sequence as n approaches infinity can lead to erroneous conclusions. Consider the sequence a n = (n 2 + 1) / (2n 21).
An incorrect approach might involve saying the limit is ∞/∞, while the correct approach involves dividing by n 2 to get lim n→∞ (1 + 1/n 2) / (2 – 1/n 2) = 1/2.
Epsilon-Delta Definition and Error Avoidance
The epsilon-delta definition provides a rigorous framework for understanding limits. By explicitly defining the relationship between ε (the desired closeness of the function value to the limit) and δ (the corresponding closeness of x to the limit point), one can directly address the intuitive understanding of limits and avoid errors stemming from vague notions. For instance, the epsilon-delta definition clarifies that the function need not be definedat* the limit point, only arbitrarily close to it.
This eliminates potential errors arising from assuming the function must be defined at the limit point itself.
Practice Problems: Limits of Polynomial Functions
My dear students, let us now delve into the fascinating world of limits applied to polynomial functions. Understanding these concepts is crucial for your journey in calculus. Through careful practice, you will master the art of evaluating limits, a fundamental skill that will serve you well in more advanced mathematical explorations. These problems will solidify your understanding and prepare you for more complex challenges.
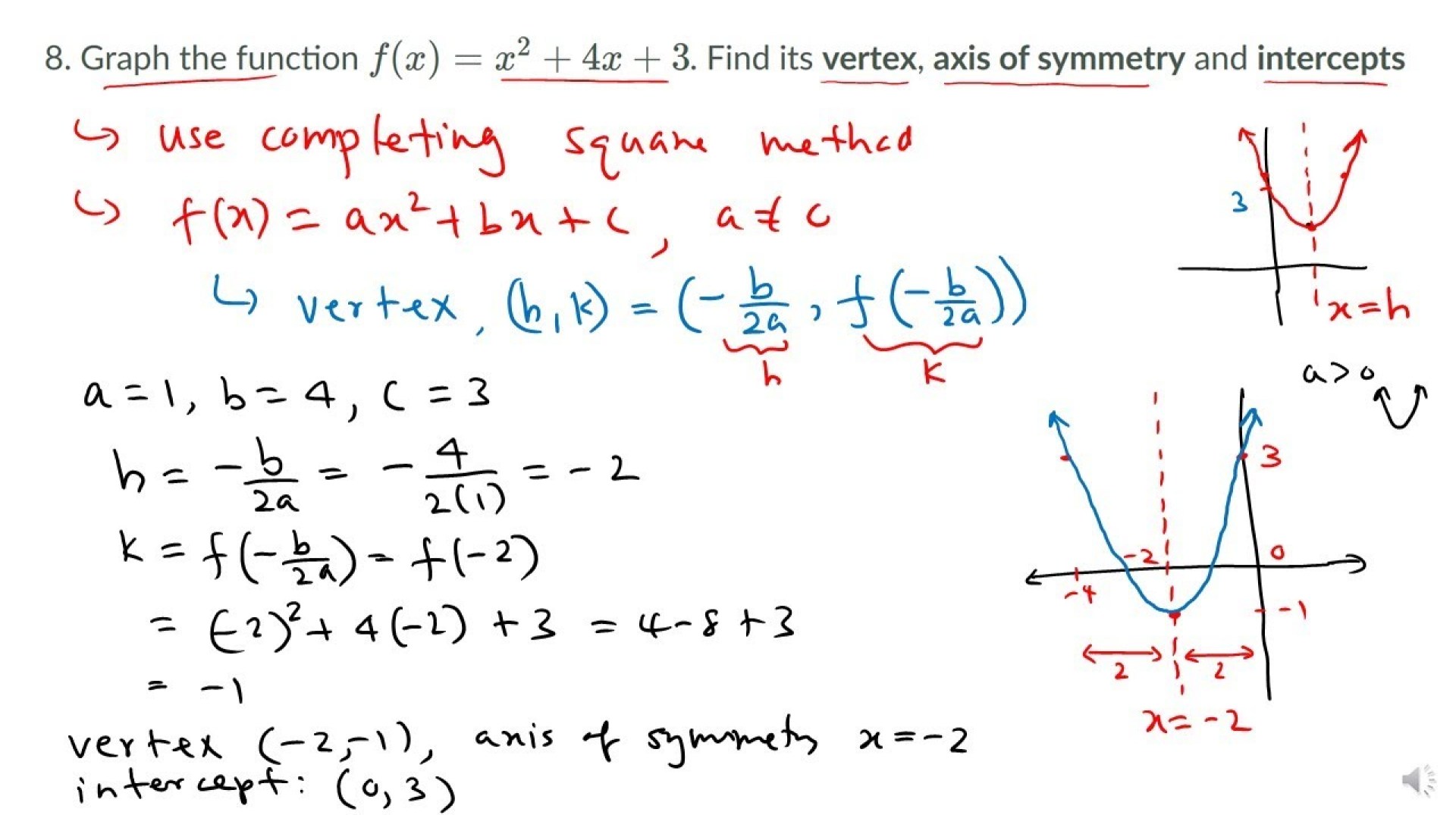
Limits of Polynomial Functions: Problem Set and Solutions
The following problems demonstrate various techniques for evaluating limits of polynomial functions. Remember, my young Padawans, the key lies in careful observation, strategic simplification, and precise application of limit laws. Each step is a brushstroke on the canvas of mathematical understanding, leading to the final masterpiece – the solution.
Problem 1: limx→2 (x²
-4)/(x – 2)- (a) Substitution: If we directly substitute x = 2, we get (2²
-4)/(2 – 2) = 0/0, an indeterminate form. This indicates that further simplification is necessary. - (b) Simplification/Factoring: We can factor the numerator as a difference of squares: x²
-4 = (x – 2)(x + 2). Thus, the expression becomes [(x – 2)(x + 2)]/(x – 2). - (c) Cancellation: We can cancel the (x – 2) terms, provided x ≠ 2 (which is true since we are considering the limit as x approaches 2, not equals 2). This simplifies the expression to (x + 2).
- (d) Re-Substitution: Now, substituting x = 2, we get 2 + 2 = 4.
- (e) State the Limit: Therefore, lim x→2 (x²
-4)/(x – 2) = 4.
- (a) Substitution: If we directly substitute x = 2, we get (2²
Problem 2: limx→∞ (3x³ + 2x)/(x³ – 5)
- (a) Substitution: Direct substitution of x = ∞ leads to an indeterminate form (∞/∞).
- (b) Simplification/Division: To handle limits at infinity involving polynomials, we divide both the numerator and the denominator by the highest power of x present, which is x³ in this case. This gives us [3 + (2/x²)]/[1 – (5/x³)].
- (c) Limit Properties: As x approaches infinity, the terms (2/x²) and (5/x³) approach 0.
- (d) Re-Substitution: The expression simplifies to 3/1 = 3.
- (e) State the Limit: Therefore, lim x→∞ (3x³ + 2x)/(x³
-5) = 3.
Problem 3: limx→-1 (x³ + 2x² + x)
- (a) Substitution: We can directly substitute x = -1 into the polynomial: (-1)³ + 2(-1)² + (-1) = -1 + 2 – 1 = 0.
- (b) Simplification/Factoring (Not Required): In this case, direct substitution works perfectly, so no further simplification is needed.
- (c) Re-Substitution (Not Applicable): No re-substitution is required.
- (d) State the Limit: Therefore, lim x→-1 (x³ + 2x² + x) = 0.
Summary Table
| Problem Number | Problem Statement | Limit Value |
|---|---|---|
| 1 | limx→2 (x²
| 4 |
| 2 | limx→∞ (3x³ + 2x)/(x³ – 5) | 3 |
| 3 | limx→-1 (x³ + 2x² + x) | 0 |
Practice Problems: Limits of Rational Functions
My dear students, let us now delve into the fascinating world of limits applied to rational functions. These problems will test your understanding of factoring, simplification, and the application of limit laws, including dealing with indeterminate forms and infinite limits.
Remember, patience and a methodical approach are key to mastering these techniques. Each step, each calculation, brings you closer to a deeper understanding. Embrace the challenge!
Limits of Rational Functions: Problems and Solutions
The following problems illustrate various techniques for evaluating limits of rational functions. We’ll progress from simpler problems to those requiring more advanced methods.
| Problem | Solution Steps | Final Answer |
|---|---|---|
| Problem 1 (Easy): Evaluate $\lim_x \to 2 \fracx^2 – 4x – 2$ |
| 4 |
| Problem 2 (Medium): Evaluate $\lim_x \to 1 \fracx^3 – 1x^2 – 1$ |
| $\frac32$ |
| Problem 3 (Hard): Evaluate $\lim_x \to 0 \frac\sin(x) – xx^3$ |
| $-\frac16$ |
In summary, evaluating limits of rational functions often involves simplifying the expression by factoring and canceling common factors. When direct substitution leads to an indeterminate form like $\frac00$, techniques like factoring or L’Hôpital’s rule become necessary. Understanding these techniques is crucial for a thorough grasp of limit calculations, and remember, my dear students, practice is the key to unlocking your full potential.
Each problem solved is a step towards mastery.
Practice Problems: Limits Involving Trigonometric Functions
My dear students, we now embark on a journey into the fascinating world of limits involving trigonometric functions. These problems often require a blend of trigonometric identities and limit properties, sharpening your understanding of both. Prepare yourselves, for the path ahead is rewarding, though it may demand careful consideration.
The following problems will test your ability to apply various techniques to evaluate limits involving trigonometric functions. Remember, patience and a systematic approach are key to success. Do not be discouraged by initial challenges; persistence will lead to mastery.
Limits of Trigonometric Functions: Problem Solving
Let us delve into the specifics. The problems below showcase the diverse ways trigonometric functions can appear within limit expressions. Pay close attention to the strategies employed in their solutions. These strategies are transferable to a wide array of similar problems.
- Problem 1: Evaluate
.
- Solution 1: We can use the known limit
. We rewrite the expression as:
. Let
. As
,
. Therefore, the limit becomes
.
- Problem 2: Evaluate
.
- Solution 2: Let
. Then, as
,
. Also,
. Thus,
. The limit becomes
.
- Problem 3: Evaluate
.
- Solution 3: We can use L’Hôpital’s Rule or multiply by the conjugate. Using the conjugate:
This simplifies to:
.
Further Exploration
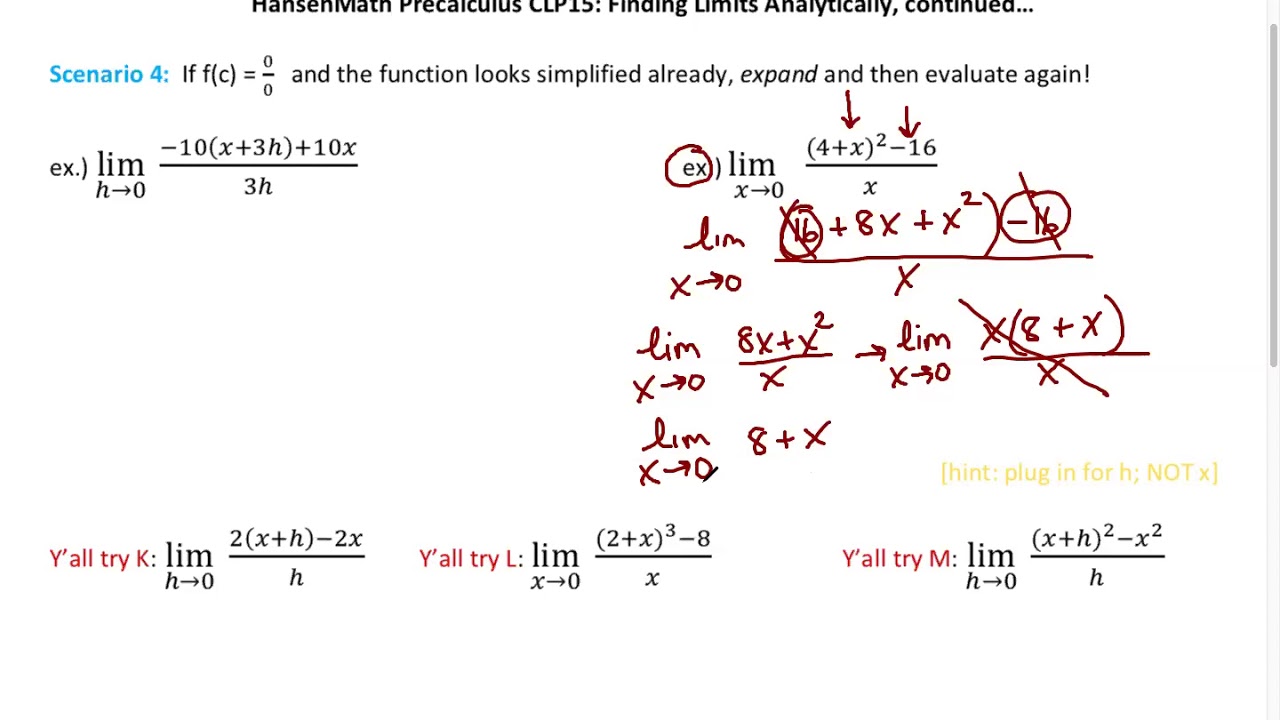
My dear students, we stand at the precipice of a new understanding, a deeper dive into the heart of calculus. Having mastered the subtleties of limits, we now embark on a journey to explore the derivative – a concept of profound beauty and immense power in unraveling the secrets of functions. Prepare yourselves for a revelation, a glimpse into the very essence of change itself.
Limit Definition of the Derivative
The derivative, my friends, is the precise mathematical measure of how a function changes at any given point. It is defined as the limit of a difference quotient, a ratio that captures the instantaneous rate of change. Formally, for a function \(f(x)\), the derivative at a point \(x\) is denoted \(f'(x)\) and is given by:
\(f'(x) = \lim_h \to 0 \fracf(x+h)
f(x)h\)
This limit exists only if the function is continuous at the point \(x\) and the limit approaches the same value from both the left and the right.
Geometric Interpretation of the Derivative
Imagine the graph of your function, a flowing curve through the landscape of numbers. The derivative at a specific point, my inquisitive minds, represents the slope of the tangent line to that curve at that very point. This tangent line, a fleeting touch, kisses the curve at only one point, revealing the instantaneous direction of the function’s change. A simple diagram would show a curve, a point on the curve, and a line tangent to the curve at that point.
The slope of this tangent line is the derivative at that point.
Illustrative Example (Polynomial)
Let’s consider the polynomial \(f(x) = x^2 + 2x + 1\). Using the limit definition:\beginalign* f'(x) &= \lim_h \to 0 \frac(x+h)^2 + 2(x+h) + 1 – (x^2 + 2x + 1)h \\ &= \lim_h \to 0 \fracx^2 + 2xh + h^2 + 2x + 2h + 1 – x^2 – 2x – 1h \\ &= \lim_h \to 0 \frac2xh + h^2 + 2hh \\ &= \lim_h \to 0 (2x + h + 2) \\ &= 2x + 2\endalign* Thus, \(f'(x) = 2x + 2\).
At \(x = 2\), \(f'(2) = 2(2) + 2 = 6\).
Illustrative Example (Non-Polynomial)
Let’s explore \(f(x) = \frac1x\). Applying the limit definition:\beginalign* f'(x) &= \lim_h \to 0 \frac\frac1x+h – \frac1xh \\ &= \lim_h \to 0 \frac\fracx – (x+h)x(x+h)h \\ &= \lim_h \to 0 \frac-hhx(x+h) \\ &= \lim_h \to 0 \frac-1x(x+h) \\ &= -\frac1x^2\endalign*Therefore, \(f'(x) = -\frac1x^2\). At \(x = 4\), \(f'(4) = -\frac116\). Note that the function is not defined at \(x=0\), and the derivative is also undefined there.
Derivative Notation
The derivative, a concept of such significance, deserves multiple expressions of its elegance. We use several notations:
- \(f'(x)\) (Lagrange’s notation): This concise notation highlights the function \(f\) and indicates the derivative.
- \(\fracdfdx\) (Leibniz’s notation): This notation emphasizes the derivative as a ratio of infinitesimals, a powerful visual representation.
- \(\fracdydx\) (Leibniz’s notation): This is used when \(y\) is explicitly defined as a function of \(x\).
Table Summarizing Key Concepts
| Concept | Definition/Explanation | Example |
|---|---|---|
| Limit Definition of the Derivative | \(f'(x) = \lim_h \to 0 \fracf(x+h)
| \(f(x) = x^2 \implies f'(x) = 2x\) |
| Geometric Interpretation | Slope of the tangent line to the graph of \(f(x)\) at a point \(x\) | See diagram above |
| Derivative Notations | \(f'(x)\), \(\fracdfdx\), \(\fracdydx\) | For \(f(x) = x^3\), \(f'(x) = \fracdfdx = \fracdydx = 3x^2\) |
Limitations of the Limit Definition
The limit definition, while fundamental, has limitations. It may not be directly applicable for functions with discontinuities at the point of interest, or for functions where the limit of the difference quotient does not exist. More sophisticated techniques are needed for such cases.
Significance of the Derivative
The derivative reveals the instantaneous rate of change of a function. Understanding this rate of change allows us to analyze the behavior of the function – where it’s increasing or decreasing, its maximum and minimum values, and its overall trend. This insight is invaluable in numerous fields, from physics and engineering to economics and biology.
Common Queries
What’s the difference between a limit and a derivative?
A limit describes the value a function approaches as its input approaches a certain value. A derivative, on the other hand, describes the instantaneous rate of change of a function at a specific point – essentially, it’s the slope of the tangent line at that point, and it’s
-defined* using a limit.
Can you have a limit that doesn’t exist?
Absolutely! Limits don’t exist when the function approaches different values from the left and right, approaches infinity, or oscillates wildly.
Why are limits important in precalculus?
Limits form the foundation of calculus. Understanding limits is crucial for grasping concepts like continuity, derivatives, and integrals.
How do I handle indeterminate forms like 0/0?
Indeterminate forms require further manipulation, usually through factoring, simplifying, or using L’Hopital’s Rule (though that’s technically calculus). The key is to algebraically rewrite the expression to get rid of the indeterminate form.