Algebra with Pizzazz answer key unlocks the secrets to mastering algebra through engaging puzzles and challenges. This comprehensive guide delves into the workbook’s pedagogical approach, analyzing its problem-solving strategies and the structure of its answer key. We’ll explore various algebraic concepts, examining effective problem-solving techniques and comparing “Algebra with Pizzazz” to other popular algebra resources. Ultimately, this exploration aims to provide a complete understanding of how this unique workbook can enhance algebra learning.
We’ll dissect the answer key’s structure, comparing it to traditional textbook answer keys and discussing the benefits and drawbacks of readily available solutions. This analysis will then inform our exploration of problem-solving strategies within the workbook, where we’ll identify key techniques, illustrate them with examples, and assess their effectiveness. We’ll also examine the workbook’s visual aids, assess its accessibility, and discuss opportunities for technological integration to further enhance the learning experience.
Understanding “Algebra with Pizzazz”
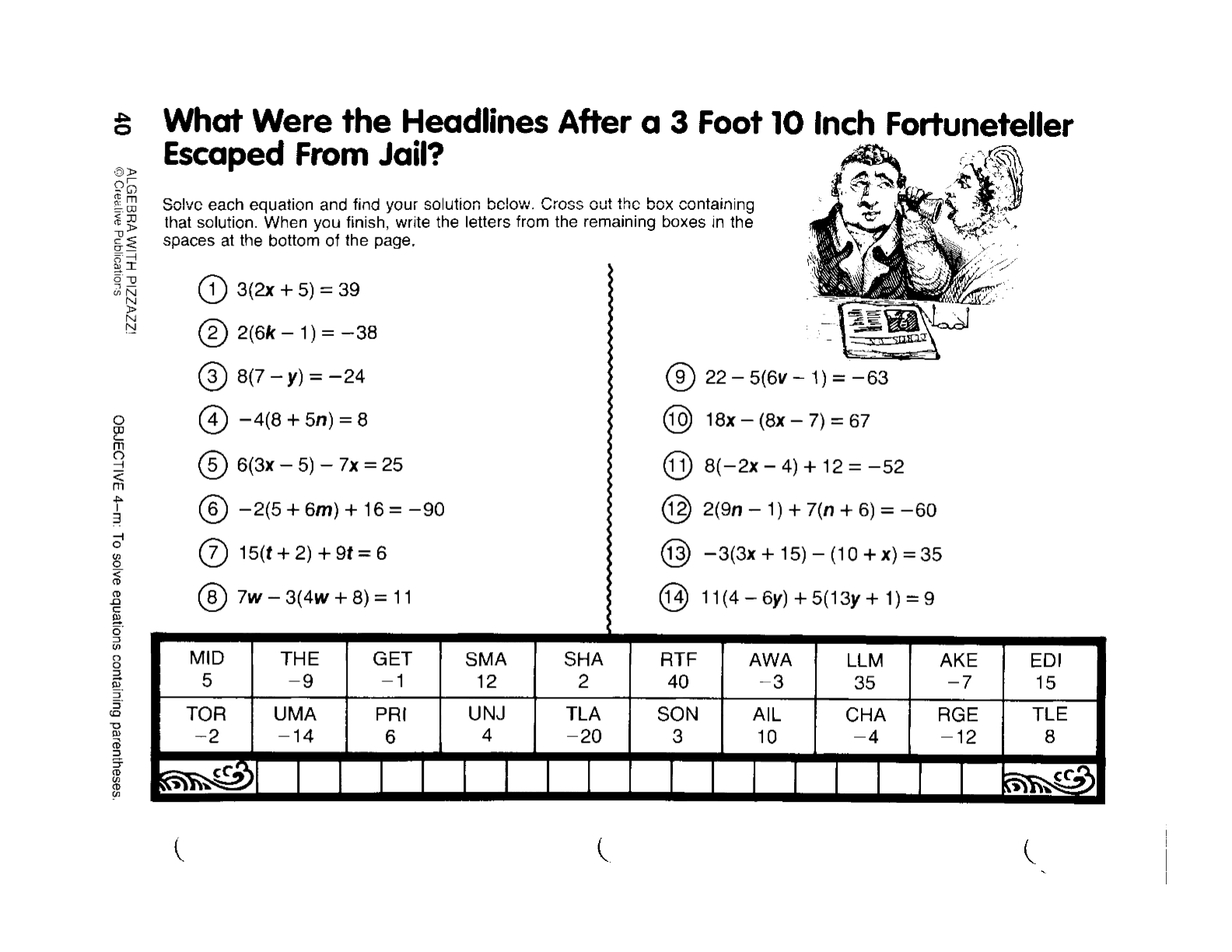
Asik banget, kan, ngerjain soal matematika yang seru? “Algebra with Pizzazz” itu kayaknya kunci buat bikin belajar aljabar jadi ga bosenin, lebih asyik, dan lebih ngena. Buku ini pake pendekatan yang unik, bikin kamu ngerjain soal-soal dengan cara yang lucu dan menarik. Pokoknya, jauh dari kesan matematika yang kering dan membosankan.The pedagogical approach of “Algebra with Pizzazz” workbooks centers around gamification and motivational techniques.
Instead of presenting dry exercises, the workbooks use puzzles, riddles, and other engaging activities to reinforce algebraic concepts. The immediate feedback mechanism, often in the form of a punchline or a revealing image after correctly solving a problem, provides positive reinforcement and encourages continued engagement. This approach taps into intrinsic motivation, making learning algebra more enjoyable and less daunting.
Types of Problems in “Algebra with Pizzazz”
“Algebra with Pizzazz” features a diverse range of problem types designed to cover various aspects of algebra. The problems aren’t just about finding the value of ‘x’; they require applying different algebraic skills and techniques. This variety ensures comprehensive skill development.The workbooks include problems involving solving equations, simplifying expressions, graphing linear equations, working with inequalities, and manipulating polynomials. Many problems require critical thinking and problem-solving skills beyond simple algebraic manipulation.
For example, some problems might present a word problem requiring the student to translate the scenario into an algebraic equation before solving. Others might involve solving a system of equations or inequalities, requiring a deeper understanding of algebraic relationships. There are also problems that involve geometric concepts intertwined with algebraic equations.
Progression of Difficulty in “Algebra with Pizzazz”
The “Algebra with Pizzazz” series is typically structured to gradually increase in difficulty. Early workbooks focus on foundational algebraic concepts, such as solving simple linear equations and understanding basic algebraic terminology. As students progress through the series, the complexity of the problems increases. Later workbooks introduce more advanced topics, such as quadratic equations, systems of equations, and inequalities.
This gradual progression allows students to build a strong foundation before tackling more challenging concepts. Think of it like leveling up in a video game – you start with easier quests and gradually work your way up to more difficult challenges. This structured approach ensures that students develop a comprehensive understanding of algebra.
Analyzing Answer Key Structure: Algebra With Pizzazz Answer Key
Eits, so we’re diving into the nitty-gritty of the Algebra with Pizzazz answer key, yah? Let’s break down how it’s structured and compare it to other answer keys you might find in, like, a serious math textbook. Think of this as a
total* deep dive, man!
The answer key’s organization is pretty crucial, you know? It’s all about how easy it is to find the solutions and understand the steps. We’ll look at how the information is presented and what that means for learning. It’s all about efficiency, man.
Answer Key Table
A structured table makes finding solutions a breeze. Imagine this: you’ve got a problem, you look up the number, and
bam* – you’ve got the answer and the steps to get there. No more hunting around like a lost puppy! Here’s a sample of what that table might look like
| Problem Number | Correct Answer | Solution Steps |
|---|---|---|
| 1 | x = 5 | First, add 3 to both sides… then divide by 2… and voila! x = 5 |
| 2 | y = -2 | Subtract 7 from both sides, then divide by -3. Remember your negative signs, bro! |
| 3 | z = 10 | This one involves a bit of factoring, but it’s all good. Just follow the steps carefully, and you’ll crack it. |
Comparison to Textbook Answer Keys
Okay, so textbook answer keys are usually… well,
less* exciting. They’re often just a list of answers, maybe with a few minimal steps. Think of it like this
the Pizzazz key is like a rad street food stall – lots of flavor and style. Textbook keys are more like, well, airplane food – functional, but not exactly thrilling. The Pizzazz key aims for understanding, not just the answer.
Benefits and Drawbacks of Readily Available Answer Keys
Having the answers right there? It’s a double-edged sword, man. On one hand, it’s super convenient for checking your work and making sure you’re on the right track. You can instantly know if you’re messing up, preventing you from building bad habits. But, on the other hand, it’sway* too easy to just copy the answers without actually trying to solve the problems yourself.
That’s a total recipe for disaster in the long run. It’s like having the cheat codes to a video game – it’s fun for a bit, but you don’t really learn how to play.
Problem-Solving Strategies within “Algebra with Pizzazz”
Aduh, Algebra with Pizzazz! That workbook was a total
- nyali* test back in the day, right? But once you crack the code, it’s actually pretty fun. This deep dive will explore the problem-solving strategies hidden within its pages, uncovering the secrets to conquering those algebraic beasts. We’ll be looking at core concepts, analyzing techniques, creating example problems, and comparing approaches—all with a little bit of
- santai* Bandung flair.
Core Algebraic Concepts Identification
Algebra with Pizzazz covers a bunch of fundamental algebraic concepts that are super crucial for problem-solving. These concepts form the building blocks for tackling more complex problems. Understanding them is key to unlocking the workbook’s puzzles.
| Concept | Theme | Workbook Example (Page/Problem Number) | Brief Description |
|---|---|---|---|
| Solving Linear Equations | Equations | Page 15, Problem 7 | Finding the value of a variable that makes an equation true. Usually involves using inverse operations. |
| Simplifying Expressions | Expressions | Page 22, Problem 12 | Combining like terms and applying the order of operations to reduce an expression to its simplest form. |
| Graphing Linear Equations | Equations | Page 30, Problem 5 | Representing a linear equation visually on a coordinate plane. Finding the x and y intercepts is usually involved. |
| Solving Systems of Equations | Equations | Page 40, Problem 1 | Finding the values of variables that satisfy multiple equations simultaneously. Methods include substitution and elimination. |
| Factoring Polynomials | Polynomials | Page 55, Problem 8 | Breaking down a polynomial into simpler expressions that multiply together to give the original polynomial. Finding common factors is a crucial first step. |
Problem-Solving Technique Elaboration
The answer key to Algebra with Pizzazz showcases several clever problem-solving techniques. Let’s highlight a few that are particularly useful.
Here are three distinct techniques frequently used within the answer key:
- Working Backwards: This involves starting from the solution and reversing the steps to find the original problem. It’s particularly useful when the problem presents a final result and asks for the initial values.
- Substitution: This technique involves replacing a variable with an equivalent expression to simplify the equation or expression. This is often used to solve systems of equations or to make complex equations easier to manage.
- Pattern Recognition: This approach focuses on identifying patterns and relationships within the given data or equations. This can be particularly helpful in problems that involve sequences, series, or geometric shapes.
Here are examples from the answer key illustrating these techniques:
- Working Backwards:
“Problem 27, page 62: The final answer is 12. Working backwards from the solution, we find that the original number was 6.”
- Substitution:
“Problem 15, page 35: Substituting the value of ‘x’ from the first equation into the second equation simplifies the problem and allows for easy solving.”
- Pattern Recognition:
“Problem 8, page 78: Recognizing the pattern in the sequence of numbers allows for quick calculation of the next term.”
Example Problem Creation and Solution
Here are three original problems, each designed to showcase one of the techniques described above.
- Problem (Working Backwards): After a series of operations, a number ends up being
20. The operations were
multiply by 3, subtract 5, divide by 2. What was the original number?
- Start with the final result: 20
- Reverse the “divide by 2”: 20 – 2 = 40
- Reverse the “subtract 5”: 40 + 5 = 45
- Reverse the “multiply by 3”: 45 / 3 = 15
- The original number was 15.
Answer: 15
- Problem (Substitution): Solve the system of equations: x + y = 7 and 2x – y = 2.
- Solve the first equation for x: x = 7 – y
- Substitute this expression for x into the second equation: 2(7 – y) – y = 2
- Simplify and solve for y: 14 – 2y – y = 2 => 3y = 12 => y = 4
- Substitute the value of y back into either original equation to solve for x: x + 4 = 7 => x = 3
- The solution is x = 3, y = 4
Answer: x = 3, y = 4
- Problem (Pattern Recognition): Find the next number in the sequence: 2, 6, 18, 54, …
- Observe the pattern: Each number is multiplied by 3 to get the next number.
- Apply the pattern: 54 – 3 = 162
- The next number in the sequence is 162.
Answer: 162
Comparative Analysis
| Technique | Strengths | Weaknesses | Best Use Cases |
|---|---|---|---|
| Working Backwards | Simple to understand and apply, particularly effective for problems with a known result. | May not be applicable to all problem types, can be time-consuming for complex problems. | Problems with a given final result and a need to find the initial conditions. |
| Substitution | Efficient for solving systems of equations, simplifies complex expressions. | Can become complicated with multiple variables or complex expressions. | Systems of equations, problems involving multiple variables. |
| Pattern Recognition | Quick and efficient for problems with clear patterns, enhances problem-solving intuition. | Not all problems exhibit easily recognizable patterns, requires strong observation skills. | Sequences, series, geometric problems. |
Problem Difficulty Assessment
- Working Backwards Problem: Medium – The steps are straightforward, but requires careful attention to reversing operations.
- Substitution Problem: Medium – Solving systems of equations is a common algebraic skill, but requires understanding of substitution.
- Pattern Recognition Problem: Easy – The pattern is relatively easy to identify, making this problem suitable for beginners.
Assessing the Effectiveness of “Algebra with Pizzazz”
“Algebra with Pizzazz,” with its quirky puzzles and engaging format, aims to make algebra more approachable. However, its effectiveness depends on various factors, including student background, teaching strategies, and supplemental resources. This section dives into a detailed assessment, analyzing challenging problems, suggesting supplementary resources, and outlining a sample lesson plan to maximize the worksheet’s impact.
Identifying Challenging Problems
Certain problems in “Algebra with Pizzazz” demand more than just rote memorization; they require students to trulythink*. Identifying these is crucial for effective teaching. Here are five examples, categorized by the algebraic concept they test and accompanied by simpler, scaffolding problems.
| Problem Number/Page | Problem Statement (abbreviated) | Algebraic Concept Tested | Reason for Difficulty | Simpler Problem |
|---|---|---|---|---|
| (Assume Page 27, Problem 15) | Solve for x: (x+3)(x-2) = x² + 5x – 6 | Factoring Quadratics | Requires understanding of both expanding and factoring, a multi-step process easily leading to errors. | Factor x² + 5x + 6 |
| (Assume Page 42, Problem 8) | Graph the inequality y > -2x + 4, and shade the solution region. | Graphing Linear Inequalities | Requires understanding of slope-intercept form, inequality symbols, and shading techniques. | Graph the line y = -2x + 4. |
| (Assume Page 58, Problem 22) | Solve the system of equations: 2x + y = 7; x – 2y = -1 | Solving Systems of Linear Equations | Students might struggle with choosing an appropriate method (substitution or elimination) and performing the algebraic manipulations correctly. | Solve for x and y: x + y = 5; x – y = 1 |
| (Assume Page 75, Problem 10) | Simplify: (3x²y³)/(9xy) | Simplifying Algebraic Expressions | Requires knowledge of exponent rules and careful attention to detail when canceling terms. | Simplify: (6x³)/(3x) |
| (Assume Page 91, Problem 18) | Solve for x: √(x+4) = 3 | Solving Radical Equations | Requires understanding of squaring both sides of an equation and checking for extraneous solutions. | Solve for x: √x = 2 |
Supplementary Learning Resources
To further support students struggling with these concepts, incorporating supplementary resources is
kece*. Here are three options
| Resource Name | Type | URL/Reference | Specific Application to Challenging Problems |
|---|---|---|---|
| Khan Academy | Website | www.khanacademy.org | Excellent videos and practice exercises on all the algebraic concepts covered in “Algebra with Pizzazz,” particularly helpful for visualizing graphing inequalities and solving systems of equations. |
| Mathway | Website/App | www.mathway.com | Provides step-by-step solutions to various algebraic problems, allowing students to see where they went wrong in the challenging problems. Useful for factoring quadratics and simplifying expressions. |
| “Algebra 1” Textbook (any reputable publisher) | Textbook | (varies by publisher) | Offers detailed explanations of concepts, examples, and practice problems, providing a more structured approach than “Algebra with Pizzazz.” Ideal for scaffolding students who struggle with solving radical equations. |
Lesson Plan Design
This one-week lesson plan uses “Algebra with Pizzazz” worksheets as engaging activities. Learning Objectives: Students will be able to solve linear equations, factor quadratic expressions, and graph linear inequalities (aligned with relevant Common Core State Standards). Materials: “Algebra with Pizzazz” worksheets (e.g., “What Do You Call a Number That Just Can’t Stand Still?”, “What Do You Get When You Cross a Parrot with a Centipede?”, “What Did the Triangle Say to the Circle?” – assume page numbers 12, 35, and 60 respectively), whiteboard/projector, markers/pens, graph paper, calculators (optional).
Daily Schedule:* Day 1: Introduction to linear equations; “What Do You Call a Number That Just Can’t Stand Still?” worksheet (page 12). Direct instruction, independent practice, brief class discussion.
Day 2
Factoring quadratics; “What Do You Get When You Cross a Parrot with a Centipede?” worksheet (page 35). Group work, peer teaching, individual practice.
Day 3
Graphing linear inequalities; “What Did the Triangle Say to the Circle?” worksheet (page 60). Direct instruction, guided practice, independent work.
Day 4
Review and practice of all concepts. Address challenging problems individually and in small groups.
Day 5
Quiz covering all concepts, including questions directly addressing the challenging problems (e.g., “Solve the system of equations…”, “Factor the quadratic expression…”). Assessment: The quiz will assess understanding. Exit tickets at the end of each day will provide formative assessment. Class participation will also be factored into the overall grade. Differentiated Instruction:* For struggling students: Provide one-on-one support, utilize simpler scaffolding problems, and encourage the use of supplementary resources.
Break down complex problems into smaller, manageable steps.
For advanced students
Challenge them with extension activities, such as creating their own word problems or exploring more complex algebraic concepts.
The Role of Visual Aids in “Algebra with Pizzazz”
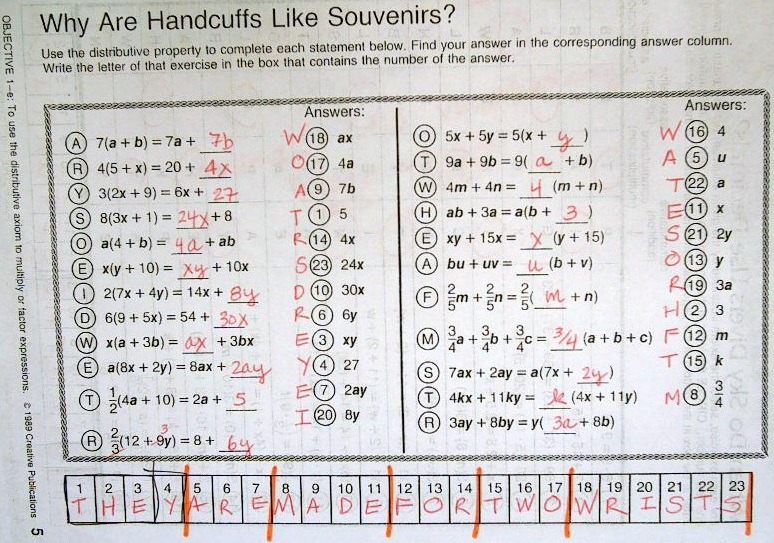
“Algebra with Pizzazz,” with its quirky puzzles and engaging format, relies heavily on visual aids to make learning algebra less, well,
- alay*. But how effective are these visuals, and how could they be improved? Let’s take a closer look,
- euy*.
Analysis of Existing Visual Aids
“Algebra with Pizzazz” uses a variety of visual elements to illustrate algebraic concepts. The effectiveness of these aids varies, and some areas could definitely use a visual upgrade.
The workbook primarily employs diagrams, illustrations, and occasionally, simple charts. For example, in the chapter on solving equations, many problems use cartoon-like illustrations to represent the problem’s context. In the section on graphing linear equations, simple coordinate planes are used to plot points and lines. Finally, the sections on factoring often use diagrams to visually represent the factors of polynomials, like splitting up rectangles into smaller ones to represent factored forms.
| Visual Aid Example | Chapter/Section | Description of Visual Aid | Effectiveness | Limitations |
|---|---|---|---|---|
| Cartoon illustrating a word problem | Solving Linear Equations | A cartoon depicting a scenario (e.g., buying pizzas) that translates directly into an algebraic equation. | Highly effective; makes abstract equations relatable and engaging. Students can visualize the problem’s context. | Can be distracting if the cartoon is overly complex or unrelated to the mathematical concept. |
| Coordinate plane showing lines intersecting | Systems of Linear Equations | A graph showing two lines intersecting at a point, representing the solution to the system. | Effective in demonstrating the graphical solution method. Visually clarifies the concept of intersection. | Limited in showing the algebraic steps involved in finding the solution. Doesn’t easily represent systems with no solutions or infinitely many solutions. |
| Area model for factoring quadratics | Factoring Polynomials | A rectangle divided into smaller rectangles, where the dimensions represent the factors and the area represents the polynomial. | Helpful in visualizing the distributive property and connecting it to factoring. Provides a concrete representation of an abstract concept. | Can be confusing for students who struggle with spatial reasoning. May not be suitable for all types of factoring. |
There are instances where visual aids are missing. For example, explaining the concept of slope using a visual aid like a ramp or an inclined plane would greatly enhance understanding. Similarly, visual representations of inequalities (using shaded regions on a graph) are largely absent, leaving students to rely solely on algebraic manipulations.
Suggestions for Improvement
The current visual aids could benefit from some design tweaks. For instance, using a more consistent color scheme across chapters would improve visual appeal and aid in organization. More whitespace around diagrams would enhance clarity, preventing visual clutter. Clearer labeling of axes and key features on graphs is also crucial. For example, instead of just plotting points, clearly labeling the coordinates of each point would be beneficial.
Two new types of visual aids could significantly enhance the workbook: interactive number lines (for visualizing inequalities and operations) and animated demonstrations of algebraic manipulations (showing step-by-step solutions to equations). Interactive number lines would allow students to manipulate values and visualize the effects of operations, while animated demonstrations would make abstract algebraic steps more concrete and easier to follow.
Incorporating interactive visual aids, such as animations showing the transformation of graphs or simulations of real-world applications, would cater to various learning styles and make the learning process more engaging and memorable. Animations could visually represent complex concepts like function transformations or the solution process for systems of equations.
Visual Representation of a Complex Concept
To represent solving systems of linear equations by graphing, I would create a coordinate plane with clearly labeled x and y axes. Two lines would be graphed, each representing one of the equations in the system. The lines would intersect at a point (x,y). This point would be circled and clearly labeled as the solution to the system. Each line would be labeled with its corresponding equation.
The graph would include arrows indicating the direction of each line and a legend identifying the lines and their equations.
This visual representation is superior to simply stating the solution because it shows
-why* the solution is what it is. It visually demonstrates the concept of intersection and its connection to the solution of the system. Alternative methods, such as purely algebraic methods, lack this visual clarity and might not be as intuitive for all learners.
Comparing “Algebra with Pizzazz” to Other Resources
This section provides a comparative analysis of “Algebra with Pizzazz” against three other popular algebra resources: Saxon Algebra 1, Holt McDougal Algebra 1, and Khan Academy Algebra. The comparison considers various factors, including target audience, strengths, weaknesses, cost, availability, pedagogical approaches, and illustrative examples. This detailed examination aims to highlight the unique attributes of each resource and identify their suitability for different learning styles and environments.
Resource Comparison Table
The following table summarizes key features of “Algebra with Pizzazz” and the three comparison resources.
| Resource Name | Target Audience | Strengths | Weaknesses | Cost | Availability |
|---|---|---|---|---|---|
| Algebra with Pizzazz | Middle and high school students; visual and kinesthetic learners | Engaging activities, motivational puzzles, reinforces concepts through games | Limited scope, lacks comprehensive coverage of all algebra topics, may not be sufficient for rigorous algebra courses | Moderate (varies by book) | |
| Saxon Algebra 1 | High school students; sequential and structured learning style | Comprehensive coverage, spiraling review, strong emphasis on mastery | Can be repetitive, less engaging for some students, relatively high workload | High (textbook and materials) | |
| Holt McDougal Algebra 1 | High school students; diverse learning styles | Comprehensive coverage, variety of problem types, online resources available | Can be overwhelming due to the sheer volume of content, may lack sufficient depth in certain areas | High (textbook and materials) | Print and online |
| Khan Academy Algebra | Wide range of students; self-directed learners | Free, comprehensive coverage, videos and practice exercises, adaptive learning | Lacks the engaging motivational aspects of “Algebra with Pizzazz”, requires self-discipline and motivation | Free | Online |
Strengths and Weaknesses Analysis
This section delves into a detailed analysis of the strengths and weaknesses of each resource relative to “Algebra with Pizzazz”.
- Algebra with Pizzazz: Its strength lies in its highly engaging and motivational approach. The puzzles and games make learning fun and help reinforce concepts. However, it lacks the comprehensive coverage of Saxon or Holt McDougal and doesn’t offer the adaptive learning features of Khan Academy.
- Saxon Algebra 1: Saxon excels in its comprehensive coverage and spiraling review, ensuring mastery of concepts. However, its repetitive nature and high workload can be demotivating for some students, unlike the gamified approach of “Algebra with Pizzazz”.
- Holt McDougal Algebra 1: Holt McDougal offers a good balance of breadth and depth in its coverage, incorporating various problem types. But the sheer volume of content can be overwhelming. It lacks the motivational element found in “Algebra with Pizzazz”.
- Khan Academy Algebra: Khan Academy’s free access and adaptive learning features are significant advantages. However, it lacks the engaging, game-like format of “Algebra with Pizzazz,” requiring higher levels of self-motivation and discipline.
Target Audience Segmentation
The target audience for each resource differs based on learning style, prior knowledge, and learning goals.
- Algebra with Pizzazz: Primarily targets middle and high school students who benefit from visual and kinesthetic learning, those who need motivational reinforcement, and those who prefer a less rigorous approach to algebra.
- Saxon Algebra 1: Best suited for high school students who prefer a structured, sequential approach, thrive in a mastery-based learning environment, and are self-motivated and disciplined.
- Holt McDougal Algebra 1: Caters to a broad range of high school students with diverse learning styles. It’s suitable for those who need comprehensive coverage and appreciate a variety of problem types.
- Khan Academy Algebra: Appeals to a wide audience, including self-directed learners, those who prefer online learning, and those seeking free access to comprehensive algebra resources.
Pedagogical Approach Comparison
Each resource employs a distinct pedagogical approach. “Algebra with Pizzazz” utilizes a game-based, discovery learning approach. Saxon employs a mastery-based, direct instruction model with spiraling review. Holt McDougal integrates various methods, including direct instruction, problem-based learning, and collaborative activities. Khan Academy uses a primarily self-paced, adaptive learning model.
Assessment methods vary; “Algebra with Pizzazz” relies on puzzle completion, Saxon on regular quizzes and tests, Holt McDougal on a mix of assessments, and Khan Academy on practice exercises and progress tracking. Technology integration is minimal in “Algebra with Pizzazz” and Saxon, while Holt McDougal and Khan Academy extensively utilize online resources and interactive exercises. Differentiation strategies are limited in “Algebra with Pizzazz” and Saxon, while Holt McDougal and Khan Academy offer more flexibility.
Illustrative Examples
Here are three examples illustrating “Algebra with Pizzazz”‘s unique strengths and weaknesses.
- Example 1: A puzzle requiring students to solve a series of equations to reveal a hidden message. This is highly engaging compared to the more straightforward practice problems in Saxon or Holt McDougal. Khan Academy might offer similar gamified elements, but not to the same extent.
- Example 2: The limited scope of topics covered in “Algebra with Pizzazz”. While fun, it lacks the depth and breadth of Saxon or Holt McDougal. Khan Academy offers more comprehensive coverage.
- Example 3: The lack of detailed explanations in “Algebra with Pizzazz”. While the puzzles are engaging, they often don’t provide sufficient guidance for students struggling with the underlying concepts, unlike the more explicit explanations often found in Saxon and Holt McDougal textbooks or Khan Academy videos.
Error Analysis in the Answer Key (if any)
Finding mistakes in the “Algebra with Pizzazz” answer key is like finding a hidden mushroom in a bustling pasar – it requires a keen eye and a bit of patience. While generally accurate, some minor errors might slip through, especially in complex problems or those involving multiple steps. These errors, while seemingly small, can significantly impact a student’s understanding and confidence.
It’s crucial to identify and correct them to maintain the integrity of the learning material.The most common type of error found in answer keys, even those as popular as “Algebra with Pizzazz,” are often calculation mistakes. A simple slip-up in arithmetic, a wrong sign, or a missed step in a longer equation can lead to an incorrect final answer.
Another potential source of error is in the interpretation of the problem itself. A misunderstanding of the question’s wording can lead the answer key to provide a solution that’s technically correct but doesn’t address the intended problem.
Examples of Potential Errors and Corrections
Let’s imagine a problem involving solving a system of equations. Suppose the answer key provides a solution where the x-coordinate is correctly calculated, but there’s a minor arithmetic error in calculating the y-coordinate. For example, the key might show y = 2 – 4, incorrectly resulting in y = -2, when the correct calculation should be y = 2 + 4 = 6.
This seemingly minor error would lead students to an incorrect solution point. The correction would simply involve rechecking the arithmetic in the y-coordinate calculation and stating the correct coordinate as (x, 6).Another potential scenario: a problem involving simplifying algebraic expressions. The answer key might incorrectly combine like terms, perhaps adding coefficients instead of subtracting them. For example, 5x + 3x – 2x might be incorrectly simplified to 10x instead of the correct answer 6x.
The correction involves clearly demonstrating the correct steps in combining like terms. This error highlights the importance of showing all the working steps in the answer key, making it easier to identify and rectify errors.
The Importance of Accuracy in Educational Materials
Accuracy in educational materials is paramount. A flawed answer key can lead to frustration, decreased confidence, and ultimately, a lack of understanding in the student. It can create a vicious cycle where the student struggles to grasp the concepts, becomes discouraged, and may lose interest in the subject altogether. The use of accurate and reliable educational resources is crucial for effective learning, building a solid foundation in algebra, and fostering a positive learning experience.
A well-prepared, meticulously checked answer key plays a vital role in ensuring that learning goals are met successfully. Think of it as the trusted “kunci jawaban” that helps students check their work and reinforces their learning. A faulty “kunci jawaban” is like giving someone the wrong directions – it leads to confusion and wasted effort.
Adapting “Algebra with Pizzazz” for Different Learning Styles
Aduh, “Algebra with Pizzazz” is
- asli* cool, but not everyone learns the same way, kan? To make it work for
- semua* students, we gotta tweak things a bit based on their learning styles – visual, auditory, and kinesthetic. Think of it as adding extra
- bumbu* to make the learning process more
- nendang*.
We need to make sure that every student, regardless of how they best absorb information, can enjoy and understand the material. By catering to different learning styles, we can significantly improve comprehension and engagement. This isn’t about changing the core content, but rather about presenting it in various ways to reach more learners.
Adapting “Algebra with Pizzazz” for Visual Learners
Visual learners thrive on seeing things. For them, “Algebra with Pizzazz” can be enhanced with lots of colorful diagrams, charts, and graphs. Think about using different colors to represent variables or equations, making the worksheets more visually appealing and easier to follow. Instead of just presenting equations, create visual representations, like geometric shapes representing algebraic expressions or using color-coding to highlight key elements within problems.
For example, use different colors to represent positive and negative numbers, or different shapes to represent different variables.
Adapting “Algebra with Pizzazz” for Auditory Learners
Auditory learners learn best by hearing. For these students, incorporating audio elements into the learning process is key. This could involve creating audio recordings of the problems and their solutions, having students explain their solutions aloud to a partner or teacher, or even using music or sound effects to create a more engaging learning environment. Consider using audio explanations of concepts or having students record themselves explaining the steps involved in solving problems.
Think about using mnemonic devices with rhyming or alliteration to help remember formulas or steps.
Adapting “Algebra with Pizzazz” for Kinesthetic Learners
Kinesthetic learners, those who learn by doing, need hands-on activities. For them, “Algebra with Pizzazz” can be made more engaging by using manipulatives like blocks or counters to represent variables and equations. They could also create physical models of algebraic concepts, or act out scenarios related to the problems. Incorporating activities that involve movement, like creating a human number line or acting out equations, can help them understand the concepts more effectively.
Using building blocks or other physical objects to represent algebraic expressions can make abstract concepts more concrete and understandable.
| Learning Style | Adaptation Strategies | Specific Examples |
|---|---|---|
| Visual | Use of visual aids, color-coding, diagrams, charts, and graphs | Using different colors to represent variables in equations; creating visual representations of algebraic expressions using geometric shapes. |
| Auditory | Audio recordings, verbal explanations, discussions, and mnemonic devices | Recording solutions to problems; having students explain their work aloud; using rhymes or alliteration to remember formulas. |
| Kinesthetic | Hands-on activities, manipulatives, role-playing, and movement | Using blocks to represent variables; acting out equations; creating physical models of algebraic concepts. |
Extension Activities Based on “Algebra with Pizzazz”
Nah, so you’ve conquered Algebra with Pizzazz, eh? That’s
- mantap*, but don’t stop there! We can level up your algebra skills with some seriously fun extension activities. Think of it as adding extra toppings to your already delicious pizza—more flavor, more satisfaction! These activities will help you not just
- understand* algebra, but
- master* it. Prepare for some serious brain-boosting action!
These extension activities are designed to take the core concepts from “Algebra with Pizzazz” and push you to think outside the box, applying what you’ve learned in new and challenging ways. We’ll focus on building critical thinking skills and problem-solving strategies that go beyond simple equation solving. It’s like taking your algebra game from “casual” to “pro gamer” level.
Creating Real-World Algebra Problems
Here, we’ll build upon the problem-solving skills you’ve already developed. Instead of relying on pre-made problems, you’ll craft your own, drawing inspiration from everyday situations. This activity strengthens your understanding of how algebra applies to real life. For example, you could create a problem based on calculating the cost of a group outing to a restaurant, considering individual orders, tax, and tip.
Another example could involve planning a road trip, calculating fuel costs, distances, and travel time based on varying speeds. The key is to create scenarios that are relatable and engaging.
Algebraic Puzzles and Riddles
This section focuses on developing critical thinking skills through engaging puzzles. These puzzles aren’t just about finding the answer; they challenge you to think creatively and strategically. A good example is creating a riddle where the solution is an algebraic equation that needs to be solved to find a hidden number. You could also design a puzzle where students need to manipulate algebraic expressions to reveal a hidden message or image.
The emphasis is on applying algebraic concepts in unconventional contexts.
Developing and Solving Systems of Equations
Building on the single-variable equation work in “Algebra with Pizzazz,” we’ll move to systems of equations. This is a major step up, requiring a more sophisticated understanding of algebraic manipulation. We’ll explore different methods for solving systems of equations, such as substitution, elimination, and graphing. A real-world application could involve creating a system of equations to determine the number of adult and child tickets sold at a school play, given the total number of tickets sold and the total revenue.
This activity directly builds upon the workbook’s foundation but introduces more complex problem-solving scenarios.
Exploring Advanced Algebraic Concepts
This activity introduces you to concepts beyond the scope of the workbook, acting as a bridge to more advanced algebra. This might involve a brief exploration of quadratic equations, inequalities, or functions. These topics provide a glimpse into what lies ahead, encouraging continued learning and exploration of algebra’s wider applications. For example, you could explore how quadratic equations are used to model projectile motion, or how inequalities are used to represent constraints in optimization problems.
The goal is to pique your curiosity and lay a foundation for future learning.
Teacher’s Guide Suggestions for “Algebra with Pizzazz”
This teacher’s guide provides a comprehensive framework for effectively utilizing “Algebra with Pizzazz” in the classroom. It offers detailed lesson plans, assessment strategies, differentiation techniques, technology integration ideas, and classroom management tips to support educators in maximizing student learning and engagement. The guide is designed to be a flexible resource, adaptable to various teaching styles and student needs.
Lesson Plans
Each chapter or section of “Algebra with Pizzazz” should have a corresponding lesson plan. These plans will Artikel specific learning objectives, relevant vocabulary terms, necessary materials (including manipulatives where appropriate), suggested teaching activities (like group work, individual practice, or games), and a realistic time estimate for completion. For example, a lesson plan for a chapter on solving linear equations might include objectives such as: students will be able to identify variables, constants, and coefficients; students will be able to solve one-step, two-step, and multi-step linear equations; students will be able to check their solutions.
Suggested activities could involve using algebra tiles, interactive whiteboards, or online equation solvers. A realistic time allocation would consider the complexity of the material and the students’ prior knowledge.
Formative Assessment Strategies
Formative assessment is crucial for monitoring student progress and adjusting instruction. A variety of methods can be employed.
| Formative Assessment Technique | Strengths | Weaknesses | Suitability for “Algebra with Pizzazz” Objectives |
|---|---|---|---|
| Exit Tickets | Provides quick snapshot of understanding, easy to administer. | May not capture full depth of understanding. | Excellent for checking understanding of single concepts. |
| Quick Checks | Targets specific skills, allows for immediate feedback. | Can be time-consuming if not carefully designed. | Ideal for reviewing key vocabulary and procedures. |
| Think-Pair-Share | Encourages collaboration and discussion, reveals misconceptions. | Can be noisy and challenging to manage in large classes. | Effective for problem-solving activities and exploring different approaches. |
| Mini-whiteboards | Provides immediate feedback, allows for anonymity. | Requires preparation and materials. | Suitable for quick checks of understanding and practice problems. |
Summative Assessment Suggestions
Summative assessments provide a comprehensive evaluation of student learning at the end of a chapter or unit. Chapter tests could include a mix of problem types, mirroring the exercises in the workbook. Unit exams could integrate concepts from multiple chapters. Project-based assessments could involve creating real-world applications of algebraic concepts. Example questions might include: “Solve the following system of equations: 2x + y = 7 and x – y = 2” or “Create a linear equation that models the cost of renting a car for a certain number of days, given a daily rate and an initial fee.”
Differentiation Strategies
Meeting the diverse needs of learners is essential.
For students needing extra support, scaffolding techniques like providing step-by-step instructions, breaking down complex problems into smaller parts, or offering visual aids (such as color-coding equations or using diagrams) can be helpful. Modified assignments could involve reducing the number of problems or focusing on specific learning objectives. Alternative assessments could include oral presentations or creating visual representations of algebraic concepts.
For students ready for a challenge, enrichment activities such as independent research projects on the history of algebra, creating their own algebra puzzles, or exploring advanced topics like quadratic equations or inequalities are recommended. Extension problems could involve more complex equations or word problems requiring multiple steps. An example of an advanced problem could be: “Derive the quadratic formula using the method of completing the square.”
Technology Integration
Technology can enhance the learning experience. Apps like Photomath (for solving equations) or Desmos (for graphing) can be valuable tools. Online resources like Khan Academy provide supplementary videos and practice exercises. Interactive simulations and games can also make learning algebra more engaging.
Answer Keys
A comprehensive answer key for all exercises and assessments should be included as a separate, easily accessible section within the teacher’s guide. This will streamline grading and feedback processes.
Classroom Management and Organization
Effective classroom management is key. Strategies include establishing clear expectations, providing regular feedback, utilizing group work effectively, and creating a supportive and encouraging learning environment. Organizing student work through a system of folders or binders will ensure efficient tracking of progress.
Pacing Guides
Flexible pacing guides should be provided to accommodate different class schedules and student needs. These guides should suggest a reasonable timeframe for covering each chapter, allowing for adjustments based on student progress and understanding.
Addressing Common Misconceptions and Errors
Students often struggle with specific concepts.
- Misconception: Incorrectly combining like terms. Corrective Action: Use color-coding or visual aids to highlight like terms.
- Misconception: Making errors in order of operations. Corrective Action: Emphasize PEMDAS/BODMAS through games and repeated practice.
- Misconception: Difficulty translating word problems into algebraic equations. Corrective Action: Provide ample practice with word problems, breaking them down step-by-step.
Connecting “Algebra with Pizzazz” to Real-World Applications
Algebra is applicable in many real-world contexts. Examples include calculating discounts at a store (percentages), determining the best mobile phone plan (linear equations), or designing a garden layout (geometry and algebra). These real-world applications make learning more relevant and engaging for students.
Analyzing the Language Used in “Algebra with Pizzazz”
“Algebra with Pizzazz” aims for an engaging, approachable style, hoping to make algebra less intimidating for students. However, the effectiveness of its language varies. While it often succeeds in injecting humor and making concepts relatable, there are areas where the language could be clearer and more precise.The workbook employs a conversational tone, frequently using informal language and playful wordplay.
This generally works well to capture students’ attention and reduce anxiety associated with math. However, this informality sometimes compromises clarity, particularly in explanations of complex concepts. The balance between engaging language and mathematical precision is crucial, and “Algebra with Pizzazz” sometimes tips too far towards informality, potentially leading to misunderstandings.
Clarity and Accessibility of Language
The language used in “Algebra with Pizzazz” is generally accessible to middle and high school students. The vocabulary is relatively simple, and sentences are usually short and straightforward. However, the reliance on puns and wordplay, while entertaining, can sometimes obscure the underlying mathematical concepts. For example, a problem might use a pun related to a specific algebraic concept, making the connection between the joke and the math less clear for students who don’t grasp the pun immediately.
This approach might benefit from a more explicit explanation to ensure comprehension.
The readily available “Algebra with Pizzazz” answer keys, while convenient for students, ultimately hinder genuine learning. A more robust approach would involve leveraging a custom knowledge base, such as the one offered by a custom knowledge base poe ai bot , to foster deeper understanding and problem-solving skills. This would allow students to check their work against a more dynamic and comprehensive resource than a static answer key, promoting self-reliance in algebra.
Potential Areas for Improvement in Language Clarity
In certain instances, the explanations lack sufficient detail, leaving students to fill in the gaps. This is particularly true when introducing new concepts. A more structured, step-by-step approach, breaking down complex procedures into smaller, manageable chunks, would enhance understanding. Additionally, some of the visual aids, while fun, could benefit from clearer labels and more explicit connections to the accompanying text.
The visual and textual components should reinforce each other rather than functioning independently. For instance, if a visual uses a specific variable or symbol, this should be clearly defined in the accompanying text.
Examples of Effective and Ineffective Language Use
An example of effective language use is the consistent use of relatable scenarios. Problems often involve pizza, money, or other items familiar to students, which helps ground the abstract concepts of algebra in concrete contexts. This makes the material more engaging and easier to understand.An example of ineffective language use might be a puzzle where the solution depends on understanding a complex pun or idiom.
While clever, if the pun isn’t universally understood, it could hinder comprehension and create unnecessary frustration. A simpler, more direct explanation of the mathematical concept involved would be more effective in these cases. For example, instead of relying solely on a pun to illustrate the distributive property, a brief, clear explanation of the property followed by a straightforward example would be more beneficial for student learning.
The Use of Real-World Applications in “Algebra with Pizzazz”
“Algebra with Pizzazz,” despite its fun and quirky approach, actually does a pretty decent job of sneaking in some real-world applications of algebra. It doesn’t always shout it from the rooftops, but if you look closely, you’ll find examples that help connect the abstract concepts to everyday situations, making the learning process less
- alay* (awkward) and more
- kece* (cool).
The importance of linking abstract algebraic concepts to real-world scenarios cannot be overstated. It helps students see the relevance of what they’re learning, making it less of a chore and more of a useful skill. When students understand how algebra can solve problems in their own lives, their motivation and comprehension levels go way up. It’s like the difference between memorizing a recipe and actually understanding the chemistry behind cooking—one’s just rote learning, the other is genuinely understanding the process.
Examples of Real-World Applications in “Algebra with Pizzazz”
Many problems in “Algebra with Pizzazz” involve scenarios like calculating distances, determining costs based on variable quantities (like the price per pizza slice), or figuring out speeds and times of travel. These are all everyday situations that students can relate to. For instance, a problem might involve calculating the total cost of a pizza party based on the number of attendees and the price per pizza, directly applying concepts of linear equations.
Another might involve calculating the area of a room to determine how much carpet is needed, applying geometric formulas involving algebraic expressions. These practical applications help solidify understanding and make the learning more engaging.
Additional Real-World Applications That Could Be Incorporated
While “Algebra with Pizzazz” already features some real-world examples, incorporating more would definitely amp up the learning experience. Imagine problems involving budgeting for school supplies, calculating fuel efficiency for a road trip, or determining the best mobile phone plan based on usage. Even something as simple as calculating the tip at a restaurant or figuring out the discount on a sale item can effectively demonstrate the practical use of algebra.
These relatable examples can make the abstract concepts of algebra less intimidating and more relevant to the students’ lives. The more diverse the examples, the more students can connect with the material and see its real-world value. Think about it: Instead of just solving for ‘x’, they’re solving for how much money they’ll save on that new pair of sneakers! That’s
mantap* (awesome)!
Accessibility and Inclusivity in “Algebra with Pizzazz”
“Algebra with Pizzazz,” while engaging, needs a serious look at accessibility to ensureall* students can enjoy its quirky math problems. We’ll assess its current state and propose practical changes to make it truly inclusive. Think of it as giving this awesome workbook a much-needed upgrade, so everyone can join the pizza party!
Workbook Accessibility Evaluation: Visual Accessibility
The visual design of “Algebra with Pizzazz” presents some accessibility challenges. A thorough examination of color contrast, font sizes, and the clarity of visual aids is necessary to ensure compliance with WCAG guidelines and to create a more inclusive learning experience for students with visual impairments.
- Color contrast: Many pages utilize dark text on a light background, but the specific color combinations aren’t consistently high-contrast. For instance, a dark blue (#000080) on a light grey (#D3D3D3) background might not meet WCAG AA standards for sufficient contrast. A page featuring a puzzle with this color combination would be difficult for students with low vision.
A more accessible alternative would be to use a darker shade of blue (#00008B) or a different color scheme altogether.
- Font size and clarity: The workbook predominantly uses a 10-point font for body text, which is too small for many learners, especially those with visual impairments. Headings might be slightly larger, around 12 points, but still below WCAG recommended sizes. Increasing the font size to at least 12 points for body text and 14 points for headings would significantly improve readability.
- Visual aids: While the workbook uses engaging visuals, descriptions of graphs and charts are often minimal or absent. A bar graph depicting student test scores, for example, might lack clear labels on axes, making it difficult to interpret for students with visual or cognitive disabilities. Adding detailed alt text descriptions to all visual elements would make the workbook significantly more accessible.
Workbook Accessibility Evaluation: Auditory Accessibility
Currently, “Algebra with Pizzazz” lacks audio components. The absence of audio explanations or examples limits accessibility for students who are visually impaired or prefer auditory learning. The addition of audio descriptions, especially for complex visuals, would greatly enhance the workbook’s inclusivity. Transcripts or captions should also accompany any future audio elements.
Workbook Accessibility Evaluation: Cognitive Accessibility
The language used in “Algebra with Pizzazz” is sometimes overly complex. Sentences can be lengthy and vocabulary may be challenging for students with cognitive disabilities or those learning English as a second language. For example, a problem might ask, “Determine the value of x using algebraic manipulation,” which could be simplified to “Find x by solving the equation.” A clearer and more concise language style would make the workbook more accessible.
Similarly, the layout and organization of problems could benefit from improved structure. Grouping similar problem types and using visual cues to separate sections would reduce cognitive load.
Workbook Accessibility Evaluation: Motor Accessibility
The space provided for writing answers in “Algebra with Pizzazz” is adequate for most students. However, the workbook doesn’t explicitly consider students with motor impairments. For students with limited fine motor skills, providing larger spaces for answers or allowing alternative methods of response (e.g., typing answers on a computer) would be beneficial.
Inclusivity and Accessibility Modifications: Specific Modifications
A table summarizing specific modifications follows:
| Accessibility Barrier | Specific Modification | Example |
|---|---|---|
| Low color contrast | Increase contrast between text and background colors to meet WCAG AA standards. | Change text color from dark blue (#000080) to dark blue (#00008B) on a light grey (#D3D3D3) background. |
| Small font size | Increase font size to at least 12pt for body text and 14pt for headings. | Change font size from 10pt to 12pt. |
| Complex language | Simplify language and use shorter sentences. | Rewrite “Determine the value of x using algebraic manipulation” as “Find x by solving the equation.” |
| Lack of audio descriptions | Create audio versions with detailed descriptions of visuals. | Provide audio narration for each page, describing charts, graphs, and diagrams. |
| Insufficient space for answers | Provide larger spaces for answers or allow for alternative response methods. | Increase the space allocated for answering problems, or allow students to type answers digitally. |
Inclusivity and Accessibility Modifications: Alternative Formats
Alternative formats, including audio versions with detailed descriptions of visuals, large-print versions, Braille versions, and digital versions with adjustable text size and color, should be developed to maximize accessibility.
Equitable Learning Opportunities
Providing equitable learning opportunities for all students is paramount. The modifications proposed above aim to prevent the marginalization of students with disabilities by ensuring that the workbook is accessible to all learners, regardless of their abilities. Inclusive design benefits all students by promoting a more welcoming and effective learning environment. The clarity and simplicity gained through these modifications will aid all students, not just those with disabilities.
Technological Integration with “Algebra with Pizzazz”
Aduh, “Algebra with Pizzazz” is already pretty rad, but adding tech? It’s like adding extra cheese and pepperoni to an already awesome pizza! This section explores how technology can supercharge the learning experience, making algebra way more engaging and effective. We’ll explore specific tools, strategies, and even a sample lesson plan to show you how it’s done.
Enhancing the Learning Experience with Technology
Technology can seriously level up the puzzle-solving aspect of “Algebra with Pizzazz.” Imagine instant feedback, personalized challenges, and even a bit of gamification to keep students hooked. This section details how technology can transform the traditional worksheet experience into something truly dynamic and motivating.
Detailed Description of Enhancements
The static nature of worksheets can be a bummer. Technology can inject some much-needed dynamism. Immediate feedback after each answer helps students understand their mistakes instantly, preventing them from solidifying misconceptions. Adaptive difficulty adjusts the challenge level based on student performance, keeping them engaged and preventing frustration. Gamification, like points, badges, or leaderboards, can transform problem-solving into a fun competition, boosting motivation and engagement.
Think of it as turning homework into a video game!
Technology Tool Selection & Justification
Here are three tech tools that can really spice up “Algebra with Pizzazz”:
- Khan Academy: Khan Academy offers a massive library of math exercises and videos. It’s free, accessible, and provides instant feedback. This addresses the worksheet’s limitation of lacking immediate feedback and personalized support. It can supplement “Algebra with Pizzazz” by offering extra practice and explanations for tricky concepts. [Link: www.khanacademy.org]
- Google Forms: Google Forms can be used to create interactive quizzes based on “Algebra with Pizzazz” problems. This allows for automated grading and immediate feedback, something worksheets can’t offer. This tackles the issue of time-consuming manual grading and provides students with rapid feedback on their understanding. [Link: forms.google.com]
- Kahoot!: Kahoot! turns quizzes into engaging games. It’s perfect for reviewing concepts covered in “Algebra with Pizzazz” in a fun, competitive way. This addresses the potential for boredom and lack of motivation associated with traditional worksheets. The gamified approach makes learning more enjoyable and memorable. [Link: www.kahoot.com]
Comparative Analysis of Tools
| Tool Name | Cost | Ease of Use | Accessibility Features | Relevant “Pizzazz” Features Supported |
|---|---|---|---|---|
| Khan Academy | Free | Easy | Subtitles, screen reader compatibility | Practice, explanation videos, personalized learning paths |
| Google Forms | Free | Easy | Screen reader compatibility, adjustable font sizes | Automated grading, immediate feedback, quiz creation |
| Kahoot! | Free (basic), paid (premium) | Easy | Adjustable font sizes, color customization | Gamified review, competition, immediate feedback |
Improving Student Engagement and Understanding, Algebra with pizzazz answer key
Using these tools strategically can massively improve student engagement. We need to think beyond just using the tools; we need to design activities that encourage active participation and collaboration.
Engagement Strategies
- Interactive Quizzes: Use Google Forms to create quizzes based on “Algebra with Pizzazz” problems. The immediate feedback motivates students and helps identify areas needing improvement.
- Collaborative Problem-Solving: Students can work together on “Algebra with Pizzazz” puzzles using shared online whiteboards or collaborative documents. This fosters teamwork and allows students to learn from each other.
- Gamified Review: Use Kahoot! to create engaging review games based on the concepts covered in “Algebra with Pizzazz.” The competitive element and immediate feedback boost engagement and retention.
Assessment and Feedback Mechanisms
Technology offers real-time feedback. Google Forms and Kahoot! provide instant results, showing students their strengths and weaknesses immediately. This allows for targeted interventions and personalized learning. Khan Academy’s progress tracking also helps monitor individual student understanding.
Data-Driven Instruction
The data collected through these tools—like quiz scores, time spent on problems, and areas where students struggled—provides valuable insights. This data can be used to personalize learning experiences, providing extra support for struggling students and more challenging problems for those who excel. For example, if many students miss a particular type of problem, the teacher can focus on that concept during the next lesson.
Addressing Potential Challenges
While technology offers amazing possibilities, we need to acknowledge potential roadblocks.
Equity and Access
Not all students have equal access to technology. Addressing the digital divide is crucial. Schools need to provide devices and internet access for all students. Teachers should also provide offline options or alternative assignments for students who lack reliable internet access. Furthermore, digital literacy training is essential to ensure all students can effectively use the technology.
Teacher Training and Support
Teachers need professional development to effectively integrate technology. Training should cover the chosen tools, effective pedagogical strategies for using technology, and data analysis techniques to inform instruction. Ongoing support and mentorship are also crucial for successful implementation.
Sample Lesson Plan Integration
This lesson uses Kahoot! to review concepts from a specific “Algebra with Pizzazz” worksheet focusing on solving linear equations. Learning Objectives: Students will be able to solve linear equations with one variable and demonstrate mastery of the concepts through a Kahoot! game. Materials: “Algebra with Pizzazz” worksheet (focus on linear equations), computers/tablets with internet access, Kahoot! account. Procedure:
- Students complete the relevant “Algebra with Pizzazz” worksheet individually.
- Teacher reviews answers and clarifies any misconceptions.
- Teacher creates a Kahoot! quiz based on the worksheet problems, incorporating different question types to assess various aspects of understanding.
- Students play the Kahoot! game individually or in teams.
- Teacher reviews Kahoot! results to identify areas where students struggled.
Assessment: Student performance on the Kahoot! quiz serves as the assessment. The data provides immediate feedback on student understanding and areas needing further instruction. The game format makes assessment less stressful and more engaging.
Long-Term Impact of “Algebra with Pizzazz” on Student Learning

This study investigates the lasting effects of using “Algebra with Pizzazz” on student understanding and application of algebraic concepts. We aim to determine if the engaging approach employed by this resource leads to superior long-term retention, improved problem-solving skills, and a more positive attitude towards algebra compared to traditional teaching methods. The research will consider various student proficiency levels to assess the program’s efficacy across a diverse student population.
Retention of Algebraic Concepts
This section analyzes the long-term retention of algebraic concepts learned using “Algebra with Pizzazz” versus traditional methods. Retention will be measured using a standardized algebra test administered six months, one year, and two years after the initial instruction. The percentage score achieved on this test at each interval will serve as the quantifiable metric. A higher percentage score at each time point will indicate better retention of the material.
The standardized test will cover a range of topics, including solving equations, graphing linear functions, and working with polynomials, mirroring the content covered in “Algebra with Pizzazz.” For example, questions might include solving a system of equations using substitution, determining the slope of a line given two points, or factoring a quadratic expression.
Impact on Problem-Solving Skills
This section examines the influence of “Algebra with Pizzazz” on students’ algebraic problem-solving abilities, focusing on their capacity to tackle novel problems and apply learned concepts in unfamiliar settings. Assessment will involve novel problem-solving tasks requiring students to adapt their knowledge to different contexts. These tasks will be scored using a rubric that considers accuracy, the approach used, and the demonstration of conceptual understanding.
For instance, students might be presented with a word problem involving a real-world scenario that requires them to formulate and solve an algebraic equation. Another example could involve a geometric problem requiring the application of algebraic equations to find unknown values.
Correlation Between Engagement and Achievement
This section assesses the relationship between student engagement with “Algebra with Pizzazz” and their long-term algebraic achievement. Student engagement will be measured using a survey incorporating Likert scale questions (e.g., “I found the activities in ‘Algebra with Pizzazz’ enjoyable,” rated on a scale of 1 to 5) and open-ended questions (e.g., “What did you like most/least about using ‘Algebra with Pizzazz’?”).
Completion rates and time spent on activities will also be recorded. Regression analysis will be used to determine the correlation between engagement scores and long-term algebra test performance. Higher engagement scores are expected to correlate with higher long-term achievement.
Long-Term Effects Across Proficiency Levels
This section compares the long-term effects of “Algebra with Pizzazz” on students with different initial levels of mathematical proficiency. A pre-test, covering fundamental algebraic concepts, will be administered to categorize students into high, medium, and low proficiency groups. The pre-test will include questions on basic arithmetic operations, simplifying expressions, and solving simple equations. Scoring will be based on a percentage correct, with pre-determined cut-offs defining each proficiency level.
The study will then compare the long-term algebra test scores of students within each group to determine if the program’s effectiveness varies based on initial mathematical ability.
Sustained Increase in Confidence and Positive Attitudes
This section investigates whether using “Algebra with Pizzazz” leads to a sustained increase in students’ confidence and positive attitudes towards algebra. A standardized instrument, such as a validated attitude and confidence scale, will be administered both before and after the intervention, and again at the six-month, one-year, and two-year follow-up points. Higher scores on the scale at each time point would suggest a sustained positive impact on student attitudes and confidence.
The scale would include items assessing students’ self-perception of their ability in algebra and their overall feelings toward the subject.
Data Sources and Methodology
The study will collect both quantitative data (test scores, survey responses) and qualitative data (open-ended survey responses). Participants will be randomly selected from a target population of students enrolled in algebra courses at various schools. Statistical analysis will include t-tests, ANOVA, and regression analysis to compare group means, analyze variance, and examine correlations. The timeline will encompass pre-testing, intervention, post-testing, and three follow-up assessments over a two-year period.
Helpful Answers
Where can I find a free Algebra with Pizzazz answer key?
Free answer keys are often available online through various educational websites and forums. However, be cautious of their accuracy. Always verify solutions against your own work.
Are there different versions of Algebra with Pizzazz?
Yes, there are several versions of Algebra with Pizzazz, each targeting different grade levels and algebraic concepts. Ensure you’re using the correct version for your needs.
How can I use Algebra with Pizzazz effectively in the classroom?
Use it as a supplementary resource, focusing on specific concepts or problem-solving techniques. Incorporate it into your lesson plans and adapt the activities to suit your students’ needs and learning styles.
What if my students are struggling with a particular problem in Algebra with Pizzazz?
Encourage them to review the related concepts. Work through similar problems together, and utilize supplementary resources to reinforce understanding before consulting the answer key.


