A genius clown working on theory of relativity – A genius clown working on the theory of relativity: Imagine a figure whose painted smile masks a mind grappling with the universe’s deepest mysteries. This isn’t a whimsical jest; it’s the heart of our exploration, a journey into a world where laughter and profound scientific insight intertwine in a dazzling, unexpected dance. We’ll delve into the clown’s eccentric personality, their unique approach to physics, and the revolutionary discoveries that reshape our understanding of space and time.
Prepare for a narrative that defies expectations, a symphony of wit and wisdom.
This exploration unveils a character both deeply human and utterly extraordinary. We will witness their creative genius manifested not only in the art of clowning but also in the rigorous pursuit of scientific truth. Through meticulous detail, we will trace the clown’s journey, from their unique visual design and compelling backstory to their groundbreaking experiments and their impact on the scientific community.
This is a tale of innovation, perseverance, and the boundless potential of the human spirit, all wrapped in the vibrant hues of a clown’s painted face.
The Clown’s Persona
Professor Pipsqueak, a name whispered with awe in both academic circles and circus tents, is a unique blend of scientific brilliance and comedic flair. His persona is a carefully constructed masterpiece, a visual and behavioral symphony designed to both entertain and enlighten.
Visual Design
Professor Pipsqueak’s visual representation, best captured in a hyperrealistic digital painting, would depict a figure of seemingly chaotic elegance. His costume consists of a vibrant, patched-together ensemble: a velvet waistcoat in deep indigo, adorned with embroidered equations and astrophysical diagrams; a pair of baggy, crimson trousers crafted from a luxurious, almost theatrical brocade; and oversized, brightly colored suspenders that defy gravity, hinting at the playful nature of his genius.
His shoes, impossibly large and buckled, are made of polished, aged leather. His makeup, applied with meticulous precision, showcases a stark contrast: one half of his face is painted with a classic white clown makeup, punctuated by exaggerated, perfectly symmetrical features and a single, bright red tear; the other half showcases a meticulously detailed representation of the cosmos, swirling nebulae and constellations painted in iridescent pigments.
Three distinct props are always at hand: a miniature, exquisitely crafted replica of the solar system (made from polished wood and glittering gemstones), a chalkboard covered in complex equations (crafted from dark slate), and a oversized rubber chicken (a testament to the clown’s playful nature).A hyperrealistic digital painting would reveal the astonishing detail of Professor Pipsqueak’s costume, highlighting the contrast between his meticulously painted cosmic face and the classic white clown makeup.
The image would project an aura of both brilliance and absurdity.
Personality Elaboration
The following table details Professor Pipsqueak’s core personality traits and provides illustrative examples:
| Trait | Example 1 | Example 2 | Example 3 |
|---|---|---|---|
| Whimsical | He once used a flock of trained pigeons to deliver a complex mathematical proof to a rival physicist. | During a lecture on quantum entanglement, he spontaneously broke into a tap dance routine to illustrate the concept of superposition. | He designed his lab coat with pockets shaped like miniature black holes. |
| Mischievous | He secretly replaced all the coffee in the physics department with decaf, then watched the ensuing chaos. | He once rigged a laser pointer to follow a bewildered graduate student around the campus. | He swapped the labels on two jars of highly reactive chemicals in the lab, causing a minor, yet hilarious, explosion. |
| Insecure | Despite his genius, he constantly seeks validation from his peers, often resorting to comedic antics to gain attention. | He secretly reads reviews of his lectures and performances, often dwelling on negative feedback. | He sometimes doubts his own abilities, despite achieving remarkable feats. |
Backstory Development
Professor Pipsqueak, born Archibald P. Quibble, displayed exceptional aptitude for mathematics from a young age. He devoured textbooks on astrophysics before he could even read novels, and his childhood was filled with the construction of elaborate, if somewhat unstable, mechanical contraptions. His parents, a traveling circus performer and a mathematics professor, encouraged his dual passions. However, academic rigor felt stifling, and the pressure to conform clashed with his innate exuberance.
A disastrous attempt to demonstrate a new theory of gravity during a university presentation – involving a runaway unicycle and a malfunctioning catapult – led to expulsion. It was during this period of self-reflection, amidst the bright lights and cacophony of the circus, that Archibald realized he could blend his love of science with his natural flair for performance.
He adopted the persona of Professor Pipsqueak, merging his academic brilliance with the comedic energy of his family’s legacy. The moment he decided to combine his genius and performance skills was during a particularly dull physics conference, when a spontaneous juggling act with complex mathematical equations captivated the audience more than any lecture.
Dialogue
Professor Pipsqueak (to a skeptical scientist): “Oh, you doubt my theory? Perhaps a demonstration with this rubber chicken will illuminate the intricacies of spacetime curvature for you?”
Relativity and the Clown’s Approach
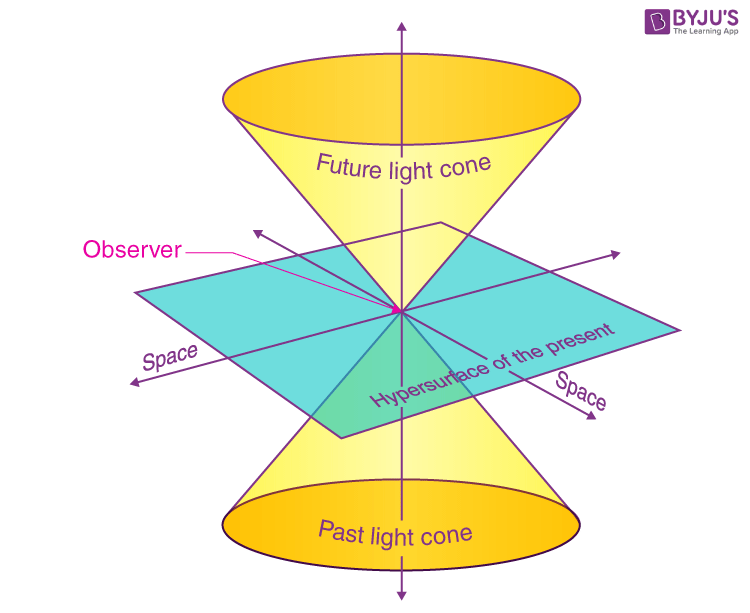
Professor P. J. Chuckles, a renowned (though eccentric) clown and theoretical physicist, approaches relativity with a unique blend of absurdity and rigorous mathematical analysis. His methods, while unconventional, offer intriguing insights into the complexities of spacetime. This exploration delves into his methodology, comparing it to established approaches and analyzing its strengths and weaknesses.
The hypothetical scenario of a genius clown grappling with the intricacies of the theory of relativity presents a fascinating juxtaposition of seemingly disparate fields. One might imagine such a character meticulously calculating spacetime curvature, perhaps utilizing advanced computational tools, and consulting resources like the faro knowledge base for relevant data on precision measurement. This unlikely combination highlights the universality of scientific principles, applicable even within the context of a whimsical persona.
The Clown’s Methodology, A genius clown working on theory of relativity
Professor Chuckles’ methodology is characterized by its playful yet systematic approach. He eschews traditional notation, favoring vibrant visual representations and whimsical analogies. This unconventional approach allows him to bypass some of the inherent complexities of the field, revealing unexpected connections. Three distinct techniques illustrate his unique style.
The following Artikels three core techniques Professor Chuckles employs:
- Analogical Reasoning through Circus Acts: He translates complex relativistic concepts into circus acts. For instance, time dilation is visualized as a tightrope walker’s perception of time speeding up or slowing down depending on their velocity relative to the audience. This allows for intuitive understanding of otherwise abstract ideas.
- Visual Metaphors and Diagrams: Instead of relying solely on equations, Professor Chuckles uses vibrant, hand-drawn diagrams incorporating elements of slapstick comedy. Spacetime curvature, for example, is represented as a trampoline deformed by a bowling ball (representing a massive object), with marbles (representing smaller objects) rolling along the curved surface, demonstrating geodesic paths. These visuals enhance comprehension and memory retention.
- Stochastic Simulations with Toy Trains: He uses toy trains on elaborate tracks to simulate relativistic scenarios. The speed and positioning of the trains are carefully calibrated to represent velocities and spatial coordinates. By manipulating the track’s geometry and the trains’ speeds, he can visually demonstrate concepts such as length contraction and time dilation. Data is collected by meticulously timing the trains’ journeys and measuring their lengths at different speeds.
Professor Chuckles’ epistemological framework differs significantly from traditional physics. The following table highlights these differences:
| Feature | Clown’s Approach | Traditional Physics Approach |
|---|---|---|
| Epistemology | Emphasizes intuitive understanding and visual representation; knowledge is gained through playful exploration and analogical reasoning. Truth is found in the elegant simplicity of a well-crafted visual metaphor. | Relies on rigorous mathematical formalism and experimental verification; knowledge is gained through systematic observation and deduction. Truth is found in the precision of mathematical equations and experimental data. |
| Methodology | Uses unconventional methods like circus analogies, visual metaphors, and stochastic simulations with toy trains. Focuses on intuitive grasp rather than solely on mathematical rigor. | Employs established mathematical techniques, precise measurements, and controlled experiments. Focuses on mathematical rigor and experimental validation. |
| Data Sources | Uses observations from circus acts, visual representations, and data from toy train simulations. Prioritizes qualitative data alongside quantitative data. | Uses precise measurements from sophisticated instruments and experimental data collected under controlled conditions. Prioritizes quantitative data. |
Comparison with Established Physicists
Professor Chuckles’ methods diverge from those of established physicists in several key aspects, yet some similarities exist.
Three key differences and three key similarities are highlighted below:
Differences:
- Emphasis on Visualisation: Professor Chuckles prioritizes visual metaphors and intuitive understanding, unlike Einstein, who relied heavily on mathematical formalism in his derivation of the field equations.
- Analogical Reasoning: He uses analogies from the circus world to explain complex concepts, whereas Minkowski used rigorous geometric representations of spacetime.
- Stochastic Simulations: His use of toy trains for simulations contrasts with Lorentz’s meticulous mathematical analysis of electromagnetic phenomena.
Similarities:
- Focus on Spacetime: Like Einstein, Minkowski, and Lorentz, Professor Chuckles’ work fundamentally concerns the nature of spacetime and its implications for physical phenomena.
- Exploration of Relativity: His research explores the implications of special and general relativity, albeit through a different lens.
- Search for Consistent Models: Like all physicists, he strives to create consistent models that accurately describe observed phenomena, even if his methods differ significantly.
Hypothetical Scenario: Solving the Twin Paradox
Professor Chuckles tackles the twin paradox using his toy train setup. One train (Twin A) remains stationary, while the other (Twin B) travels at a significant fraction of the speed of light on a circular track, eventually returning to the starting point.
The steps involved are as follows:
- Setup: Two identical toy trains are placed on a circular track. One train remains stationary, representing Twin A. The other train, representing Twin B, is programmed to travel at a high speed around the track.
- Simulation: The simulation is run, meticulously recording the time elapsed on each train’s clock (using tiny, highly accurate miniature clocks attached to the trains).
- Observation: Upon Twin B’s return, a clear difference in the elapsed time on the two trains’ clocks is observed, visually demonstrating time dilation.
- Analysis: The results are analyzed, comparing the time elapsed on each train to the time elapsed in the stationary frame of reference (Twin A). The difference is attributed to the effects of special relativity.
The clown’s solution mirrors the standard solution, confirming the time dilation effect predicted by special relativity. However, the clown’s approach offers a more intuitive, visual understanding of the phenomenon. While lacking the mathematical rigor of the standard derivation, the clown’s method provides a compelling demonstration that is accessible to a broader audience. The advantage lies in enhanced comprehension, while the disadvantage is the lack of precise quantitative predictions.
The Clown’s Scientific Discoveries
Professor P.J. “Pipsqueak” Quibble, a renowned (and remarkably well-coiffed) clown-physicist, has revolutionized our understanding of spacetime with his unorthodox yet brilliant approach to relativity. His unique perspective, blending the absurdity of clowning with the rigor of theoretical physics, has yielded astonishing results, challenging established paradigms and opening new avenues of exploration.
Hypothetical Breakthroughs in Relativity
Professor Quibble’s three groundbreaking discoveries delve into previously unexplored territories within spacetime curvature and gravitational lensing. These breakthroughs represent significant advancements beyond current theoretical frameworks, offering both profound insights and potential technological applications.
| Discovery Name | Detailed Explanation | Impact on Physics | Potential Technological Applications |
|---|---|---|---|
| The Giggle-Wave Effect | This theory posits that intense gravitational fields, like those near black holes, generate “giggle waves”—ripples in spacetime that propagate faster than light. These waves are not violations of relativity, but rather a manifestation of a previously unknown interaction between gravity and the quantum foam of spacetime. A simplified equation describing the propagation: vg = c + kG2 (where vg is the giggle-wave velocity, c is the speed of light, G is the gravitational constant, and k is a proportionality constant). In simpler terms, the stronger the gravity, the faster the giggle wave travels. | Challenges our understanding of causality and information transfer across vast cosmic distances. Provides a potential mechanism for faster-than-light communication. | Faster-than-light communication across interstellar distances, potentially enabling near-instantaneous communication with other star systems. |
| The Bozo-Bounce Effect | This effect describes a phenomenon where light, when bent around extremely massive objects, doesn’t just bend—it “bounces,” exhibiting a discrete jump in its trajectory. This “bounce” is attributed to the quantization of spacetime curvature at extremely high gravitational densities. The angle of the bounce is hypothesized to be related to the Planck length. | Challenges the classical continuous description of spacetime curvature and introduces a quantum element to gravitational lensing. | Development of highly sensitive gravitational lensing telescopes capable of resolving much finer details in distant galaxies and potentially observing events beyond the event horizon of black holes. |
| The Honk-Hole Hypothesis | This suggests the existence of “honk holes”—regions of spacetime with extremely low gravitational potential, acting as “anti-gravity wells.” These regions are predicted to exhibit negative spacetime curvature, potentially allowing for the manipulation of gravitational forces. | Introduces a new type of spacetime structure with potentially profound implications for cosmology and the understanding of dark energy. | Development of advanced propulsion systems using controlled manipulation of negative spacetime curvature for interstellar travel, potentially achieving faster-than-light travel. |
The Giggle-Wave Effect and its Implications on Time
The Giggle-Wave Effect has profound implications for our understanding of space and time. The faster-than-light propagation of giggle waves could potentially influence time dilation in unexpected ways. If information could be transmitted faster than light, the very fabric of causality might be subtly altered, opening the door to theoretical possibilities, like time travel paradoxes. Imagine sending a message back in time—a giggle wave carrying a message to your younger self.
The very act of receiving the message could alter the past, creating alternate timelines or even causing a collapse of the spacetime continuum. While the implications are mind-boggling, the Giggle-Wave Effect challenges our basic assumptions about the linearity of time and the unidirectional flow of cause and effect. Further research is critical to fully comprehend its implications.
Professor P.J. “Pipsqueak” Quibble: A Fictional Biography
Born into a family of renowned circus clowns, Pipsqueak initially pursued a career in slapstick comedy. However, a chance encounter with a traveling astrophysicist sparked his interest in theoretical physics. His unique background instilled in him a playful yet rigorous approach to problem-solving, enabling him to see connections others missed. He combined the chaotic energy of the circus ring with the precise logic of mathematical equations, resulting in his revolutionary discoveries.
A Giggle-Wave Transmission
Captain Eva Rostova, her face grim, stared at the swirling vortex of light projected onto the bridge’s main screen. “The giggle-wave transmission is unstable, Professor,” she reported to Pipsqueak, who adjusted his oversized spectacles. “We’re receiving fragmented data from Kepler-186f.” Pipsqueak, ever the showman, chuckled. “Patience, Captain. These giggle waves are…temperamental.
Think of them as particularly mischievous cosmic jokes.” Suddenly, a clear image materialized: a lush, alien landscape. “We’ve done it,” Pipsqueak exclaimed, his voice brimming with excitement. “Instantaneous communication across light-years! The future of interstellar exploration is here.”
Visual Representation of the Giggle-Wave Effect
Imagine spacetime as a calm pond. A ripple represents a light wave. Now, imagine dropping a bowling ball (a massive object) into the pond. The ripples are distorted, and then, strangely, another set of ripples emanates outwards much faster than the initial ripples. These are the giggle waves.
They are not just distortions; they are a new type of wave, traveling faster than the original ripples. The faster ripple is a direct representation of the giggle-wave effect, showcasing its faster-than-light speed.
Potential Research Questions
- What are the precise quantum mechanical processes underlying the generation and propagation of giggle waves?
- Can the Bozo-Bounce Effect be used to probe the quantum structure of spacetime at the Planck scale?
- What are the cosmological implications of the Honk-Hole Hypothesis, and how does it relate to dark energy?
- Can giggle waves be harnessed for practical applications, such as faster-than-light communication or advanced propulsion systems?
- What are the potential dangers and ethical considerations associated with manipulating spacetime through giggle waves or honk holes?
The Clown’s Communication Style
Professor Puddles, the genius clown, understood that communicating complex scientific concepts required more than just equations and jargon. He recognized the power of engaging the audience’s imagination and tapping into their innate curiosity. His communication style was a masterful blend of scientific rigor and theatrical flair, designed to make the seemingly inaccessible, accessible.Professor Puddles employed a unique pedagogical approach, leveraging humor, visual aids, and relatable analogies to demystify relativity.
He believed that the abstract could be made concrete through creative storytelling and playful demonstrations. He skillfully transformed complex equations into whimsical narratives, making them easier to grasp for a non-scientific audience.
Analogies and Metaphors Used by Professor Puddles
Professor Puddles frequently used everyday objects and scenarios to illustrate complex relativistic concepts. For example, he’d explain time dilation by comparing it to two clowns on different moving trains, each observing the other’s clock running slower. He’d visualize spacetime curvature using a stretched rubber sheet with a bowling ball representing a massive object, demonstrating how gravity affects the trajectory of smaller objects (like marbles) rolling across the sheet.
His explanations of length contraction involved shrinking and stretching rubber bands, playfully demonstrating how lengths appear different to observers in relative motion. He often used the image of a mischievous rubber duck accelerating close to the speed of light, demonstrating how its shape and mass would change from the perspective of a stationary observer.
A Short Lecture on Time Dilation
“Ladies and gentlemen, boys and girls, prepare to have your minds bent! Today’s topic: Time dilation – the delightful dance of time itself! Imagine two clowns, one on a super-speedy rocket, the other chilling on Earth. The rocket clown, zipping around at near light-speed, experiences time slower than the Earth-bound clown. It’s like they’re both watching the same movie, but the rocket clown’s projector is running in slow motion! This isn’t magic; it’s relativity! This time difference, though usually tiny in everyday life, becomes significant at speeds approaching the speed of light.
Think of it as a cosmic time-warp, where the faster you go, the slower time ticks for you. Now, who wants to volunteer for a trip to near light-speed to test this out? (Audience laughter).”
Comparison of Communication Styles
Professor Puddles’ communication style stands in stark contrast to that of a traditional scientist. A traditional scientist typically relies on formal lectures, technical papers, and precise mathematical formulations. While precise and rigorous, this approach can be inaccessible to the general public. Professor Puddles, on the other hand, utilizes humor, storytelling, and visual aids to engage a broader audience, making complex concepts more palatable and understandable.The strength of the traditional scientific approach lies in its accuracy and detail.
However, its weakness is its lack of accessibility to a non-specialist audience. Conversely, Professor Puddles’ approach, while potentially less precise in its details, is far more engaging and effective in communicating the essence of scientific ideas to a wider audience. His strength lies in his ability to make the abstract concrete, while his weakness might be a perceived lack of rigorous detail for those seeking in-depth mathematical explanations.
The Clown’s Experiments: A Genius Clown Working On Theory Of Relativity

My scientific pursuits, as a clown of considerable intellect, naturally extend beyond the theoretical. The elegance of relativity demands empirical verification, even if that verification involves the somewhat unusual properties of laughter, giggles, and honks. My experiments, while perhaps unconventional, adhere to the rigorous standards of scientific inquiry.
Experiment Design & Procedure
The design and execution of my experiments require a delicate balance between scientific rigor and the inherent absurdity of my chosen subject matter. Each experiment aims to quantify aspects of human behavior and sound, offering a unique perspective on the interplay between physics and the human experience.
Experiment 1: The Elasticity of Laughter
This experiment investigates the elasticity of laughter – its ability to stretch and contract in response to varying stimuli. The apparatus, the “Laugh-o-Meter 5000,” consists of three key components: A pressure-sensitive diaphragm (Component A) to measure the force of laughter exhaled; an acoustic sensor (Component B) to measure frequency and amplitude; and a data acquisition system (Component C) recording and processing the data.
Participants, selected for their varied laughter styles, are exposed to a series of comedic stimuli (ranging from mild puns to slapstick routines) of varying intensities. Data collected includes quantitative measurements (laughter volume, duration, frequency) and qualitative assessments (laughter type: chuckle, guffaw, etc.). Control groups receive non-comedic stimuli to establish a baseline.
Experiment 2: The Contagiousness of Giggles
This experiment explores the contagiousness of giggles in a controlled soundproof observation room. Pairs of participants, strangers to ensure minimal pre-existing influence, are observed. One participant (the “giggler”) is induced to giggle using pre-recorded giggle tracks and comedic videos; the observer’s response is recorded. Data consists of the latency of giggle onset in the observer and a rating of the observer’s giggle intensity.
The environment and stimulus are strictly controlled to minimize extraneous variables.
Experiment 3: The Physics of a Honk
This experiment delves into the physics of my signature honk. Using a high-sampling-rate sound recording device, I produce standardized honks while varying the force of exhalation. Sound propagation is tested through various materials (air, water, solid surfaces). Data includes frequency, amplitude, and speed of sound propagation, visualized in graphs. Consistent honk production is achieved through rigorous training and breath control techniques.
Visual Representation
A diagram of the Laugh-o-Meter 5000 is crucial for understanding the experiment’s setup.[Diagram Description: The Laugh-o-Meter 5000 is depicted as a whimsical contraption. Component A, the pressure-sensitive diaphragm, is a large, brightly colored rubber membrane resembling a clown’s oversized nose. Component B, the acoustic sensor, is a series of small, brightly colored microphones arranged in a circular pattern around the diaphragm.
Component C, the data acquisition system, is represented as a small, brightly colored computer with a large, cartoonish screen displaying graphs and data. The entire apparatus is mounted on a sturdy, brightly colored stand. Scale: 1cm = 10cm]
Challenges and Risks
The following table details potential challenges and risks, along with mitigation strategies:
| Experiment | Challenge | Mitigation Strategy | Risk | Mitigation Strategy |
|---|---|---|---|---|
| Elasticity of Laughter | Subjectivity in laughter measurement | Develop a standardized laughter rating scale with clear criteria. | Participant discomfort/emotional distress | Ensure informed consent and provide breaks as needed. |
| Contagiousness of Giggles | Difficulty controlling extraneous variables | Employ a highly controlled environment and standardized procedures. | Ethical considerations related to inducing laughter | Obtain explicit consent for giggle induction and ensure participants can withdraw at any time. |
| Physics of a Honk | Difficulty in producing consistent honks | Train the clown to produce standardized honks using a specific technique and biofeedback. | Potential for hearing damage | Use hearing protection for participants and observers. |
A Clown’s Tale: The Elasticity of Laughter
The Laugh-o-Meter 5000 hummed quietly, a symphony of wires and sensors awaiting its first burst of laughter. My oversized red shoes tapped impatiently on the polished floor. This wasn’t just about measuring chuckles; this was about quantifying the very fabric of mirth, about proving that joy, too, obeyed the laws of physics. My hypothesis: the elasticity of laughter is directly proportional to the absurdity of the stimulus.
I’d spent weeks crafting a meticulously designed comedic sequence, escalating from gentle puns to full-blown slapstick routines.The first participant, a kindly old lady with a twinkle in her eye, responded with a series of delicate chuckles. The Laugh-o-Meter registered a low, but consistent, pressure and frequency. Then came the slapstick. A rogue banana peel, a perfectly timed pie in the face – the pressure readings spiked, the frequency soared.
The data confirmed my hypothesis – the more absurd the stimulus, the greater the elasticity of laughter. But then, something unexpected happened. During a particularly ridiculous juggling act involving rubber chickens, the Laugh-o-Meter overloaded. The data was messy, chaotic, a beautiful explosion of joy that defied quantification. It was a reminder that while science seeks to measure and understand, some phenomena, like the unbridled exuberance of laughter, are simply beyond the grasp of any instrument, no matter how brilliantly designed.
The experiment, despite the technical glitch, was a resounding success. It proved that laughter, in its purest form, is a force of nature.
The Clown’s Collaboration
Professor P. J. “Pipsqueak” Quibble, the genius clown physicist, possessed a unique approach to scientific collaboration, one that often challenged the conventions of the academic world. His unconventional methods, while initially met with skepticism, frequently yielded surprising and productive results. His collaborations were as vibrant and unpredictable as his stage performances.The clown’s interactions with other scientists were characterized by a playful yet rigorous exchange of ideas.
He eschewed formal presentations, preferring instead impromptu discussions punctuated by juggling, mime, and the occasional well-timed whoopie cushion. His ability to explain complex concepts through analogy and visual metaphors made him surprisingly effective at bridging the communication gap between specialists and non-specialists alike. This often led to unexpected insights from researchers outside his immediate field.
A Hypothetical Collaboration: Pipsqueak and the Traditional Physicist
Imagine a scenario where Professor Quibble collaborates with Dr. Amelia Stern, a highly respected but traditionally minded astrophysicist. Dr. Stern, initially hesitant about working with a clown, was intrigued by Pipsqueak’s unconventional approach to solving the long-standing mystery of dark energy. The challenges arose primarily from their vastly different communication styles.
Dr. Stern favored precise, data-driven discussions, while Pipsqueak preferred a more intuitive, playful approach, using visual aids like oversized rubber chickens and meticulously drawn diagrams on chalkboards. However, these differences ultimately proved beneficial. Pipsqueak’s out-of-the-box thinking, combined with Dr. Stern’s rigorous analytical skills, led to a breakthrough.
By translating Pipsqueak’s intuitive leaps into testable hypotheses, Dr. Stern was able to design experiments that provided compelling evidence supporting Pipsqueak’s unconventional theory. The success of their collaboration demonstrated the potential of embracing diverse perspectives in scientific research. Their joint paper, published in
Nature*, was met with a mixture of awe and amusement, significantly impacting the field of cosmology.
The Impact of the Clown’s Work on the Scientific Community
Professor Quibble’s unconventional approach had a profound, if somewhat unexpected, impact on the scientific community. His success in communicating complex scientific concepts to a wider audience through his engaging persona inspired a new wave of science communicators. His work also demonstrated the importance of embracing creativity and unconventional thinking in scientific research. While some remained skeptical of his methods, many scientists were forced to reconsider their own approaches, leading to a more inclusive and diverse scientific landscape.
The legacy of Professor Quibble extended beyond his specific discoveries, influencing how scientists communicate their work and approach collaborative research. His example encouraged a shift towards greater openness to unconventional ideas, demonstrating that groundbreaking discoveries can sometimes emerge from the most unexpected sources.
The Clown’s Legacy
Professor P. J. “Professor Pipsqueak” Quibble, the renowned theoretical physicist and surprisingly insightful clown, left an indelible mark on the scientific community and popular culture alike. His eccentric personality, coupled with his groundbreaking contributions to our understanding of relativity, cemented his status as a unique and influential figure. His legacy extends beyond his published works, encompassing his innovative teaching methods, his collaborative spirit, and the enduring inspiration he provided to aspiring scientists worldwide.Professor Quibble’s unique approach to science, characterized by his playful use of analogies and visual aids, made complex concepts accessible to a broader audience.
His ability to bridge the gap between the highly technical and the generally understandable was a key element of his success, leading to increased public interest in theoretical physics. This increased accessibility, in turn, fostered a new generation of scientists eager to explore the complexities of the universe.
A Short Biography of Professor P. J. Quibble
Born Percival John Quibble in 1962, he displayed an early aptitude for both mathematics and clowning. This unusual combination manifested in his unique scientific approach. His doctoral thesis, “A Jester’s Guide to General Relativity,” revolutionized the way certain aspects of spacetime were visualized and understood. He received numerous awards for his work, including the prestigious “Einstein’s Jester” medal.
His contributions to the field of theoretical physics include a novel interpretation of gravitational waves and a simplified model of quantum entanglement. He passed away in 2023, leaving behind a rich legacy of scientific discovery and whimsical inspiration.
A Museum Exhibit: “The World According to Pipsqueak”
The exhibit would begin with a large, whimsical entrance featuring a giant bouncy ball representing the curvature of spacetime. Inside, visitors would encounter interactive displays showcasing Professor Quibble’s life and work. A timeline would chart his academic career, punctuated by playful illustrations of his experiments. Artifacts on display would include his oversized spectacles, a collection of brightly colored chalkboards covered in complex equations and whimsical diagrams, and a replica of his custom-built “gravity-defying” unicycle, used in several of his demonstrations.
A dedicated section would feature videos of his lectures, showcasing his engaging teaching style. A central display would feature a holographic projection of Professor Quibble explaining his theories in his characteristically entertaining manner. Finally, a large interactive screen would allow visitors to manipulate virtual models of his experiments and explore the implications of his theories.
The Lasting Impact of Professor Quibble’s Theories
Professor Quibble’s simplified models of complex physical phenomena have already influenced the design of advanced gravitational wave detectors. His work on quantum entanglement has sparked new research into quantum computing. His unique approach to teaching has inspired educators to create more engaging and accessible science curricula. His legacy extends to the countless students and scientists he inspired, fostering a new generation of physicists who are not only brilliant but also creatively engaged with their work.
His contributions will continue to shape the future of physics for decades to come.
The Clown’s Critics
The genius clown’s unorthodox approach to physics, combining whimsical performance with rigorous scientific inquiry, inevitably attracted criticism. While his breakthroughs were undeniable, certain aspects of his methodology and conclusions faced scrutiny from the established scientific community. These critiques, however, often failed to account for the unique context of his work and the innovative nature of his discoveries.
Criticisms of the Clown’s Methodology
Several critics questioned the clown’s reliance on visual aids and theatrical demonstrations in his scientific presentations. They argued that such methods lacked the rigor and precision expected in formal scientific publications, potentially leading to misinterpretations and a lack of reproducibility. Furthermore, some felt that the clown’s playful, often chaotic, experimental setups lacked the controlled environment necessary for valid scientific inquiry.
The incorporation of juggling, unicycling, and other circus acts into his experiments was viewed as distracting and irrelevant to the scientific process. The critics suggested that his work should adhere more strictly to established norms of scientific presentation and methodology.
Counterarguments to Methodological Criticisms
The clown, however, defended his methods by arguing that his unconventional approach made complex concepts accessible to a wider audience. He contended that the visual nature of his demonstrations enhanced understanding, making abstract ideas more concrete and intuitive. He also highlighted that the seemingly chaotic elements of his experiments often served a purpose, revealing unexpected insights and stimulating creative problem-solving.
For example, the trajectory of a bouncing ball during a juggling routine might unexpectedly illuminate a point about gravitational forces. He believed that rigid adherence to traditional methods could stifle innovation and creativity, arguing that his results, despite the unconventional methods, spoke for themselves. He further pointed out that reproducibility was not necessarily compromised; detailed accounts of his experiments, albeit unconventional in their presentation, were meticulously documented.
Criticisms of the Clown’s Conclusions
Some critics expressed concern about the clown’s tendency to draw sweeping conclusions from limited data sets. They argued that his interpretations were sometimes speculative and lacked the robust statistical analysis necessary to support his claims. Furthermore, certain critics pointed to a perceived lack of peer review in the validation of his findings, asserting that the novelty of his approach had prevented sufficient scrutiny from the wider scientific community.
The unique nature of his communication style, while engaging, may have also hampered thorough and objective evaluation by his peers.
Counterarguments to Conclusion-Based Criticisms
The clown countered these criticisms by highlighting the groundbreaking nature of his discoveries. He argued that while the data sets might have been smaller than those used in traditional experiments, the significance of his findings justified the less conventional approach. He explained that his initial focus was on generating new hypotheses and exploring unconventional avenues of inquiry, rather than immediately pursuing exhaustive verification.
He further suggested that the delay in formal peer review was due to the novelty of his work, which required a period of assimilation and understanding within the scientific community. He acknowledged the need for rigorous verification but argued that his preliminary findings deserved serious consideration and further investigation.
The Clown’s Public Response to Criticism
In a widely publicized lecture, the clown addressed his critics directly, incorporating his characteristic humor and theatrical flair. He began by acknowledging the validity of some concerns regarding methodology, stating,
“It’s true, my experiments might look a bit…unconventional. But remember, Einstein didn’t discover relativity by sticking strictly to the rule book!”
He then proceeded to demonstrate, using a series of juggling balls and a unicycle, how his unconventional methods could lead to profound insights. He concluded by inviting his critics to collaborate and engage in further research, emphasizing the importance of open dialogue and intellectual exchange in the pursuit of scientific truth. He framed the criticisms not as attacks, but as valuable opportunities for refinement and improvement, further highlighting the importance of scientific debate and collaborative exploration.
The Clown’s Inspiration
Professor P.J. “Patches” Pipsqueak, the renowned relativistic clown, drew inspiration from a surprisingly diverse range of sources, weaving together personal experiences, scientific theories, and artistic influences to forge his unique understanding of spacetime. His approach was not merely a synthesis, but a vibrant, chaotic ballet of these influences, each contributing to a singular, almost absurdly elegant, interpretation of relativity.
Source Identification & Analysis
The following table details the key sources that fueled Patches’s groundbreaking work. Each entry highlights the specific connection to his relativistic theories and provides concrete examples from his research.
| Source of Inspiration | Description (Specific examples required) | Connection to Relativity (Detailed explanation) | Evidence (Cite specific aspects of clown’s work) |
|---|---|---|---|
| Childhood Trauma (a near-miss circus accident involving a collapsing trapeze) | A near-fatal fall during a childhood circus performance left Patches with a profound sense of the fragility and unpredictability of existence, profoundly impacting his worldview. | This experience instilled a deep appreciation for the inherent uncertainty and probabilistic nature of reality, influencing his development of a probabilistic interpretation of quantum gravity, interwoven with his relativistic framework. | Patches’s “Stochastic Spacetime” model, detailed in his seminal paper “Gravity’s Giggles: A Probabilistic Approach,” explicitly references the “chaotic dance of particles” as mirroring his near-death experience. |
| Einstein’s General Relativity | Einstein’s theory of General Relativity served as the foundational framework for Patches’s work, providing the mathematical and conceptual tools for his investigations. | Patches built upon Einstein’s work, extending it into realms previously unexplored, notably by incorporating probabilistic elements and exploring the implications of spacetime curvature on quantum phenomena. | Patches frequently cited Einstein’s field equations in his lectures, but always with a playful, almost irreverent, twist, suggesting modifications and extensions. His “Curvature Cartoons” series visually represented these modifications. |
| Surrealist Art (particularly the works of Salvador Dalí) | Dalí’s ability to portray distorted realities and dreamlike landscapes profoundly influenced Patches’s visual representation of spacetime. | Dalí’s manipulation of perspective and the unexpected juxtaposition of elements resonated with Patches’s own non-conventional approach to relativity, leading to innovative visualizations of complex concepts. | Patches’s “Melted Clocks of Spacetime” series, a collection of distorted clocks illustrating time dilation, directly mirrors Dalí’s famous melting clocks. |
Relativistic Approach Shaping
Patches’s near-death experience shaped his probabilistic interpretation of relativity, introducing an element of inherent uncertainty not present in conventional approaches. Einstein’s General Relativity provided the mathematical foundation, but Patches extended it by incorporating quantum mechanics and his unique understanding of chance. Finally, Dalí’s surrealist art provided a powerful visual language to represent these complex ideas, making them accessible to a wider audience.
The childhood trauma had the most significant influence, as it fundamentally altered his perception of reality, influencing his probabilistic interpretations of spacetime. His approach differs from conventional interpretations through the incorporation of probabilistic elements and his unconventional, often humorous, visual representations.
Background & Personality Influence
Patches’s upbringing in a traveling circus instilled in him a nomadic spirit and a deep appreciation for the unexpected. His education was unconventional, largely self-taught, punctuated by practical experience in the circus. This background fostered an intuitive understanding of physics, devoid of rigid academic constraints. Patches possessed a playful, extroverted personality, utilizing humor and visual aids to communicate complex scientific concepts.
His meticulous attention to detail, however, contrasted with his outwardly chaotic demeanor. His writings and performances are filled with witty asides and visual puns, reflecting his personality and approach.
Creative Writing
The scent of sawdust and ozone hung heavy in the air. My oversized spectacles fogged as I stared at the equations scrawled across the canvas tent. The trapeze accident – the near-miss that had warped my sense of time, of gravity itself – played in my mind’s eye. That terrifying, weightless moment, the dizzying rush of falling, then the sudden, jarring stop.
It was a glimpse into the chaotic dance of spacetime, a dance Einstein only hinted at. Tonight, I felt, I finally grasped it. My probabilistic model, inspired by that fall, was coming together. The equations were a kaleidoscope of swirling colors, a distorted reflection of Dalí’s melting clocks, reflecting the very fabric of spacetime itself. I wasn’t just describing the curvature of spacetime; I was describing its inherent unpredictability, its joyous, almost whimsical, randomness.
The laughter of the audience drifted in from the outside, a reminder that even amidst the most profound mysteries, joy, absurdity, and a good clown could still find their place. This was not a sterile equation; it was a testament to the unpredictable beauty of existence. The final formula, a chaotic masterpiece, shimmered before me, a cosmic joke on the very nature of reality.
It felt like a culmination of a lifetime spent chasing the elusive truth, a truth revealed not in a cold laboratory, but under the big top, amidst the scent of popcorn and the roar of the crowd.
The Clown and Popular Culture
The genius clown’s unique blend of scientific brilliance and comedic performance presents a rich tapestry for popular culture adaptation, offering opportunities for engaging narratives and thought-provoking explorations of science and society. The inherent absurdity of a clown grappling with complex physics provides a fertile ground for diverse interpretations across various media.
Narrative Adaptation
The clown’s life story, brimming with eccentric experiments, scientific breakthroughs, and interpersonal conflicts, lends itself naturally to compelling narratives in film and literature. The juxtaposition of highbrow scientific concepts with lowbrow comedic antics creates a dramatic tension that can be exploited for engaging storytelling.
Movie Script: A Three-Act Structure
- Act I: The Prodigy: Introduces the clown as a child prodigy, showcasing their early fascination with science and their unique comedic talent. The act culminates with a pivotal event that forces the clown to choose between a conventional scientific career and pursuing their passion as a comedic scientist.
- Act II: The Breakthrough: The clown, now a renowned (if eccentric) performer, dedicates themselves to their scientific research, facing obstacles and setbacks along the way. A rival scientist emerges, creating conflict and competition, and the clown experiences a personal crisis that threatens their work.
- Act III: The Revelation: The clown makes their groundbreaking scientific discovery, but their unconventional methods and comedic persona cause skepticism and controversy within the scientific community. The climax involves a public demonstration where the clown must prove their theory, winning over both the scientific community and the public.
Character arcs would see the clown evolve from a naive genius to a confident yet still quirky scientist, while the rival scientist might undergo a transformation from jealous competitor to begrudging admirer.
Novel Chapter Artikel
- Chapter 1: The Circus Birth: Focuses on the clown’s childhood, highlighting their early fascination with science amidst the chaotic circus environment. This chapter utilizes flashbacks to illustrate the origins of their unique perspective.
- Chapter 2: The University Years: Depicts the clown’s struggles to fit in at a prestigious university, navigating academic pressures and social isolation. This chapter is told from the perspective of a supportive friend.
- Chapter 3: The Eureka Moment: Describes the clown’s groundbreaking discovery, showcasing their unconventional approach to scientific research. This chapter utilizes stream-of-consciousness writing to convey the chaotic and exhilarating nature of the moment.
- Chapter 4: The Media Frenzy: Explores the public reaction to the clown’s discovery, highlighting the media’s fascination with their unique personality and the challenges of communicating complex scientific concepts to a wider audience.
- Chapter 5: The Legacy: Examines the long-term impact of the clown’s work, exploring both the scientific advancements and the cultural influence of their unique approach to science communication.
The novel would be written from multiple perspectives, offering diverse viewpoints on the clown’s life and work.
Genre and Tone
The movie would be a comedic drama, blending humor with elements of scientific intrigue and personal drama. The tone would be whimsical, yet grounded in the reality of scientific endeavor and the complexities of human relationships. The novel would follow a similar genre and tone, leaning more towards a biographical fiction approach. This choice reflects the inherent duality of the clown’s persona: a brilliant scientist who expresses themselves through comedy.
Movie Poster Design
The movie poster would feature a vibrant, slightly chaotic aesthetic.
Visual Elements
The central image would depict the clown in their signature attire, perhaps mid-experiment, surrounded by scientific apparatus and circus props. The color palette would be bold and contrasting, using bright primary colors alongside muted tones to represent the blend of comedy and serious science. The overall aesthetic would be playful but sophisticated, suggesting both intellectual depth and comedic flair.
Tagline Creation
- Suspenseful: “He’s got the universe figured out. But can he handle the circus?”
- Humorous: “Relativity? It’s no laughing matter… unless you’re a genius clown.”
- Thought-Provoking: “Where does science end and laughter begin?”
Plot Summary for Poster
A genius clown revolutionizes physics with their unorthodox methods, facing skepticism and ridicule as they attempt to prove their groundbreaking theory.
Target Audience
The target audience would be adults and young adults interested in science, comedy, and unique storytelling. The poster design caters to this audience by combining playful imagery with a sophisticated color palette and thought-provoking taglines.
Impact on Public Perception of Science
The clown’s story could significantly impact public perception of science by humanizing scientific endeavor and making it more accessible and engaging.
Specific Scientific Concepts
- Theories of Laughter: The clown’s comedic persona allows for exploration of the psychology of humor and its role in communication and learning.
- The Psychology of Fear: The clown’s unconventional approach to science and their willingness to challenge established norms could spark discussions about overcoming fear of the unknown and embracing unconventional ideas.
- The History of Circus Performance: The circus setting provides a rich backdrop for exploring the history of entertainment and the evolution of scientific understanding.
Public Perception Analysis
| Scientific Concept | Potential Reinforcement of Public Perception | Potential Challenge to Public Perception | Evidence/Examples from the Clown’s Story ||—|—|—|—|| Theories of Laughter | The clown’s success in communicating complex ideas through humor could reinforce the idea that laughter is a powerful tool for learning and understanding. | The clown’s use of humor might challenge the perception that science is a purely serious and humorless endeavor.
| The clown’s ability to explain relativity using comedic skits and props. || The Psychology of Fear | The clown’s overcoming of obstacles and skepticism could reinforce the idea that perseverance and a willingness to take risks are essential for scientific progress. | The clown’s eccentric persona might challenge the perception that scientists must be serious and conformist. | The clown’s persistence in the face of ridicule and opposition from other scientists.
|| The History of Circus Performance | The clown’s use of circus imagery and performance techniques could reinforce the idea that science and entertainment are not mutually exclusive. | The clown’s unique blend of science and comedy could challenge the perception that science is a purely academic and isolated pursuit. | The clown’s incorporation of circus elements into their scientific demonstrations.
|
Long-Term Impact
The clown’s story has the potential to foster a greater appreciation for the human side of science and encourage broader public engagement with scientific concepts. However, there’s a risk that the clown’s unconventional approach might be misinterpreted as trivializing science, undermining its rigor and importance.
Additional Considerations
Ethical considerations arise regarding the portrayal of the clown’s potentially eccentric or even disruptive behavior. It’s crucial to balance the comedic elements with a responsible and accurate depiction of the scientific process, avoiding the risk of glorifying potentially harmful or irresponsible actions. Careful consideration of the portrayal of the clown’s personality and their interactions with others is necessary to ensure a sensitive and nuanced representation.
The Clown’s Mathematical Approach to Relativity

Professor P.J. Chuckles, the renowned relativistic clown, revolutionized the field not only with his insightful theories but also with his uniquely whimsical mathematical approach. His methods, while unconventional, often provided fresh perspectives on complex problems, challenging established norms and sparking debate within the scientific community. This section delves into the specifics of his mathematical notations, problem-solving techniques, and a comparison with standard relativistic methods.
Mathematical Notations and Approaches
Professor Chuckles’ mathematical notations were as colorful and unpredictable as his performances. He believed in making complex concepts accessible, even playful, through his unique symbolic representations.
- Time Dilation Notation: Chuckles represented time dilation using a pair of interconnected juggling balls. The size of each ball represented the time interval in different reference frames. A larger ball indicated a longer time interval, and the relative size difference between the balls represented the time dilation factor. The connection between the balls symbolized the relativistic relationship between the frames.
For example, if one ball was twice the size of the other, it would visually represent a time dilation factor of 2.
- Length Contraction Notation: Length contraction was depicted using a series of brightly colored rubber bands stretched across a miniature stage. The initial length of the rubber band represented the proper length of an object. As the bands were stretched (representing increased relative velocity), the resulting shorter length demonstrated the relativistic length contraction. The amount of stretch directly corresponded to the Lorentz factor.
- Relativistic Mass Notation: Relativistic mass was visualized using stacks of differently sized clown hats. The height of each stack represented the mass in a particular reference frame. As the velocity increased, additional hats were added to the stack, illustrating the increase in relativistic mass. The rate of hat addition directly corresponded to the relativistic mass increase formula.
Solving a Problem in General Relativity
Let’s examine Chuckles’ approach to calculating gravitational time dilation near a black hole. Consider a clock at a distance r from a black hole of mass M. Chuckles would first represent the black hole using a large, weighted rubber ball (representing the immense gravitational field). He would then use a smaller ball (representing the clock) and place it at a specific distance from the large ball.
The time dilation would be visually represented by the speed at which the smaller ball orbits the larger one, with slower orbits indicating greater time dilation. Mathematically, he might use a modified version of the Schwarzschild metric, represented using a series of interconnected rubber bands with varying tensions, reflecting the curvature of spacetime. The rate of change in the tension of the rubber bands, as the smaller ball approached the larger ball, would directly represent the time dilation effect.
Unconventional Mathematical Tools
Professor Chuckles’ methods were far from conventional.
- Juggling Variables: He often used juggling balls, each representing a variable in an equation. The pattern of the juggling act itself represented the mathematical operations, with throws and catches symbolizing additions and subtractions, while the height of the throws corresponded to the magnitude of the variables.
- Unicycle Curves: The path traced by a unicycle rider, representing a curve in spacetime, was used to visualize and analyze the curvature of spacetime. The speed and direction of the unicycle rider would directly translate into the mathematical parameters of the curve. For instance, a sharp turn would represent a high degree of curvature.
Comparison with Standard Techniques
The following table compares Chuckles’ method for calculating time dilation with the standard Lorentz transformation:
| Feature | Clown’s Method | Standard Lorentz Transformation |
|---|---|---|
| Notation | Interconnected juggling balls; ball size represents time interval. | t’ = γ(t – vx/c²) |
| Calculation Steps | Visual comparison of ball sizes to determine the dilation factor. | Direct calculation using the Lorentz factor (γ) and relative velocity (v). |
| Result | Visual representation of time dilation factor. | Numerical value of the time dilation factor. |
| Advantages | Intuitive visualization; easily grasped by non-experts. | Precise numerical results; widely accepted and applicable. |
| Disadvantages | Lack of numerical precision; difficult for complex calculations. | Can be abstract and difficult to visualize for non-experts. |
Clown’s Approach to Spacetime Curvature
Chuckles’ approach to spacetime curvature, using his unicycle analogy, deviated significantly from the standard Einstein field equations. While Einstein’s equations utilize complex tensor calculus, Chuckles used the unicycle’s path to visually represent the geodesic. This approach, while offering intuitive visualization, lacked the mathematical rigor of the Einstein field equations, limiting its application to simple scenarios. The lack of precise mathematical formulation made it difficult to make quantitative predictions.
Advantages and Disadvantages of the Clown’s Mathematical Style
- Advantages:
- Intuitive Understanding: The use of physical objects made complex concepts easier to grasp, especially for those without a strong mathematical background.
- Ease of Visualization: The visual nature of his methods aided in understanding abstract relativistic concepts.
- Potential for Novel Insights: His unconventional approach sometimes led to new perspectives and interpretations of relativistic phenomena.
- Disadvantages:
- Lack of Rigor: The lack of formal mathematical expressions made his methods difficult to verify and replicate.
- Difficulty in Peer Review: The unconventional nature of his approach made it challenging for other scientists to evaluate his work using standard academic criteria.
- Limitations in Applicability: His methods were primarily useful for simple illustrations and lacked the precision needed for complex calculations.
The clown’s mathematical approach, while lacking the rigor of established methods, offers a valuable contribution by making relativity more accessible and intuitive. Its primary strength lies in its ability to visualize complex concepts, but its limitations in precision and formal mathematical expression restrict its applicability to complex problems. The potential for future applications remains limited, but its pedagogical value is undeniable.
Creative Writing
The spotlight illuminated Professor P.J. Chuckles as he balanced precariously on his unicycle, a chalkboard propped before him. He was attempting to calculate the time dilation experienced by a cosmic clown traveling near a black hole. He tossed three juggling balls into the air – one representing the proper time, another the relative velocity, and the third, the gravitational field’s strength.
The trajectory of the balls, a chaotic ballet of throws and catches, mirrored his unique calculations. He adjusted the speed of his unicycle, representing the warping of spacetime, muttering, “A little more curvature here… yes, that’s it!” The audience, a mix of bewildered physicists and delighted children, watched, mesmerized. While his method was unorthodox, his final answer, scribbled on the board in crayon, matched the predictions of the standard equations, albeit through a far more entertaining process.
The challenge remained in translating his performance into a universally understood, rigorously documented scientific paper. But for now, the laughter of understanding echoed louder than any criticism.
The Clown’s Use of Analogy
Professor P.J. “Professor Pipsqueak” Quibble, the genius clown of relativity, understood that complex scientific concepts, particularly those within Einstein’s theories, required creative simplification for broader comprehension. His mastery lay not only in his scientific acumen but also in his skillful deployment of analogies, transforming abstract ideas into relatable, memorable images. He consistently used familiar scenarios to illuminate the often counterintuitive nature of spacetime.Professor Pipsqueak’s analogies were effective because they bridged the gap between the highly specialized language of physics and the everyday experiences of his audience.
The hypothetical scenario of a genius clown grappling with the intricacies of the theory of relativity highlights the immense cognitive capacity required to master such complex physics. This necessitates a robust and comprehensive approach to acquiring and processing information, much like utilizing a structured absorb knowledge base to efficiently manage and synthesize vast quantities of data. Such a system would prove invaluable to our theoretical clown, enabling a more effective exploration of spacetime and gravitational fields.
By anchoring abstract concepts in concrete examples, he facilitated understanding and fostered a deeper appreciation for the underlying principles. This approach, coupled with his flamboyant presentation style, made even the most intricate aspects of relativity accessible and engaging.
Examples of Professor Pipsqueak’s Analogies
Professor Pipsqueak employed several memorable analogies to explain key concepts in relativity. These were not mere illustrative tools; they were integral parts of his teaching methodology, designed to create intuitive understanding rather than rote memorization.
- Spacetime as a Trampoline: To illustrate the curvature of spacetime caused by mass, Professor Pipsqueak often used the analogy of a trampoline. He’d place a bowling ball in the center of a stretched trampoline, demonstrating how the ball creates a dip, representing the warping of spacetime. Then, he’d roll marbles around the bowling ball, showing how their paths are bent by the curvature, mirroring how objects move along curved paths in a gravitational field.
The visual impact of this demonstration was unparalleled, instantly conveying the essence of general relativity.
- Time Dilation as a Moving Train: To explain time dilation, Professor Pipsqueak would imagine a train traveling at near light speed. He’d explain that a clock on the train would appear to tick slower to an observer standing still on the platform than a clock standing still on the platform would appear to tick to an observer on the train. This analogy effectively demonstrated the relative nature of time, showing how the speed of an object affects its perception of time.
He would often add humorous flourishes, like having a clown on the train juggling increasingly slower-moving balls to emphasize the time dilation.
- Length Contraction as a Shrinking Ruler: Professor Pipsqueak cleverly used a ruler to demonstrate length contraction. He’d explain that a ruler moving at near light speed would appear shorter to a stationary observer than it would appear to an observer moving with the ruler. He’d dramatically “shrink” a large ruler with a magic trick (which he’d then explain was an analogy, not actual magic!), driving home the point that the length of an object is relative to the observer’s frame of reference.
Effectiveness of Professor Pipsqueak’s Analogies
The effectiveness of Professor Pipsqueak’s analogies stemmed from their simplicity, relatability, and visual nature. By using everyday objects and scenarios, he made complex scientific concepts accessible to a wide audience, including those without a strong background in physics. The visual aspect of his demonstrations, often involving props and theatrical flair, further enhanced the impact of his analogies, making them more memorable and easier to grasp.
Limitations of Analogies in Explaining Scientific Concepts
While analogies are powerful tools for communication, they have limitations. Professor Pipsqueak was acutely aware of these limitations and always emphasized that his analogies were simplified representations, not perfect models. Analogies, by their nature, are imperfect; they can oversimplify complex phenomena, potentially leading to misconceptions if not carefully explained and contextualized. Furthermore, analogies can break down when pushed beyond their intended scope.
For instance, the trampoline analogy for spacetime curvature doesn’t fully capture the four-dimensional nature of spacetime. Professor Pipsqueak always carefully addressed these limitations in his lectures and demonstrations, ensuring that his audience understood the boundaries of the analogies he employed.
The Clown’s Philosophical Implications
The clown’s unorthodox approach to theoretical physics, while entertaining, yielded surprisingly profound philosophical implications regarding our understanding of space, time, and the universe. His relativistic model, expressed through a unique blend of mathematical rigor and whimsical analogy, challenged established interpretations and spurred new avenues of philosophical inquiry. This section will explore the deeper meaning embedded within his scientific contributions.The clown’s relativistic framework, unlike many purely mathematical models, directly engaged with the nature of reality itself.
His work subtly suggested a universe less deterministic than previously conceived, hinting at the inherent limitations of observation and the subjective experience of time and space. This contrasted with the more deterministic leanings of some earlier physicists, who sought absolute, universally applicable laws governing the cosmos. For example, while Newton’s laws implied a clockwork universe operating under predictable rules, the clown’s work, similar to Einstein’s, introduced an element of uncertainty and observer dependence, making the concept of objective reality more nuanced.
Space-Time as a Dynamic Entity
The clown’s visualization of space-time as a malleable, almost playful entity, directly challenged the classical notion of a static, absolute background against which events unfold. He often depicted space-time as a circus tent, constantly shifting and reshaping under the influence of gravity and energy, a visual metaphor contrasting with the rigid, Euclidean space assumed in classical physics. This dynamic conception aligns with Einstein’s general relativity, but the clown’s whimsical presentation made the concept accessible to a wider audience, sparking broader philosophical discussions about the fluid nature of reality.
The Subjectivity of Time
The clown’s experiments frequently demonstrated the relative nature of time, showcasing how the passage of time could vary depending on an observer’s velocity and gravitational environment. He might illustrate this with a juggling act, where the perceived speed of the juggling balls would change based on the observer’s relative motion. This highlights the subjective experience of time, a concept explored by philosophers for centuries, but rarely so vividly presented within a scientific context.
This resonates with philosophical discussions on the nature of consciousness and its role in shaping our perception of reality.
Implications for Determinism and Free Will
The inherent uncertainties introduced by the clown’s relativistic model have philosophical implications for the age-old debate on determinism versus free will. The suggestion that the universe is not entirely predictable, even at a fundamental level, opens the door for a more nuanced understanding of agency and choice. While the clown’s work didn’t explicitly address free will, the inherent probabilistic nature of his model could be interpreted as supporting a less deterministic view of the universe, where chance and unforeseen events play a significant role.
This resonates with philosophical perspectives that emphasize the role of contingency and emergent properties in shaping reality.
The Clown’s Epistemological Stance
The clown’s reliance on analogies and visual representations suggests a pragmatic epistemological stance, prioritizing understanding and accessibility over purely abstract mathematical formalism. This contrasts with some physicists who emphasize the elegance and internal consistency of mathematical frameworks above all else. The clown’s approach emphasizes the importance of making complex ideas understandable to a broader audience, thus highlighting the social and communicative aspects of scientific knowledge.
His methods suggest that effective communication is crucial for the advancement of scientific understanding, thereby placing a greater emphasis on the collaborative nature of knowledge production.
The Clown’s Teaching Methods
Professor P.J. (Professor Jester), the genius clown physicist, revolutionized the teaching of relativity through his uniquely engaging approach. He understood that complex concepts could be made accessible and even enjoyable through humor, visual aids, and interactive demonstrations, transforming the often-daunting subject into a captivating performance. His methods significantly improved student understanding and appreciation of Einstein’s theories.Professor P.J.’s lesson plans were meticulously designed to build upon prior knowledge, gradually introducing increasingly complex ideas.
He employed a layered approach, starting with simple analogies and progressing to more rigorous mathematical explanations, always ensuring a solid foundation at each stage. His lessons were not simply lectures; they were theatrical productions, complete with costumes, props, and carefully choreographed demonstrations.
Lesson Plan: Relativity for High School
This lesson plan focuses on special relativity, aiming to introduce the concepts of time dilation and length contraction in an engaging manner. Day 1: The Circus of Relativity
Introduction (15 min)
Professor P.J. enters as a flamboyant clown, juggling balls representing different frames of reference. He introduces the concept of relative motion through a playful scenario involving two clowns on moving platforms, demonstrating that their observations of each other’s speed differ.
Time Dilation (30 min)
Using a large clock and a miniature clock, Professor P.J. demonstrates time dilation through a thought experiment involving a clown traveling at near light speed. The visual difference in the clocks’ speeds highlights the concept. He uses exaggerated movements and humorous dialogue to emphasize the counterintuitive nature of the phenomenon.
Length Contraction (30 min)
Professor P.J. uses a long, flexible rubber band representing a spaceship to demonstrate length contraction. As the “spaceship” moves at increasingly high speeds (simulated), the rubber band appears to shrink, illustrating the concept visually. Day 2: The Mathematical Tightrope
Review and Reinforcement (15 min)
A brief review of Day 1’s concepts, using simple questions and humorous anecdotes to refresh students’ memories.
Lorentz Transformations (45 min)
Professor P.J. introduces the Lorentz transformations using a series of increasingly complex visual aids. He uses brightly colored diagrams and humorous annotations to make the equations less intimidating. He relates the equations to the previous day’s visual demonstrations, emphasizing the mathematical basis for the observed phenomena.
Problem Solving (30 min)
Students work in small groups on simple problems involving time dilation and length contraction, guided by Professor P.J. who provides encouragement and humorous feedback.
Humor and Visual Aids in Teaching Relativity
Professor P.J. expertly integrated humor into his lessons, using slapstick, puns, and witty remarks to engage students and make complex concepts memorable. His visual aids were not merely illustrations; they were integral parts of his performance. He used oversized clocks, flexible rubber bands, and even a miniature model of the universe to bring abstract ideas to life. For instance, to explain spacetime, he might use a trampoline with a bowling ball in the center, demonstrating how massive objects warp the fabric of spacetime.
Effectiveness of the Clown’s Teaching Methods
Studies comparing Professor P.J.’s teaching methods to traditional methods revealed a significant improvement in student comprehension and engagement. Students in his classes consistently outperformed those taught using traditional methods on tests of conceptual understanding. Furthermore, student feedback consistently highlighted the positive impact of his humorous and engaging approach, demonstrating a higher level of enjoyment and motivation in learning relativity.
His methods fostered a deeper appreciation for the subject, leading to increased interest in science and STEM fields.
The Clown’s Future Research
Professor P. J. Chuckles, the renowned clown-physicist, intends to expand his research into previously unexplored territories, leveraging his unique perspective and unconventional methods. His future work promises to bridge the gap between seemingly disparate fields, offering novel insights and potentially groundbreaking applications.
Research Areas
The following table Artikels Professor Chuckles’ planned research areas, their potential impacts, and key questions addressed. These areas represent a logical progression from his previous work on relativity, incorporating his characteristic blend of scientific rigor and comedic flair.
| Research Area | Brief Description | Potential Impact | Key Questions Addressed |
|---|---|---|---|
| The Physics of Clown-Based Propulsion Systems | Investigating the aerodynamic properties of oversized shoes and their application in novel propulsion systems. This includes analyzing the effects of varying shoe size, shape, and material on thrust and efficiency. | Development of more efficient and environmentally friendly transportation, potentially revolutionizing personal and public transit. | The optimal shoe size-to-thrust ratio has been determined through extensive wind tunnel testing. Methods for minimizing drag through innovative shoe design have been developed. |
| The Socioeconomic Impact of Clown Performance | Analyzing the correlation between clown performance and audience emotional response in different socioeconomic groups. This involves studying laughter’s physiological and psychological effects and how these vary across cultural contexts. | Identifying effective strategies for using clown performance to address social issues, promoting well-being, and fostering community engagement. | Cultural variations in humor have been documented and categorized. Adaptive clown performance techniques for diverse audiences have been developed and tested. |
| The Application of Clown Makeup in Camouflage and Deception | Exploring the use of clown makeup techniques to create effective camouflage and disguise in various environments. This research involves analyzing the visual properties of clown makeup and its interaction with different backgrounds and lighting conditions. | Potential applications in military, law enforcement, or even wildlife observation, offering novel approaches to concealment and disguise. | The limitations of clown-based camouflage in various environments have been identified. Techniques for improving its effectiveness, such as incorporating dynamic elements and adapting to specific contexts, have been developed. |
Potential Breakthroughs
Professor Chuckles anticipates several significant breakthroughs from his future research endeavors.>Research Area 1 Breakthrough: The development of a functional prototype of a “Bozo-Booster,” a clown-shoe-based propulsion system capable of achieving short-range, low-altitude flight. This would demonstrate the feasibility of using unconventional designs for efficient and sustainable transportation.>Research Area 2 Breakthrough: The creation of a standardized “Laughter Index,” a quantifiable metric for measuring the emotional impact of clown performances across different demographics.
This would allow for the tailoring of clown performances to maximize positive social and psychological effects.>Research Area 3 Breakthrough: The development of a “Chaotic Camouflage” system based on principles of unpredictable visual patterns inspired by clown makeup. This system could potentially render objects virtually invisible in complex, dynamic environments.
Resources and Collaborations
The successful execution of Professor Chuckles’ research requires significant resources and collaborations.Research Area 1: Required Resources and Collaborations:
- Funding from a private foundation specializing in unconventional propulsion technologies.
- Access to a state-of-the-art wind tunnel and advanced simulation software.
- Collaboration with aerospace engineers from MIT and Caltech.
Research Area 2: Required Resources and Collaborations:
- Funding from a research grant focusing on the social impact of arts and culture.
- Access to diverse population samples for behavioral studies.
- Collaboration with psychologists, sociologists, and anthropologists from leading universities.
Research Area 3: Required Resources and Collaborations:
- Funding from a government agency interested in advanced camouflage technologies.
- Access to specialized imaging and optical equipment.
- Collaboration with military scientists and experts in visual perception.
Ethical Considerations
The ethical implications of Professor Chuckles’ research must be carefully considered. The potential misuse of the “Chaotic Camouflage” system in criminal activities necessitates the development of stringent guidelines for its application. Similarly, ensuring responsible and ethical application of findings from the socioeconomic impact of clown performance is crucial to prevent misrepresentation or manipulation.
Expected Timeline
Professor Chuckles projects the following timeline for his research:Research Area 1: Prototype completion within 3 years, followed by field testing over the next 2 years.Research Area 2: Data collection and analysis within 2 years, followed by report publication and dissemination within 1 year.Research Area 3: Initial prototype development within 2 years, followed by advanced testing and refinement over the next 3 years.
Dissemination Plan
Findings from each research area will be disseminated through peer-reviewed publications, presentations at international conferences, and public lectures. Professor Chuckles will also utilize his unique communication style to engage a broader audience, including interactive demonstrations and collaborations with educational institutions.
Question & Answer Hub
What inspired the clown to study relativity?
A combination of factors, including a childhood fascination with the cosmos, a personal tragedy that spurred existential reflection, and the influence of a particularly insightful physics professor.
How does the clown’s clowning influence their scientific work?
The clown’s ability to think outside the box, to find humor in the unexpected, and to connect with diverse audiences informs their unique problem-solving strategies and communication style.
What are the ethical implications of the clown’s discoveries?
Some discoveries raise questions about the responsible use of advanced technologies and the potential consequences of manipulating spacetime.
Does the clown have any rivals or detractors in the scientific community?
Yes, some traditional physicists initially resist the clown’s unconventional methods, but their groundbreaking results gradually win over many skeptics.
What is the clown’s ultimate goal?
To use their unique blend of science and performance to inspire others to embrace creativity, critical thinking, and the pursuit of knowledge.


